Can the Tangents help?

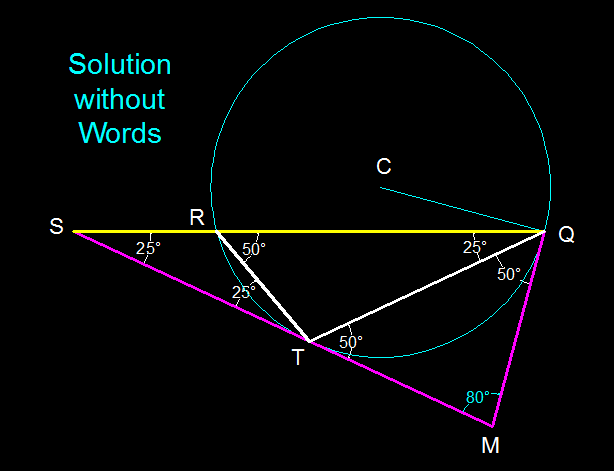
Draw $RT$ . Since $SR=TR$ , $\triangle TRS$ is isosceles. So $\angle RTS=\angle RST=25^\circ$ . By the exterior angle theorem, $\angle QRT = 25+25=50^\circ$ .

Draw $QC$ and $CR$ and $CT$ . $\angle CTR=90-25=65^\circ$

Since $\triangle CTR$ is isosceles, $\angle CRT=\angle CTR=65^\circ$ .Therefore, $\angle CRQ=65-50=15^\circ$ .

Since $\triangle QCR$ is isosceles, $\angle CQR=\angle CRQ=15^\circ$ . Therefore, $\angle MQS=90-15=75^\circ$ .

Let $K$ be the intersection of $QS$ and $QT$ . $\angle QKT=\dfrac{360-2(65)}{2}=115^\circ$

The sum of the interior angle of a quadrilateral is $360^\circ$ . We have

$\angle QMT=360-75-115-90=$ $\boxed{80^\circ}$