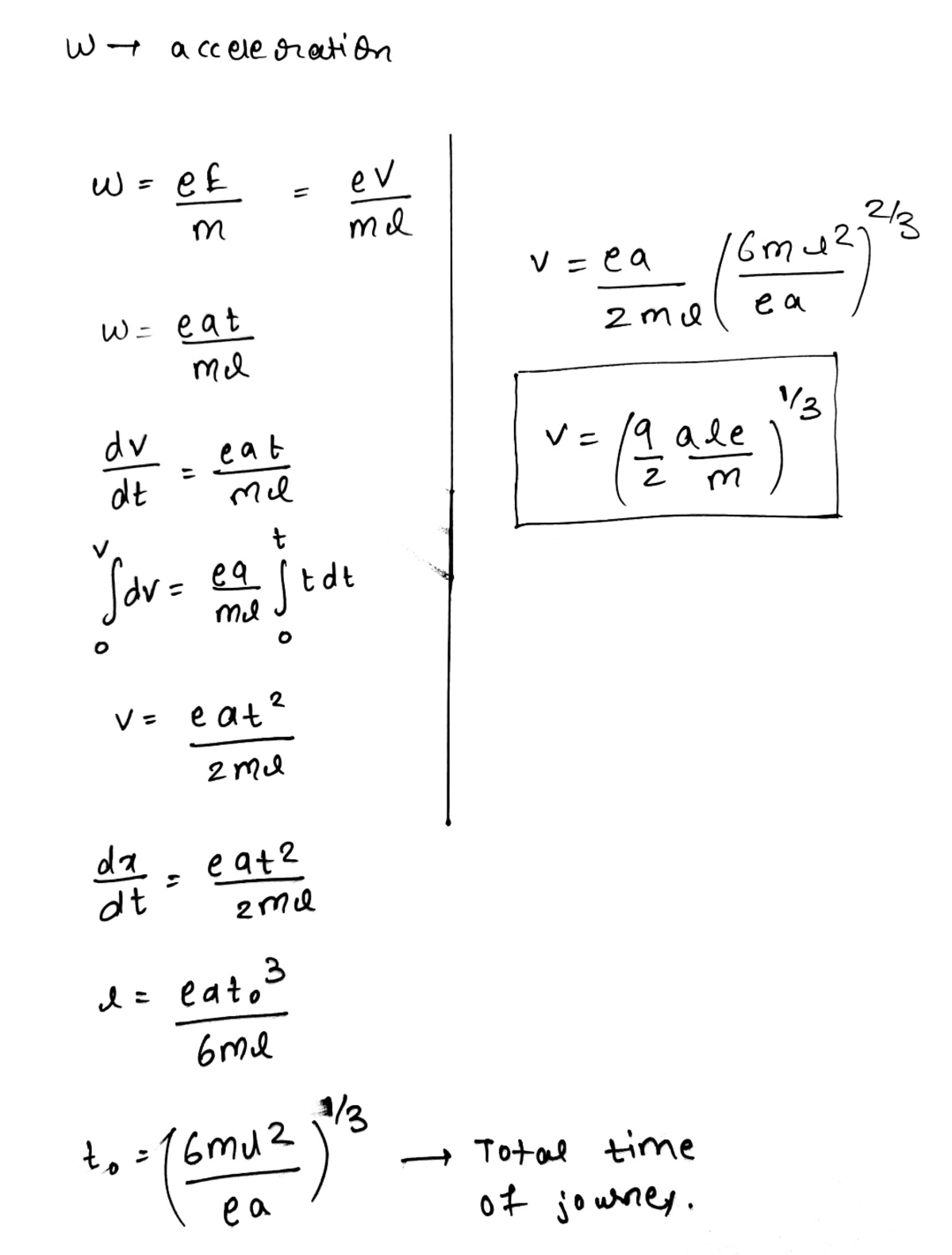
Capacitor Exercise

This was a fun problem. Write Newton's Second Law:

$F = m \ddot{x} \\ q E = m \ddot{x} \\ \frac{q V}{\ell} = m \ddot{x} \\ \frac{q a t}{\ell} = m \ddot{x} \\ \ddot{x} = \frac{q a }{m \ell} t$

The second time derivative of position is proportional to the time. This means that the position is a cubic polynomial in time:

$x = A t^3 + B t^2 + C t + D$

Find expressions for the higher time derivatives:

$x = A t^3 + B t^2 + C t + D \\ \dot{x} = 3 A t^2 + 2 B t + C \\ \ddot{x} = 6 A t + 2 B \\ \frac{d}{dt}\ddot{x} = 6 A$

The position, velocity, and acceleration are all zero initially. This gives:

$x = A t^3$

Compare the third derivative expressions at $t = 0$ :

$6 A = \frac{q a}{m \ell} \\$

Resulting in:

$x = \frac{q a}{6 m \ell} t^3$

Find the collision time with the final plate $(t_f)$ :

$\ell = \frac{q a}{6 m \ell} t_f^3$

Then plug $t_f$ into the velocity equation:

$v_f = \frac{3 q a}{6 m \ell} t_f^2$

Plugging in numbers gives:

$v_f \approx 30530$

Note also that this speed is far below the speed of light (about $10^{-4} c$ ), which means that our Newtonian approach was valid. If the end result had come out to be some significant fraction of the speed of light, we would have had to re-do everything using relativistic dynamics.