Cars on a Straight Road
Cars A, B, C, and D are on a straight road (not necessarily at the same point). They each drive at a constant speed (which is possibly different from each other).
Car A meets
- car B at 10 am
- car C at 1 pm
- car D at 4 pm.
Car D meets
- car B at 12 pm
- car C at 2 pm
- car A at 4 pm (as stated earlier).
What time do car B and car C meet?
Hint:
There is a nice way to represent this problem.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
[See if the hint can help you approach this problem, before reading the full solution below.]
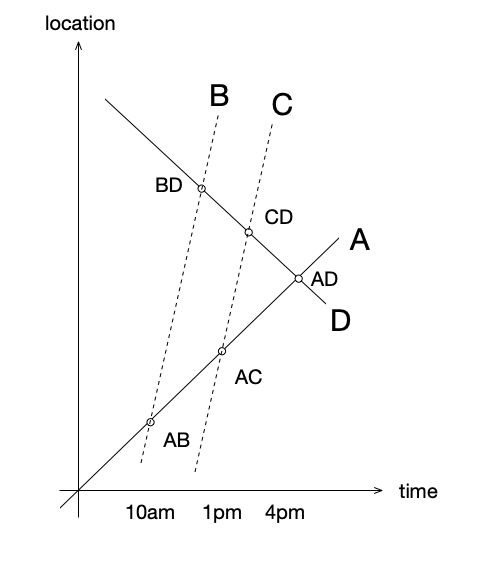
Hint: Draw the location-time graph.
Each car will be represented by a straight line because it travels at a constant speed.
What can we say about the lines representing cars B and C?
On the location-time graph, draw lines A and D to represent the path taken by each car.
Mark out points "AB, AC, AD, BD, BC" at the given times on the given lines, to represent when any 2 of these cars meet.
We know car B is at points AB and BD, and travels in a straight line, so the line representing car B is formed by connecting AB and BD.
Similarly, the line representing car C is formed by connecting AC and CD.
Now, observe that triangles "AD-CD-AC" and "AD-BD-AB" are similar in the ratio 1:2, and so line B is parallel to line C.
This tells us that B and C will never meet (and also that they have the same speed+direction).
Here is an example of what the location-time graph might look like with cars A and D are traveling in opposite directions.
Note: Is it possible that A, B, C, D are all driving in the same direction?
If yes, what additional information can we conclude?
Can you come up with such a graph to show that this scenario is possible?
Note: Can you draw the graphs representing these scenarios:
1) A+D in the same direction with A faster,
2) A+D in same directions with D faster,
3) A stationary,
4) D stationary.
This shows us that we cannot draw any conclusions about the relative speed / direction of cars A and D. They could have been any 2 (non-vertical) lines that we drew on the plane to intersect once at 4pm (at some location). In fact, one of them could have been a horizontal line (meaning that the car is traveling at a constant speed of 0).