Centroids and Circumcenters and Incenters, Oh My!

Say we have a triangle $ABC$ with $\angle B=\angle C$ , so that sidelengths $b=c$ . Let $D$ be the midpoint of $BC$ .

Helpfully, the line $AD$ (the axis of symmetry of the triangle) is simultaneously the median through $A$ , the perpendicular bisector of $BC$ , and the angle bisector of $A$ ; so the centroid, circumcentre and incentre of a triangle all lie on this line. (As a side fact, if these points are collinear, the triangle must be isosceles, but we don't need that here).

The centroid $G$ is easiest to locate: we have $AG=\frac23 AD = \frac23 b \cos \theta$ , where $\theta=\frac12 A$ (this is a standard result)

Now consider the circumcentre $O$ . It lies on the intersection of the perpendicular bisectors of the sides. Say the midpoint of $AB$ is $E$ . Then $\angle AEO=90^\circ$ and $AO=\frac12 b \sec \theta$ .

The incentre $I$ is the intersection of the angle bisectors. The triangle $AIB$ has $AB=b$ , $\angle BIA=\theta$ , and $\angle ABI=45-\frac12 \theta$ .

By the sine rule: $\begin{aligned} \frac{AI}{\sin \angle ABI} &=\frac{AB}{\sin \angle AIB} \\ \frac{AI}{\sin (45-\frac12 \theta)} &=\frac{b}{\sin (180-\angle ABI-\angle BIA)} \\ \frac{AI}{\sin (45-\frac12 \theta)} &=\frac{b}{\sin (135-\frac12 \theta)} \\ AI &=\frac{b\sin (45-\frac12 \theta)}{\sin (135-\frac12 \theta)} \\ AI &=b \cot{45+\frac12 \theta} \end{aligned}$

We want $G$ to be the midpoint of $O$ and $I$ ; that is $\begin{aligned} 2AG&=AO+AI \\ \frac43 b \cos \theta &=\frac12 b \sec \theta + b \tan{45+\frac12 \theta} \\ \frac43 \cos \theta &=\frac12 \sec \theta + \cot{45+\frac12 \theta} \end{aligned}$

At this point, we can make use of the substitution $t=\tan\frac12 \theta$ to rewrite this as

$\frac43 \cdot \frac{1-t^2}{1+t^2} =\frac12 \cdot \frac{1+t^2}{1-t^2} + \frac{1-t}{1+t}$

Cross-multiplying, cancelling and rearranging, this becomes $\left(t^2-8t+1\right)\left(t^2-4t+1\right)=0$

with solutions $t=2\pm\sqrt3$ , $t=4\pm\sqrt{15}$ .

Checking these, we find two triangles that satisfy the condition; one is equilateral - but we disregard this as the three centres are not distinct.

In the other, we find $\tan \frac12 \theta=4-\sqrt{15}$ . In this triangle, $B=C$ are the largest angles; and we find $\sec B=\boxed{4}$ .

Label the sides $\overline{\rm AB}$ , $\overline{\rm BC}$ & $\overline{\rm CA}$ as $c$ , $a$ & $b$ respectively. Draw $\overline{\rm AA'}$ $($ the median from $A$ onto $\overline{\rm BC}$ $)$ , $\overline{\rm OA'}$ , $\overline{\rm AD}$ $($ the perpendicular from $A$ onto $\overline{\rm BC}$ $)$ . Extend $\overline{\rm OG}$ to meet $\overline{\rm AD}$ at $H$ . Draw $\overline{\rm IX}$ $\perp$ $\overline{\rm BC}$ . $H$ is the orthocentre since $O$ , $G$ & the orthocentre lie on the $\textbf{Euler line}$ and $\overline{\rm OG}$ meets $\overline{\rm AD}$ at $H$ .

$\overline{\rm HG}$ $=$ $2$ $\overline{\rm GO}$ $=$ $\overline{\rm IO}$ $[$ $G$ is the midpoint of $\overline{\rm IO}$ $]$ .

$\overline{\rm HG}$ $=$ $\overline{\rm IO}$

Subtracting $\overline{\rm IG}$ from both sides gives, $\overline{\rm HI}$ $=$ $\overline{\rm GO}$ . $\Rightarrow$ $\overline{\rm HI}$ $=$ $\overline{\rm IG}$ $=$ $\overline{\rm GO}$ $\Rightarrow$ $\overline{\rm IO}$ $=$ $2$ $\overline{\rm HI}$

Treating $\overline{\rm OH}$ as a transversal cutting the parallel lines $\overline{\rm OA'}$ , $\overline{\rm IX}$ and $\overline{\rm HD}$ ,we can say that, $\overline{\rm XA'}$ $=$ $2$ $\overline{\rm DX}$ .

$\overline{\rm XA'}$ $=$ $\overline{\rm XC}$ $-$ $\overline{\rm A'C}$ $=$ $($ $s$ $-$ $c$ $)$ $-$ $\frac{a} {2}$

On simplifying, this gives $\overline{\rm XA'}$ $=$ $\frac{b-c} {2}$

$\overline{\rm DX}$ $=$ $\overline{\rm BX}$ $-$ $\overline{\rm BD}$

Let, $\overline{\rm BD}$ $=$ $x$

Now, $c^2$ $-$ $x^2$ $=$ $\overline{\rm AD^2}$

Also, $b^2$ $-$ $(a-x)^2$ $=$ $\overline{\rm AD^2}$

Equating these two equations gives , $c^2$ $-$ $x^2$ $=$ $b^2$ $-$ $(a-x)^2$ $\Rightarrow$ $x$ $=$ $\frac{c^2+a^2-b^2} {2a}$

$2$ $\overline{\rm DX}$ $=$ $2$ $($ $\overline{\rm BX}$ $-$ $\overline{\rm BD}$ $)$ $=$ $2$ $\{$ $($ $s$ $-$ $b$ $)$ $-$ $\frac{c^2+a^2-b^2} {2a}$ $\}$

On simplifying, this gives, $2$ $\overline{\rm DX}$ $=$ $\frac{(b-c)(c+b-a)} {a}$

$\overline{\rm XA'}$ $=$ $2$ $\overline{\rm DX}$

$\Rightarrow$ $\frac{b-c} {2}$ $=$ $\frac{(b-c)(c+b-a)} {a}$ $\Rightarrow$ $(b-c)$ $(2b+2c-3a)$ $=$ $0$

Repeating the same constructions mentioned earlier on the other two sides similarly gives us, $(c-a)$ $(2c+2a-3b)$ $=$ $0$ and $(a-b)$ $(2a+2b-3c)$ $=$ $0$

The triangle can't be equilateral since $I$ , $G$ & $O$ are given to be distinct points. Hence it has to be either isosceles or scalene.

If $\bigtriangleup ABC$ is not isosceles, then,

$2b+2c-3a$ $=$ $0$ $\Rightarrow$ $\frac{b+c}{a}$ $=$ $\frac{3}{2}$ . Similarly, $\frac{c+a}{b}$ $=$ $\frac{3}{2}$ and $\frac{a+b}{c}$ $=$ $\frac{3}{2}$

But then, $\frac{3}{2}$ $=$ $\frac{b+c}{a}$ $=$ $\frac{c+a}{b}$ $=$ $\frac{a+b}{c}$ $=$ $\frac{2\bcancel{(a+b+c)}}{\bcancel{(a+b+c)}}$ $=$ $2$ , which is obviously a contradiction.

Hence, $\bigtriangleup ABC$ is isosceles. Let $b$ $=$ $c$ (WLG)

From the other two equations (which concur into one), we get, $(a-b)(2a-b)$ $=$ $0$

$\Rightarrow$ $2a-b$ $=$ $0$ $[$ Since $a$ $\neq$ $b$ $]$

$\Rightarrow$ $b$ $=$ $2a$

$b$ $=$ $2a$ $=$ $\overline{\rm AC}$ $>$ $a$ $=$ $\overline{\rm BC}$ $\Rightarrow$ $\angle ABC$ $>$ $\angle BAC$

$\angle ABC$ is the biggest angle in $\bigtriangleup ABC$ . Hence, $\sec$ $($ $\angle ABC$ $)$ $=$ $\frac{b}{a/2}$ $=$ $\frac{2a}{a/2}$ $=$ $\boxed{4}$ .

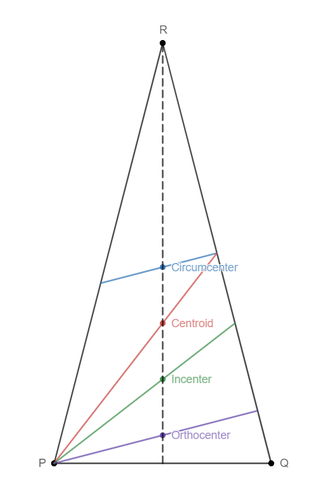
If the centroid is the midpoint of the circumcenter and incenter, and all three points are distinct points, then the three points must be collinear. The orthocenter is already collinear with the circumcenter and centroid (on the Euler line), so if the incenter is collinear with them as well, at least one altitude and one angle bisector are on the same line, which means the triangle must be an isosceles triangle, and the collinear points are on a straight line perpendicular to the base of the isosceles triangle.

Let the base of the isosceles triangle be $2x$ , its legs be $y$ , and its height be $h$ .

Then the sides of the isosceles triangle are $a = 2x$ , $b = y$ , $c = y$ , and $T = \frac{1}{2} \cdot 2x \cdot h = xh$ , and by the Pythagorean Theorem, $x^2 + h^2 = y^2$ .

That means the circumradius is $R = \frac{abc}{4T} = \frac{2x \cdot y \cdot y}{4xh} = \frac{y^2}{2h}$ , which will be positioned at a height of $h - R = \frac{2h^2 - y^2}{2h} = \frac{(2x^2 - y^2)\sqrt{y^2 - x^2}}{2(x - y)(y + x)}$ above the base of the triangle.

It also means that the inradius is positioned at a height of $r = \frac{2T}{a + b + c} = \frac{2xh}{2x + y + y} = \frac{xh}{x + y} = \frac{x\sqrt{y^2 - x^2}}{x + y}$ above the base of the triangle.

Finally, the centroid is positioned at a height of $p = \frac{h}{3} = \frac{\sqrt{y^2 - x^2}}{3}$ above the base of the triangle.

By the midpoint formula, $\frac{(2x^2 - y^2)\sqrt{y^2 - x^2}}{2(x - y)(y + x)} + \frac{x\sqrt{y^2 - x^2}}{x + y} = \frac{2\sqrt{y^2 - x^2}}{3}$ , which simplifies to $(y - 2x)(y - 4x) = 0$ and solves to $y = 2x$ or $y = 4x$ .

The solution $y = 2x$ represents an equilateral triangle in which the centroid, circumcenter, and incenter are not distinct, so it must be that $y = 4x$ , with the base angle as the biggest angle, and the secant of that angle is $\frac{y}{x} = n = \boxed{4}$ .