Chain on a hillock
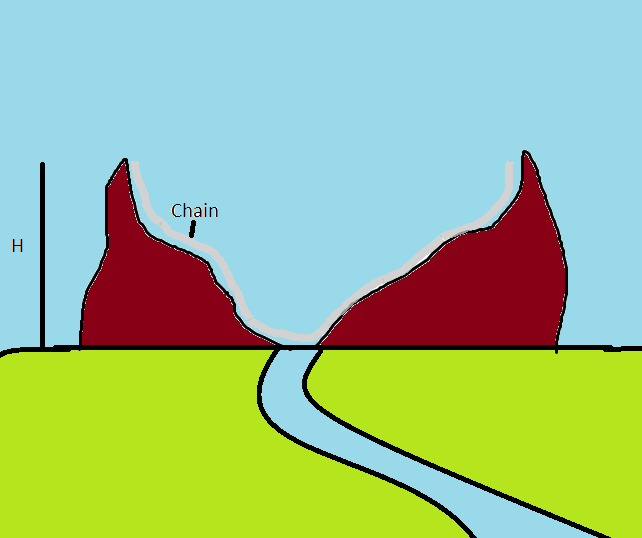 Consider two hillocks adjacent to each other with smooth surfaces,
Consider two hillocks adjacent to each other with smooth surfaces,
They may have any shape, A silver chain lying midway between them initially has "half" of its length on one hillock and half on the other hillock ,, and rises to an equal height 'H" initially on either of them, ( Refer to figure)
The earth as usual has gravitational field.
And the slope of the part where the chains end terminate have slope 90 degree.
You may refer to the figure now
Now if i slightly displace the chain towards the centre and release, find the time period of oscillations.
( in seconds)
DETAILS
Mass= 1
height =1
l= 1
g =10
NOTE
Assume all ideal Conditions.
HINT
internal tension of chain cancels out
It is a free chain.
The answer is 1.40496.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
consider any small element of mass 'dm' of length 'dx'
Let the linear mass density of the chain be ' λ '
Then the forces acting on this small component is dT ( change in tension from right of this component to left) and
dm(g sin (x)) along the hillock
or we have d m g s i n θ − d T = d m a
Now being an inextensible string, also due to lack of friction there is no chance of slacking,
so acceleration of all components have same magnitude but different directions.
Now
being a free chain, its tension at the ends are 0 , (qualititavely it can also be explained as arising from the fact that net tension should cancel out as an internal force so ∫ d T should be 0 when over whole chain it is integrated)
also
g s i n θ d m = g s i n θ λ d l = g λ ( d l s i n θ ) = g λ d y
thus we have
d m g s i n θ − d T = d m a
= g λ d y − d T = a d m
Now when i slightly displace the chain,, the lengths of chain on either hill change by l+dl and l-dl, and height to h+dh and h-dh,
Then integrating expression for part 1 under limits
we get
g λ d y − d T = a d m g λ ( h 2 − h 1 ) = m a ( d o w n w a r d a l o n g t h e h i l l ) f o r h 1 = h + d h a n d h 2 = h − d h w e h a v e − 2 g λ d h = m a o r − m g λ 2 d h = a = − 2 m g λ s i n Φ d l = − 2 l g d l = l ¨ ( r e p r e s e n t s a c c e l e r a t i o n a l o n g l e n g t h )
s o 2 π 2 g l = 1 . 4 0 4 9 6
Now isnt that a beautiful result , it is equivalent to a pendulum with half its length, please correct me if I am wrong