Subtract Out The Hole

Geometry Level 1

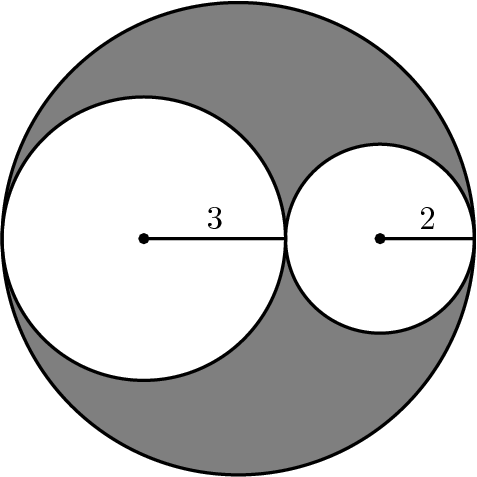
Circles of radii 2 and 3 are externally tangent and are circumscribed by a third circle, as shown in the figure. Find the area of the shaded region.

6 π 6\pi 4 π 4\pi 9 π 9\pi 3 π 3\pi 12 π 12\pi

View wiki
Anish Harsha by Anish Harsha , 20, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

Rishabh Jain
Feb 5, 2016

Radius of the biggest circle = A B 2 = 3 + 3 + 2 + 2 2 = 5 \frac{AB}{2}=\dfrac{3+3+2+2}{2}=5
Hence shaded area= Area of biggest circle - (Area of two smaller circles) \color{#302B94}{\text{Area of biggest circle - (Area of two smaller circles)}}
= π ( 5 ) 2 − ( π ( 3 ) 2 + π ( 2 ) 2 ) \pi (5)^2- (\pi(3)^2+\pi(2)^2) = 12 π \Large 12\pi

11 Helpful 0 Interesting 0 Brilliant 0 Confused

Why you take AB as diameter of bigger circle?

Yashkrit Gupta - 5 years, 4 months ago

Log in to reply

because they are touching externally

Modo 942000 - 5 years, 4 months ago

Log in to reply

Can i get proof of this?

Yashkrit Gupta - 5 years, 4 months ago

Log in to reply

@Yashkrit Gupta – "Circles of radii 2 and 3 are externally tangent" copied from the text

Modo 942000 - 5 years, 3 months ago

Area of shaded region = Area of big circle − Area of two smaller circles. \color{#D61F06}{\text{Area of shaded region}}=\color{#3D99F6}{\text{Area of big circle}}-\color{#20A900}{\text{Area of two smaller circles.}}

Radius of big circle = 3 + 3 + 2 + 2 2 = 5 \color{#624F41}{\text{Radius of big circle}}=\frac{\color{magenta}{3}+\color{magenta}{3}+\color{#EC7300}{2}+\color{#EC7300}{2}}{2}=\color{grey}{5}

Now, Area of shaded region.
⇒ π ( 5 ) 2 − [ π ( 3 ) 2 + π ( 2 ) 2 ] = 12 π \Rightarrow \pi (\color{grey}{5})^2-[\pi (\color{magenta}{3})^2+\pi (\color{#EC7300}{2})^2]=\boxed{\color{#302B94}{12} \pi}

4 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...