Circulisious

Geometry Level 3

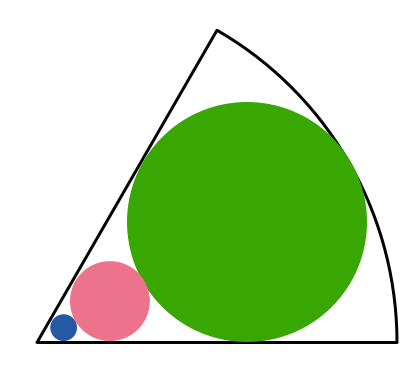
Given a sector of radius 10 and angle π 3 \frac{ \pi}{3} , we draw the largest circle (in green) that is tangent to all sides of the sector. We then draw the circle (in pink) that is tangent to the 2 sides, and also to the green circle. We repeat this process indefinately, where the next circle is shown in blue.

Find the sum of the areas of these infinitely many circles.

25 π 2 \frac{25\pi}2 20 π 2 \frac{20\pi}2 14 π 2 \frac{14\pi}2 17 π 2 \frac{17\pi}2

Gaurav Agarwal by Gaurav Agarwal , 38, Australia

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

1 solution

Ahmad Saad
Mar 2, 2016

r 1 = 1 2 ( 10 − r 1 ) ⇒ r 1 = 10 3 r 2 = 1 2 ( 10 − ( 2 r 1 + r 2 ) ) ⇒ r 2 = 10 9 r 3 = 1 2 ( 10 − ( 2 r 1 + 2 r 2 + r 3 ) ) ⇒ r 3 = 10 27 ⋮ \begin{aligned} r_1 &=& \dfrac12 (10 - r_1) \Rightarrow r_1 = \dfrac{10}3 \\ r_2 &=& \dfrac12 (10 - (2r_1 + r_2)) \Rightarrow r_2 = \dfrac{10}9 \\ r_3 &=& \dfrac12 (10 - (2r_1 + 2r_2 + r_3)) \Rightarrow r_3 = \dfrac{10}{27} \\ &\vdots & \end{aligned}

Sum of these areas form a geometric progression sum :

100 π [ ( 1 3 ) 2 + ( 1 9 ) 2 + ( 1 27 ) 2 + ⋯ ] = 100 8 π = 25 2 π . 100 \pi \left [ \left( \dfrac13\right)^2 + \left( \dfrac19\right)^2 + \left( \dfrac1{27}\right)^2 + \cdots \right ] = \dfrac{100}8 \pi = \boxed{\dfrac{25}2 \pi } .

6 Helpful 0 Interesting 0 Brilliant 0 Confused

can you prove that the sum of the areas form a geometric progression? right now you have noticed a pattern, but that's not a proof. haha i can't seem to find the proof myself which is why im asking you, can you help me out? thanks!!!

Willia Chang - 4 years, 11 months ago

Log in to reply

Are you mean that why an infinite [ (1/3)^2 + (1/9)^2 + (1/27)^2 + ... ] is an infinite geometric series ?

It's known that an infinite geometric series is an infinite series whose successive terms have a common ratio | r | < 1 , r = T2 /T1 = T3 /T2 = T4 /T3 = ...

For above series : T2 /T1 = (1/9)^2 /(1/3)^2 = 1/9 , T3 /T2 = (1/27)^2 /(1/9)^2 = 1/9

then, Its common series = 1/9

Are you meant another my explanation ?

Ahmad Saad - 4 years, 11 months ago

Log in to reply

sorry for not being specific. i meant to ask how do you know the ratio between each of the areas is 1/9th? can you prove that by induction or something?

Willia Chang - 4 years, 11 months ago

Log in to reply

@Willia Chang – method of Mathematical Induction

Let S(n) be a mathematical statement which relates to any natural number of the infinite sequence n = 1, 2, 3, . . . 

Two steps should be done to prove this statement by the method of mathematical induction:

    1) We have to prove that the statement  S(1)  is valid.

    2) We have to prove next implication. 

         If the statement  S(k)  is true, then the statement  S(k+1)  is true,  where  k  is any positive integer number.

If these two steps are done, then the statement  S(n)  is proved for all positive integer numbers  n.

You can review this method by research about ( Mathematical induction and geometric progressions ) in the Internet Sites.

Ahmad Saad - 4 years, 11 months ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...