This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
11 solutions
And yes, you hit on the reason why I set this question. Namely those who used the wrong expansion for a tower of exponents, will think that the values are equal.
Log in to reply
My whole life has been a lie! We are taught, a^b^c = (a^b)^c, and not a^(b^c).
Log in to reply
( a b ) c = a b c , which is exactly why exponentiation is evaluated right-to-left; if it's evaluated left-to-right, then just drop all the exponents down, so the additional notation is useless.
me too...i get confused...
Sir, are you meaning that our teachers are wrong t-o-t-a-l-l-y????????
Sir I am sure that a^b^c = (a^b)^c, and not a^(b^c). Even my IITian friend agrees to this.
Log in to reply
even calculator shows its same
Log in to reply
@Ananya Chattree – Calculators don't know how to calculate :)
As a follow-up question, what value(s) of x satisfy the equation
( x 2 ) ( x 2 − 1 ) ( x 2 − 2 ) = x ( x + 1 ) ( x + 2 ) ?
Log in to reply
You should post that as a separate question (assuming that you have an answer).
Maybe also stipulate that x = 1 isn't allowed?
Log in to reply
When I actually figure out the answer, (I think there is one solution just a bit greater than 1 ), I will post the question. :) If x = 1 then the LHS would be 1 0 − 1 , which would appear to be indeterminate.
Log in to reply
@Brian Charlesworth – "I will post the question. :)" Where is it?
Nice question and nice solution.
Sir , try this out
Log in to reply
Nice problem, NIhar; definitely worth a reshare. :)
I got the A one right but the B one wrong.
Instead of 3^4, I was like 3*4. XD
Hello @Calvin Lin Sir I hit option A but mistakenly got hit B . So is there any way to undo the submitted answer ?
Log in to reply
Unfortunately, no.
Log in to reply
Oops !!! I See . What i was doing just opposite? Thank you ! I realized my fault.
x^n^m= x^nm , A = 4^6 and B= 2^ 12 , A=B=4096 , stop this stupidity plz
A = 4^3^2=128^2= 4096 , B = 2^3^4=8^4=4096 , guess most ppl dont know the rules of exponents (x^n^m = x^nm). Go learn it plz
Log in to reply
No, that's not how the rules of exponents work. Please review Rules of Exponents .
Log in to reply
9^4= 3^2^4=3^(2*4), by that law its = 3^16 , that is wrong ,if an example can prove a simple law , we dont call that as a law,
B A = 2 3 2 ˙ 3 2 4 3 2 = 2 9 ˙ 3 2 2 2 ˙ 3 2 < 1 ⇒ B > A
Moderator note:
A simple 2 2 = 4 kills this question. Well done!
A = 4 3 2 = ( 2 2 ) 9 = 2 2 × 9 = 2 1 8
B = 2 3 4 = 2 8 1
Now,
Since 8 1 > 1 8
Therefore 2 3 4 > 4 3 2
⇒ A < B
Moderator note:
Great. How would you compare if the numbers are 4 5 6 and 6 5 4 instead?
I'm getting really tired of these order of operations questions and they are going to be the downfall of this relatively useful site. This is equivalent to arguing a point using some obscure dictionary to define a word. Just be clear and turn it into a math problem instead of trying to be cute.
This is exactly why these questions are important; apparently, not everyone knows how to do order of operations, and thus they can't be expected to be able to do those problems involving things like that. If you can't determine whether 2 3 4 means 2 ( 3 4 ) or ( 2 3 ) 4 , how are you supposed to solve x ( x + 1 ) x + 2 = 2 8 1 ?
Log in to reply
My point is that after this many years, the order of operations for a tower of exponents has never caught on, arguably because there just aren't many physical systems described by such a function. In general, to justify shorthand there has to be a reasonable expectation of understanding.. That's why you never see many of the words in the dictionary used in prose even if they are a perfect shorthand. Under the same logic, since tower of exponents is still ambiguous to the general population, even if there is a strict definition, it's appropriate to use the parenthesis to remove ambiguity. Either x ( ( x + 1 ) x + 2 ) or ( x ( x + 1 ) ) x + 2 . And that is what you'll see in any reasonable textbook.
Also, wouldn't you rather spend your time on math, over definitions?? Perhaps not, but I would. Regards, Jared
Log in to reply
I've written about the reason why exponentiation is right-associative (evaluated right-to-left) somewhere here; ( a b ) c = a b c , so if exponentiation is left-associative, the additional notation is useless, requiring the much more common use a ( b c ) (which can't be simplified) to be littered with parentheses. Much better to use the additional notation for right-associative. This reason (convenience) is a similar reason why 0 is defined to be even, 1 is defined to be not prime, empty sum is 0 , and empty product is 1 ; you can define otherwise, but you're simply making yourself inconvenient. The general population doesn't know since they don't even care.
By being here, the population has been reduced to "those that are interested on knowing math/[insert other field] better", and thus should actually be exposed to such problems to expand their knowledge. With more knowledge of the definitions, they can understand other people's works better and don't have to bug everyone saying that they think something is wrong when it's only a misunderstanding of definitions. Likewise with textbooks; textbooks no longer matter now, since people here should actually read more than just textbooks, delving into primary sources and stuff.
Log in to reply
@Ivan Koswara – I see your point. But allow me to contend that obscure definitions (to be interpreted as those which are not common knowledge, even by those who use math regularly. The tower of exponents rule, for one, which you agree with and is evidenced by some of the answers on this page) tend to be a source of obscurity in their own right... and that they are only useful once widely accepted.
Until that point, it is useful to provide augmentation, even if redundant. In written English, obscure concepts are frequently followed by a comma and additional words to make the point clear. In math, the language of numbers, so should parenthesis be included. This to me is not litter, but an attempt to convey information (as language's primary role) quickly and efficiently.
I think we simply disagree on the following point. That is to say that it is counter-productive to provide auxiliary information once a definition is defined. As an example I mentioned textbooks. I disagree with your assertion that they are irrelevant, but to strengthen my argument, I will add recent scientific publications in journals such as Science, Nature and Cell, where well established terms, mathematical or otherwise, are proceeded by their definitions for clarity and to save the reader from pausing to "look something up." And as a final piece of evidence, I offer this. Other than math problems for the sake of math problems, I have never seen, in textbooks, journals or otherwise, anyone express an exponent of an exponent without clarifying parenthesis.
A=4^3^2=4^9=2^18 & B=2^3^4=2^81.
2^81>2^18.
so,B>A
very good thinking
thanks.your comment inspired me
A = 4^(3^2) = 4^9 = 2^((2)(9)) = 2^18 B = 2^(3^4) = 2^81
The larger the power(n) for a number larger than 1 ( number denoted as x where x > 1), the larger the value of the final number( x^n)
As 81 > 18, thus B is larger than A
My reasoning was much simpler than what you guys have come up with: I did a quick mental calculation on how many digits there would be for 3 to the 4th power (=two) AND what approximately the number would be (I knew it was eighty-something), which made it intuitive that A can't be bigger, as (1) A's exponent is only 1 digit long and (2) 4 isn't that much bigger than 2, and B's exponent is many times larger than A's. Keep in mind, however, that this strategy wouldn't work for example for 9 to the 9th power vs 2 to the 10th power, as here the former would be the larger number, due to the latter's exponent being only slightly bigger and the base of the former being many times larger.
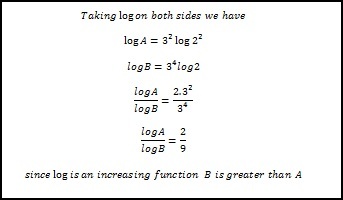
Take log to base 10.Bring the powers down and evaluate
a IS 2 POWER 18 b IS 2 POWER 81
A = 2^18
B = 2^81
Therefore B > A
I did not understand how come A=2^18
Log in to reply
Because some guy wrote it in all caps. That makes it scientific fact.
Unless specified otherwise, the expression a b c is evaluated as a ( b c ) .
Thus A = 4 3 2 = 4 9 = ( 2 2 ) 9 = 2 2 ∗ 9 = 2 1 8 , and B = 2 3 4 = 2 8 1 .
So as 2 8 1 > 2 1 8 we conclude that B > A .
(If the quantities had been expressed as A = ( 4 3 ) 2 , B = ( 2 3 ) 4 then we would have found that A = 4 3 ∗ 2 = ( 2 2 ) 6 = 2 2 ∗ 6 = 2 1 2 and B = 2 3 ∗ 4 = 2 1 2 , making A and B the same.)