Comparing Areas!
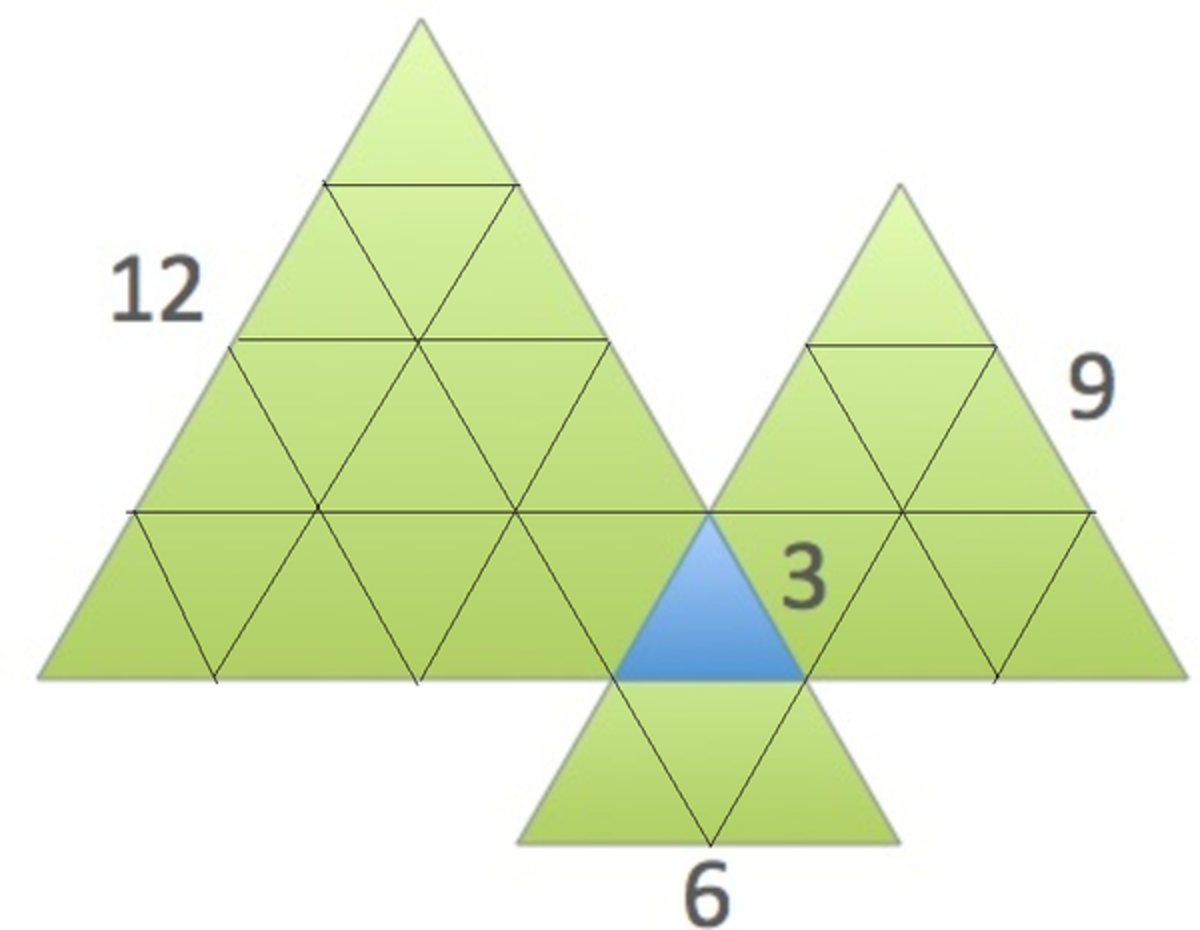
 All triangles shown are equilateral triangles with measures of the sides. What is the ratio of the blue area to the total green area?
All triangles shown are equilateral triangles with measures of the sides. What is the ratio of the blue area to the total green area?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
Thank you. Nice solution.
 A simple solution by dividing the graph into small triangles and notice the comparison.
A simple solution by dividing the graph into small triangles and notice the comparison.
Second Solution:
Area of any Equilateral Triangle is: 4 3 s 2
Area of Green Triangles: 4 3 ( 1 2 2 + 9 2 + 6 2 ) = 4 3 ( 2 6 1 )
Area of Blue Triangle: 4 3 3 2 = 4 3 ( 9 )
Area of Green Region Area of Blue Region = 2 6 1 − 2 7 9 = 2 6 1
Keep in mind that one green triangle is repeated three times : That is why we subtract 2 7 = 3 × 9 from 2 6 1
My explanation is also same as yours, Nice!
The given no.s are sides or area
Log in to reply
I guess the numbers written are the sides, but when it is formed into a grid, it means 1 out of the 26 squares are blue
Log in to reply
I will add more details.
The area of an equilateral triangle is proportional to the square of the side (with a proportionality constant of sqrt(3)/4, which is actually not relevant in this case because it will cancel in the quotient). The green area is the sum of the areas of the big triangles minus three times the area of the blue triangle. Therefore:
A(Blue) / A(Green) = (3^2) / (12^2 + 9^2 + 6^2 - 3*3^2) = 9/234 = 1/26.
Thank you. Nice solution.
I disagree. The amount of overlap between the 2 biggest triangles is undetermined. If they share a vertex, the smallest triangle shares the same vertex and the overlap is 0. If the side 9 triangle is within the side 12 triangle, sharing parts of 2 sides, the side 6 triangle is completely within the side 12 and side 9 triangles, and the overlap is the entire side 6 triangle, in which case the ratio is the area of the side 6 triangle over (the side 12 triangle plus the side 9 triangle minus the side 6 triangle). The ratio therefore can vary between that and zero. Am I missing something?
Log in to reply
It is correct, my solution explains it and Mahdi has a nice visual explanation as well.
Ah yes. I didn’t notice the little 3. Thanks for setting me straight!
Green Triangles Blue Triangle = 2 6 1