Congruent Parts Perimeter

Relevant wiki: Length and Area - Composite Figures - Intermediate

The longer arc must be $\frac16$ of the circumference of the unit circle, that is $\frac{\pi}3$ .

From symmetry, the shorter arc must be $\frac12$ of that or $\frac{\pi}6$ , since $GB$ must be equal to $BF$ .

To get lengths of the three identical straight segments, note that the yellow lines must be the same length, as they play the same role in shapes $CBED$ and $CAGF$ respectively.

The triangle $ABC$ has to be equilateral, so $AH=HI=\frac12$ .

This gives us $AB=\frac1{\sqrt3}$ and $BE=1-\frac1{\sqrt3}$ .

The final result is then the sum $\frac{\pi}3+\frac{\pi}6+3\cdot(1-\frac1{\sqrt3})\approx\boxed{2.8387}$ .

Relevant wiki: Length and Area - Composite Figures - Intermediate

Due to symmetry, the length of the longer arc is $\frac{1}{6}$ the circle's circumference, and the shorter arc is $\frac{1}{2}$ of that.

Also due to symmetry, each of the segments in the figure has the same length. Call this length $x.$ We can extend some segments and connect some points to form a rhombus.

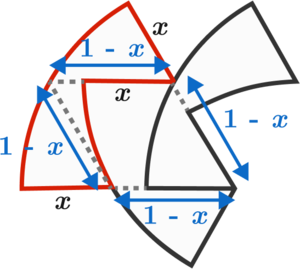
Note that the longer diameter of the rhombus is the circle's radius. Making use of special triangle relationships, we have

$\begin{aligned} \sqrt{3}(1-x) &= 1 \\ x &= 1-\frac{\sqrt{3}}{3} \end{aligned}$

Summing the arc lengths and $3x$ gives $3-\sqrt{3}+\frac{\pi}{2} \approx \boxed{2.839}.$

First, draw line from $A$ to $B$ . If the radius of the circle is $1$ , then $PQ=AB = 1$ also, so that $AC = \dfrac{1}{2}$ .

From this, we can find $AP= \dfrac{1}{\sqrt{3}}$ , so that $QA$ = $1-\dfrac{1}{\sqrt{3}}$

Then the perimeter of each piece is

$(1+\dfrac{1}{2})(\dfrac{1}{6})2\pi + 3(1-\dfrac{1}{\sqrt{3}}) = 2.83875$

Look for (or imagine) $\dfrac{1}{6}$ of radius $1$ circle pizza slices to see how $PQ=AB=1$ and $AC = \dfrac{1}{2}AB$
Consider those pieces that include points $P$ and $A$ as the center of their arcs.