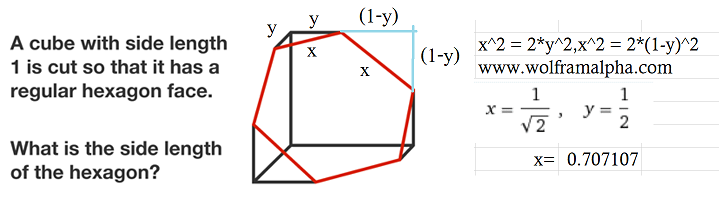
Cross section of a cube and a plane

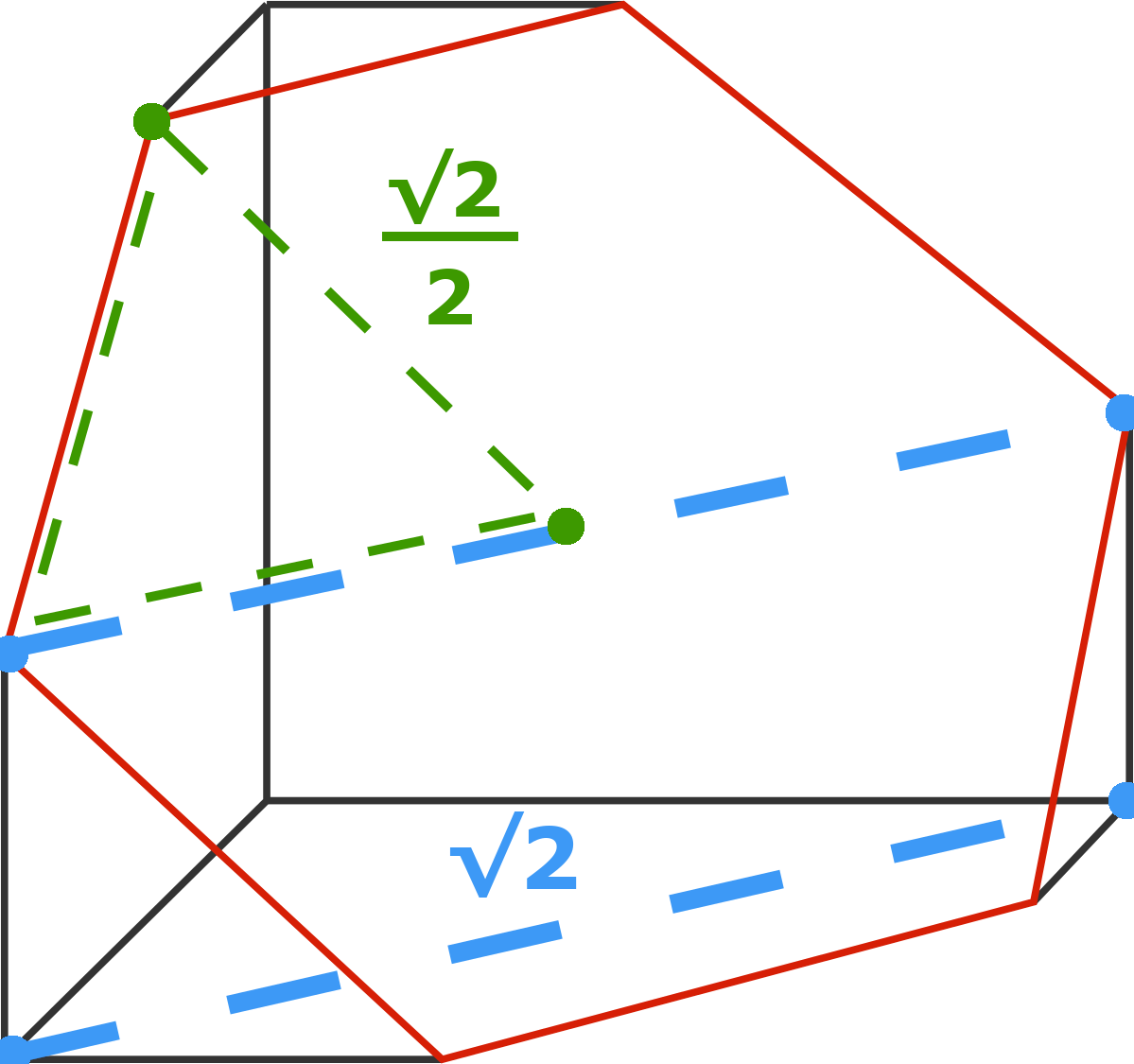We see that the diameter of the hexagon is simply the diagonal of the bottom square. Then the side length is simply half of that.

abusing symmetry, notice that 2 of the same shapes form the original cube.

it follows that the sides of the right isosceles triangle faces are $0.5, \ 0.5, \ 0.5\sqrt{2}$

$\therefore 0.5\sqrt{2}=0.7071$

note: try it yourself, use paint (or any image process software), copy one of them, rotate by 180deg, and overlap them.

Let one vertex of the regular hexagon be $a$ away from one vertex of the cube. Since the cube has a side length of $1$ , the hexagon vertex must also be $1 - a$ away from another vertex of the cube. Continuing in this fashion, each vertex of the regular hexagon must be $a$ and $1 - a$ away from vertices of the cube, as pictured below, so that each side of the hexagon is the same, namely (by Pythagorean's Theorem) $s = \sqrt{a^2 + (1 - a)^2} = \sqrt{2a^2 - 2a + 1}$ .

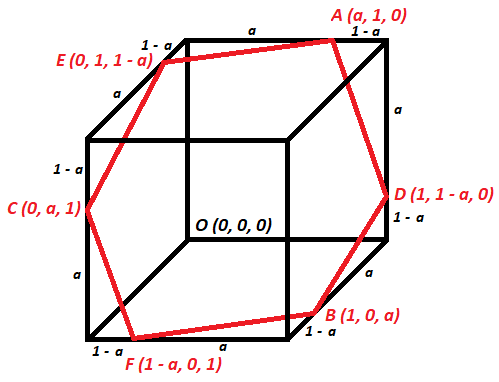
Now all $6$ points of the hexagon must also be in the same plane. Letting one corner of the cube be $O (0, 0, 0)$ , the other vertices can be labeled $A (a, 1, 0)$ , $B (1, 0, a)$ , $C (0, a, 1)$ , $D (1, 1 - a, 0)$ , $E (0, 1, 1 - a)$ , and $F (1 - a, 0, 1)$ , as pictured above. Then vector $AB = (1 - a, -1, a)$ , vector $AC = (-a, a - 1, 1)$ , vector $AD = (1 - a, -a, 0)$ , $AB \times AC = (-a^2 + a - 1, -a^2 + a - 1, -a^2 + a - 1)$ , and $AD \cdot (AB \times AC) = 2a^3 - 3a^2 + 3a - 1$ . In order for $A$ , $B$ , $C$ , and $D$ to be coplanar, $AD \cdot (AB \times AC)$ must equal $0$ , and solving $2a^3 - 3a^2 + 3a - 1 = 0$ gives $a = \frac{1}{2}$ . (For completeness, we can also show that $E$ and $F$ also lie on the plane when $a = \frac{1}{2}$ because the equation of the plane is $x + y + z = \frac{3}{2}$ )

Therefore, side length of the regular hexagon is $s = \sqrt{2a^2 - 2a + 1}$ $=$ $\sqrt{2(\frac{1}{2})^2 - 2(\frac{1}{2}) + 1}$ $=$ $\frac{\sqrt{2}}{2} \approx \boxed {0.7071}$ .

For a regular hexagon from a cube, the cut must be made through the midpoints of the edges and at angle of 45°. As the side of the hexagon ( say $S$ ) is the hypotenuse of the isosceles triangle, by Pythagoras theorem

$S = \sqrt{2} (0.5) = 0.7071$

Here's a view down the long diagonal of the cube.

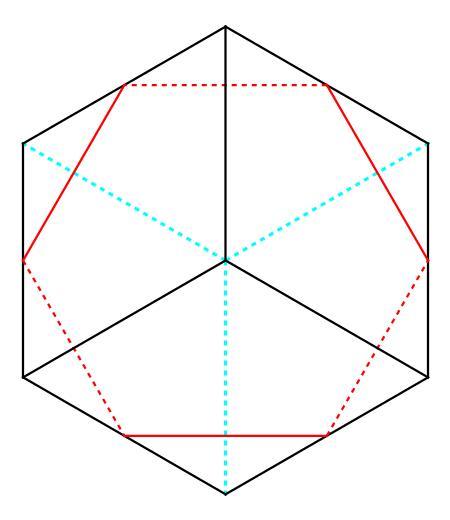
Due to the symmetry of the shape, we know that each corner of the hexagon has the same set of angles with respect to the cube.

As the hexagon edges draw lines on the cube between pairs of perpendicular lines, the only way the angles can be the same is if the angle is $45^\circ$ .

This means we can fold two adjacent faces out like this: Since the angle is $45^\circ$ , the diagonal line has the same length as a line between two opposite corners on a full unit square face. This means its length is $\sqrt{2}$ .

As both halves of the red line are sides of a regular hexagon, they are the same length. This means each hexagon side is $\frac{\sqrt{2}}{2}\approx\boxed{0.7071}$ .

After you slice it, you have two parts of the cube and both of them have the same hexagon . So the two parts of the cube are alike (this is the claim that I am not entirely sure)

Since they are equals, the sides were cut in half , so the sides of same length of the isosceles triangles are 0.5

The hypotenuses (which are the sides of the hexagon) are $\sqrt{\frac{1}{2}}$

The upper edge in the back is cut into two parts: a remains at the top left and b was cut away. Recall that $a+b=1$ . Since the hexagon is regular it holds that an edge of it has the length $c = \sqrt{a^2+a^2} = \sqrt{b^2+b^2}$ . Hence, $a=b=\frac{1}{2}$ and $c = \sqrt{\frac{1}{2}}$ .

Each face intersected by the cut has a right isosceles triangle removed. By symmetry of both the cube and hexagon, all of these triangles are congruent. When when two such sides share an edge, the short legs of the right isosceles triangle bisect the edge. This means the short legs of these triangles have length 0.5. The hypotenuses of these triangles, which are also the sides of the hexagon, have length sqrt(0.5^2+0.5^2)=sqrt(2)/2=0.7071....

This section of cube has 7 faces:

1. 3 isosceles right triangles (congruent)

2. 3 congruent trapezia

3. 1 regular hexagon

Let r = side length of hexagon. Then non hypotenuse sides of triangles are r/√2 each. Now imagine the missing triangular part for each trapezial face. By symmetry, missing triangle ≈ triangular face and consequently we get

(1-(r/√2)) = r/√2

Which gives r ≈ 0.7071.

For all the sides of the hexagon to be equal the corners must be at the centres of the edges of the cube. So the length of the sides is √((1/2)squared + (1/2)squared) = √((1/4) + (1/4)) = √(1/2) =1/√2 = 0.7071.

Here is a simple solution. Analogy: it is a regular hexagon; For all sides of the hexagon to be equal, it should pass through the diagonal of the cube(not the centre diagonal(longest), but the face diagonal). A regular hexagon is made up of six equilateral triangles and all diagonals are equal. So, half of the diagonal should be a side of an equilateral triangle.

Math: Side of cube=1; face diagonal of the cube is $\sqrt2$ . So, the side of the regular hexagon is $\frac{\sqrt{2}}{2}$ ~ 0.7071.

I got the analogy, by looking at my Rubiks cube for a while and imagining cutting it in different angles as shown in the figure.

Side of hexagon=√{(a/2)^2+(a/2)^2}, where 'a' is side of the cube.