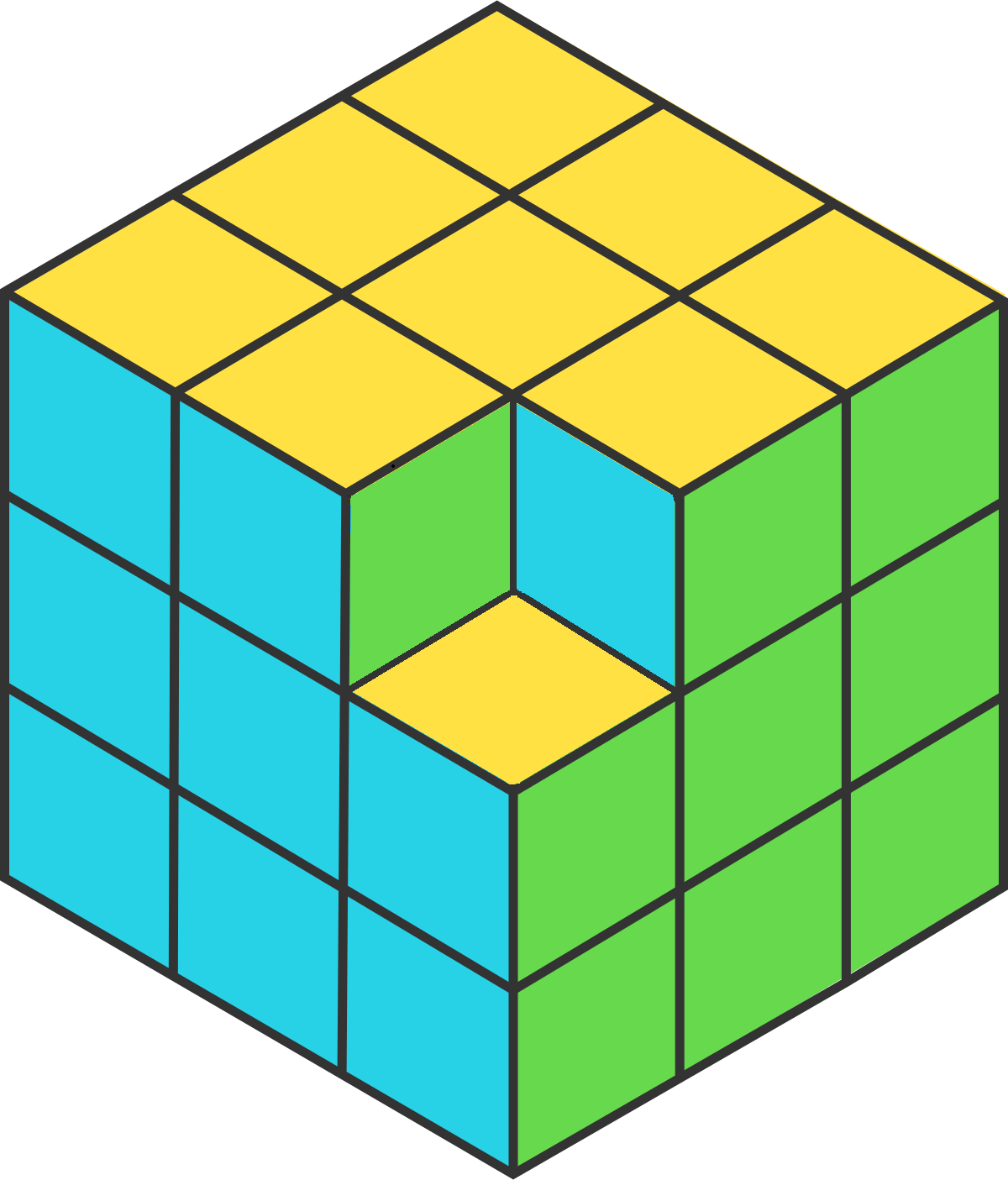
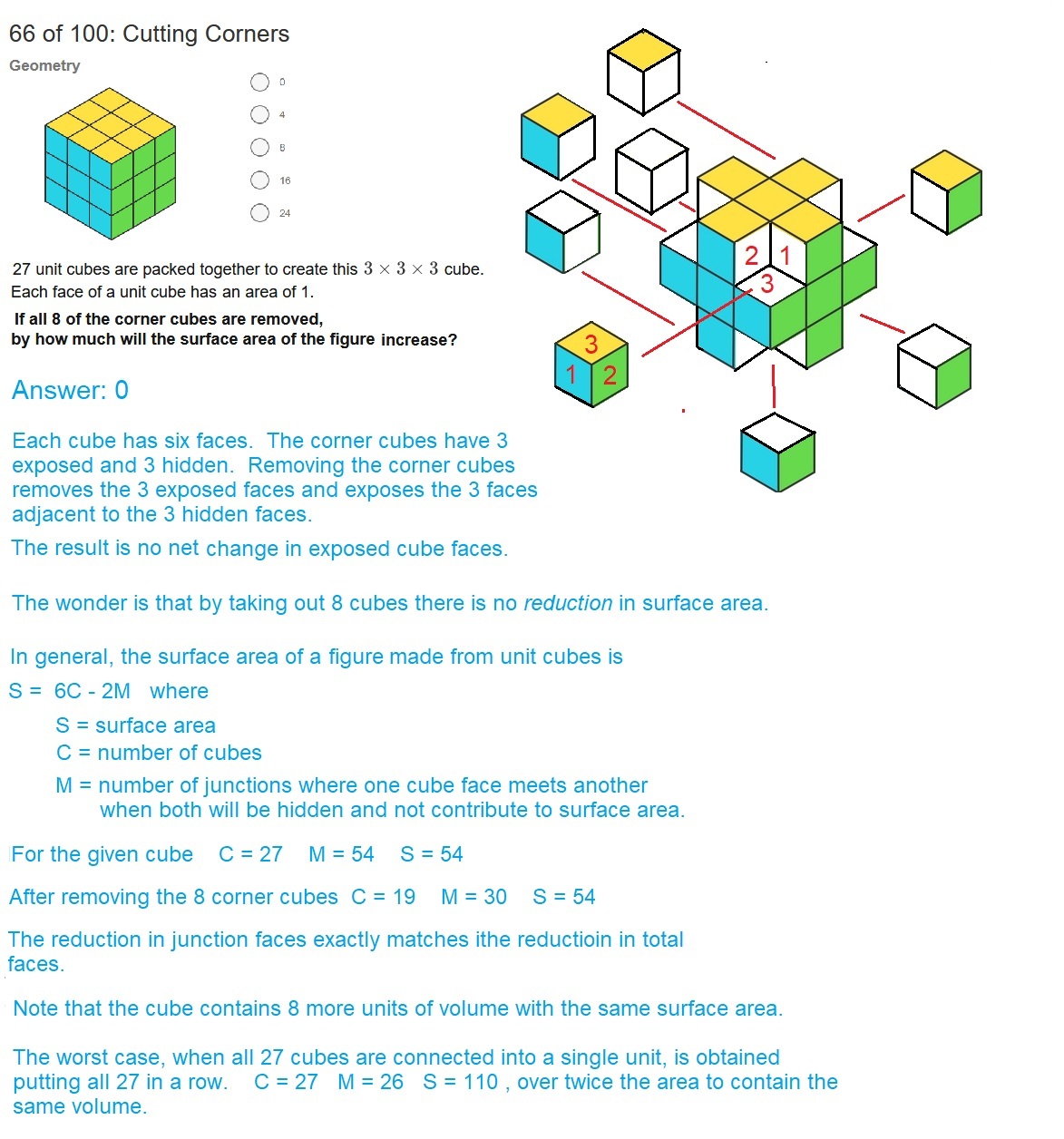
66 of 100: Cutting Corners

Imagine we remove a corner

There were THREE sides before removing

There are still THREE sides after removing

Just remove one unit cube from the top corner.

There will be 3 new surfaces with the loss of 3 old surfaces. So, ultimately total surface area won't change.

This thing will happen for all 8 corner cubes.

So, surface area change will be 0.

if all $8$ of the corner cubes are removed it will be still $3 \times 3 \times 3$ cube.

so,its area will be= $6a^2$ = $3 \times 3 \times 6$ = $54$

so, its surface area will not increase or change

Each cube has six faces. The corner cubes expose 3 and hide 3. Once removed the hidden 3 are exposed when there is no net change in the number of exposed faces. The answer is zero.

The wonder is that after taking out over 1/4 of the cubes, a volume reduction from 27 to 19, there is no -reduction- in surface area !

The surface area for C unit cubes joined face to face into a single unit is given by S = 6C - 2M where S is surface area and M is the number of face to face meetings. Removing the corners reduces C by 8, but also reduces M by 24 (3 per corner) with no net change in surface area. The same surface area now has a volume of only 19.

It remains to show that the cube is the optimal container (made with unit cubes that is) with volume 27 contained in surface area 54 while the worst case is 27 cubes in a row when a surface area of 110, over twice as much, contains the same volume.

For each corner we remove three external faces but reveal other three hidden faces, so we have no area changes.

While, Each face of cube have area 1 than three faces surface area is involve in total 3 x 3 x 3 cube for each small cube. If we remove 1 small cube it will also leave three faces surface area. So, ultimately there will be no change of surface area for removing small cubes from every corner.

If you removed one corner block that would be three sides that you are removing. In its place is also three sides. That is 0 new sides. If you do the same to all of the other corners, each one will be 0. In total it is still 0.

The area of the top of the cube underlying each removed cube is the same as that of each removed cube. The same holds true for each of the adjacent vertical faces revealed when the cubes are removed. Hence, the surface area remains the same whether the corner cubes are removed or left in place. As the surface area does not change, the amount of the increase is 0.

If x is the total surface area of the cube, we could say that $x-24+24$ would equal $x$ , so the surface area would increase by $**0**$

With the original cube, if you look directly at the yellow face you will see 9 squares. If you look directly at any of the other faces you won't see these yellow squares again.

Now remove the eight corner cubes. When you look at the yellow face you will still see 9 squares. You won't see these squares if you look directly at any other face.

This means the surface area hasn't changed.

If, however, you were to remove a strip of three cubes from along one edge (eg the three cubes that have both yellow and green on them) then when you look at the blue face you will only see 8 squares. Here the surface area will decrease.

If you instead remove the centre cube from one of the faces there are parts of the new shape that you cannot see if look directly at any of the faces, so the surface area will increase.

A cube is the same as no cube

The area of one of the faces of each small cube is 1/9. So, if we remove the corner cubes,on each face we are left with 5 of these for a surface area on each face of 5/9. There are 6 faces . So the total surface area (without the corner cubes) is 6 * (5/9) = 30/9. Now the area exposed due to the removal of each corner cube is 3 * (1/9) and there are 8 of these for a total surface area of 8 * (3/9) = 24/9, for a combined area of (30+24)/9 = 6 which is the original surface area.