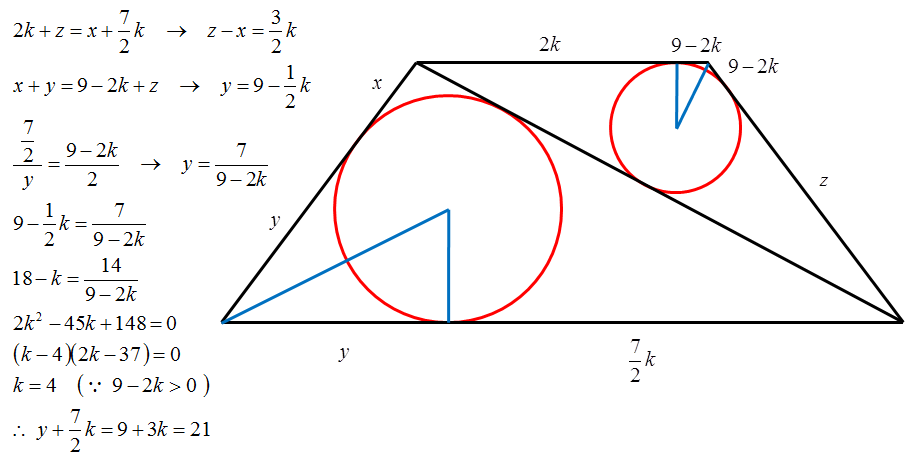Circles Packed Into A Trapezoid
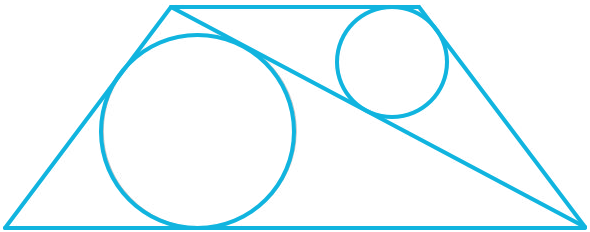
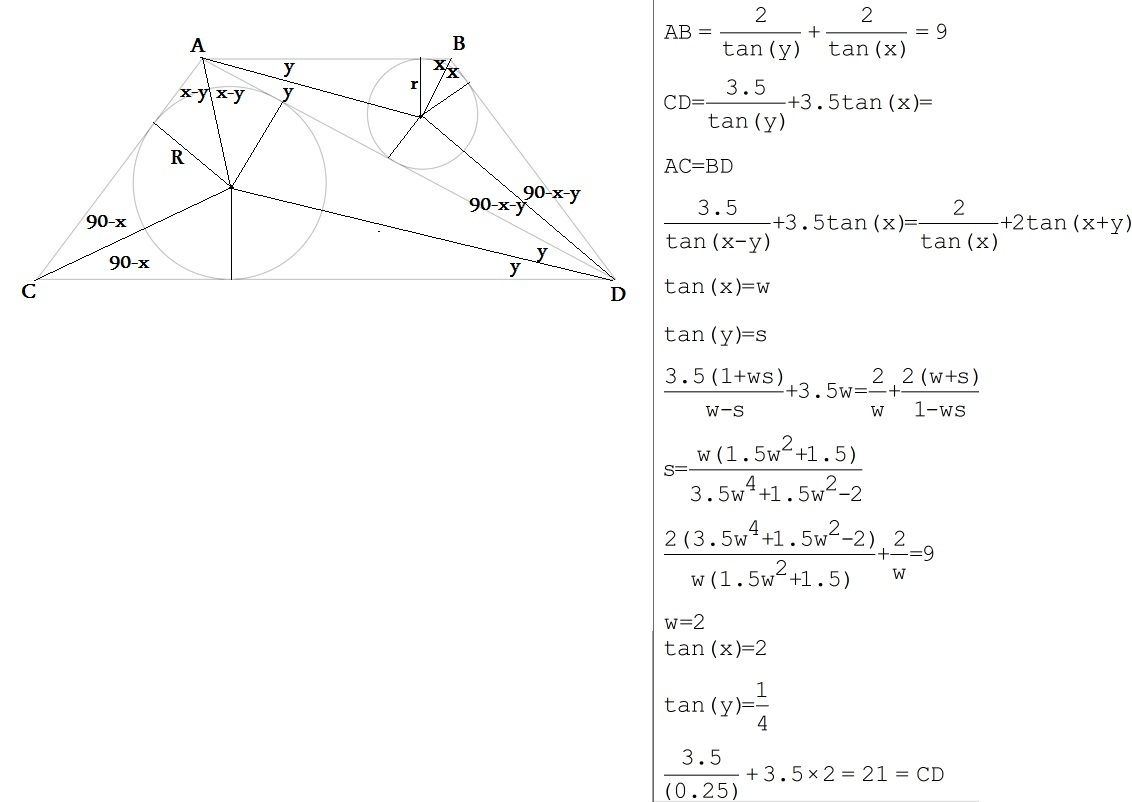
The diagram shows an isosceles trapezium divided into two triangles each inscribing a circle. Given that the length of the upper base of the trapezium is 9 and the diameters of the circles are 4 and 7, find the length of the lower base.
The answer is 21.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
@Ahmed Moh AbuBakr , we have converted your report into a solution. If you subscribe to this solution, you will receive notifications about future comments.
Log in to reply
How? ?????
@Brilliant Mathematics ... Can you edit this ... I made a mistake by clicking on the button of viewing solution
Log in to reply
You can edit your own solution by clicking the "Edit" button.
Log in to reply
@Brilliant Mathematics – No ... I am talking about the problem I clicked the button of viewing the solution
@Ahmed Moh AbuBakr, what software did you use?
Log in to reply
A latex programming .... But now I use a better program (math type) better than this
If we feel inspired, and guess that the base has length 2 1 and that the trapezium has height 8 , then simple Pythagoras shows that two other sides have length 1 0 , and that the two diagonals have length 1 7 . The figure is full of ( 6 , 8 , 1 0 ) and ( 8 , 1 5 , 1 7 ) Pythagorean triads.
That a triangle with sides 9 , 1 0 . 1 7 has inradius 2 , and that a triangle with sides 1 0 , 1 7 , 2 1 has inradius 3 . 5 is easy to check.