Dimples

If you'd do that, it would hardly qualify as carving 'dimples'.

I just did the math. It's not too complicated. Let's just say that the original sphere has a radius of 2.5 inches. If you cut a section of the sphere, which I call "section cap", with a radius of .2 inches, you will need to fill it with a dimple, which is the same as a section cap. The original SA of our sphere is 78.53981634 square inches. When we remove 1 section cap that is with a radius of .2 inch, the sphere's total SA will decrease by 0.125865414 square inches.

Not over yet! The hole needs to be filled with a new dimple. The new dimple has to have the same radius to fit in the hole. We can replace the original section cap with a new section cap from a larger sphere. Our larger sphere's radius is 3 inches. It's section cap with the same .2 inch radius would have an SA of 0.125803644 square inches. The new section cap (dimple) can fit in the same spot as the original section cap.

Since 0.125865414 square inches is greater than 0.125803644 square inches, the new total SA of our original sphere would be less after you add the new dimple SA to the original sphere's SA including the hole. If you're curious, the new SA is 78.53975457 square inches. 78.53975457 is smaller than 78.53981634. Just barely, but it is! That's just with one dimple. If you replace more original section caps with new section caps, the total SA will get even smaller.

The reverse is true if you use a new section cap from a sphere with a radius smaller than the original sphere.

Here is a more formal approach.

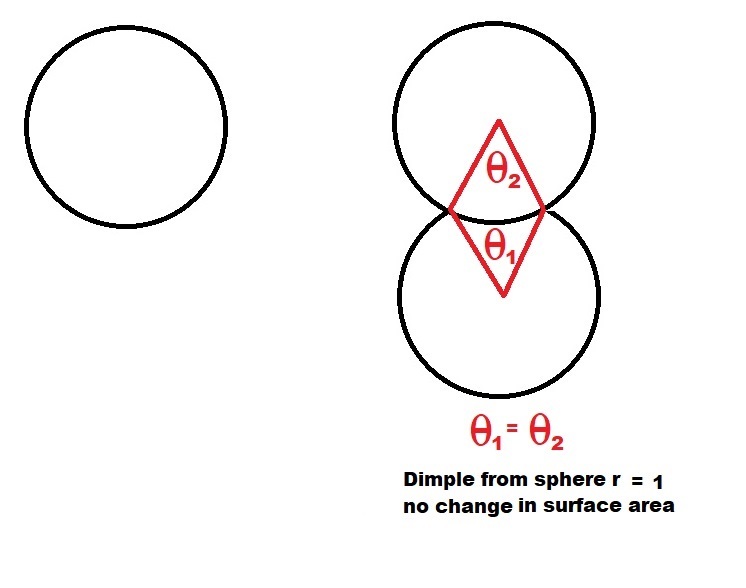
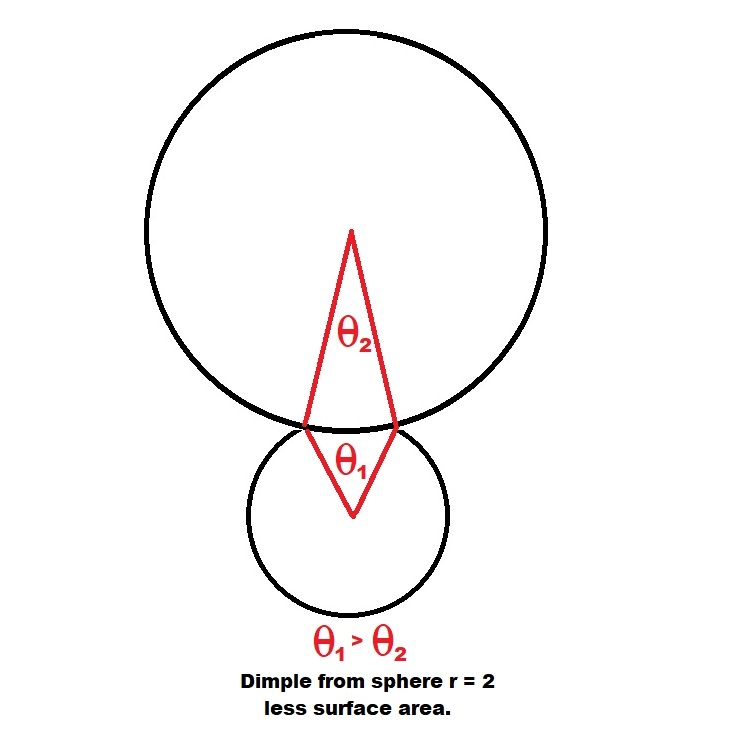
Let $R$ be the radius of the sphere, $r$ the radius of a dimple, and $rho$ the radius of the circle $C$ where the dimple intersects the sphere.

The part of the surface cut away by making the simple has the shape of a " spherical cap ". Its maximum height above the circle $C$ is $h_1 = R - \sqrt{R^2 - \rho^2},$ and the area of the cap is $A_1 = 2\pi Rh_1 = \pi(\rho^2 + h_1^2).$

The area that is cut away is replaced by the inside surface of the dimple, which is also a spherical cap. $h_2 = r - \sqrt{r^2 - \rho^2},\ \ \ A_2 = 2\pi rh_2 = \pi(\rho^2 + h_2^2).$ Thus carving the simple causes a change in the area of the sphere of $\Delta A = A_2 - A_1 = \pi(\rho^2 + h_2^2) - \pi(\rho^2 + h_1^2) = \pi(h_2^2 - h_1^2);$ it is easy to see that $\Delta A < 0$ if $h_1 > h_2$ and therefore $R < r$ .

This proves the claim that "dimpling" the sphere lowers its surface area provided that the radius of curvature of the dimples is greater than that of the sphere.

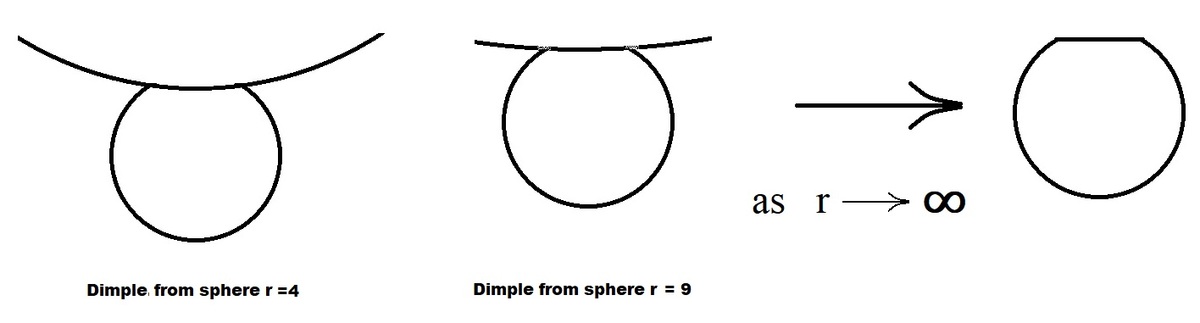
In an extreme case, we can choose $r \to \infty$ , which makes the "dimple" a flat circle of radius $\rho$ . Then $h_2 = 0$ and $\Delta A = -\pi h_1^2 < 0.$

The area-volume connection is certainly profound--

but what I like about this problem is that it addresses a misconception of how to apply that connection. The fact that 65% of people checks the wrong answer brings that out nicely.

Your counterexample makes my point... and a picture really helps! By making your big dimple, you replace the area shown in red by the area shown in green. This is clearly smaller!

Good question... but if you reflect the dimple about a flat surface (the only way to preserve its area), the reflected dimple might have a smaller curvature than the ball itself. You would still be carving stuff away!