Disks vs hoop wheel
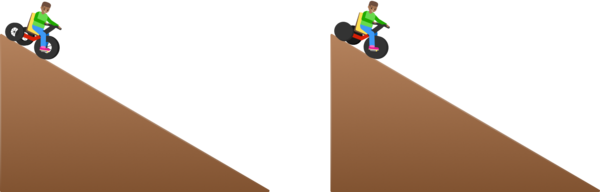
On the left, a cyclist rolls down a hill without pedaling and without using his brakes. On the right, he does the same but has replaced his wheels with disks.
Which way is faster?
Details and Assumptions:
- The disks are uniform and have the same mass and radius as the wheels.
- The friction is sufficient to avoid slipping of the wheels.
- Neglect the mass of the spokes.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
8 solutions
if the friction is the same, why should we consider the extra forces?
Log in to reply
I am not sure what extra forces you are talking about. All the forces are assumed to be the same. The difference is the distribution of mass.
Log in to reply
'I' will be different by distribution of mass, then the produce of friction will be differed accordingly, but the condition set for friction is no skip, then assuming the friction just the same in all time, that also means that mass distribution problem has been taking care of...
If the wheels and disks have the equal mass.....
I considered the problem to be unanswerable without knowing the relative rolling resistances. If the disk material is softer than the tires it will flatten more under the weight of the vehicle, slowing it down considerably. If the disks are very hard that will reduce the rolling resistance more than the difference in inertia will compensate for. I am assuming the tires are inflated to a normal pressure but that, of course, is another variable.
I had thought that both the wheel and disk has uniformly distributed mass. How were we to know that the wheel had greater density near its surface than near its center.
Log in to reply
Uniformly distributed around the circle, but one is concentrated at the circumference of it, the other is uniformly distributed over the area.
For the same mass M and R , the disk has a moment of inertia of I d = 2 1 M R 2 , while that of a wheel I w ≈ M R 2 .
Neglecting air resistance and friction, the loss in potential energy going down the hill is converted into the gain in kinetic energy, that is
M T g h = 2 1 I T ω 2 + 2 1 M T v 2 = 2 1 ( R 2 I T + M T ) v 2
where
- M T is the total mass of the cyclist and bike,
- g , the acceleration due to gravity,
- h , the height drop going down hill,
- I T , the total moment of inertia,
- ω , the angular velocity of the wheels/disks,
- R , the effective radius of the wheels/disks, and
- v , the velocity of the cyclist and bike going downhill.
Since the other quantities are constants, the disks, having almost half the I T of the wheels, results in a large v . The disks is the faster way.
Sorry for being picky but this proves only that the terminal velocity (at the bottom of the hill) is higher in case of the disk. Needs one more step to show that the travel time is shorter. (Which way is faster?)
Log in to reply
Well you can compare the accelerations of the two setups.. it's intuitive that the one with higher acceleration will have a smaller time t for moving down incline as initial velocity is 0 and distance traversed is same.
also acceleration of COM in each case is a= g/(1+I/MR^2) where I is moment of inertia about the Centre of mass(COM) of disk/ring
The question should make it clear that the slope of the hill is constant, as pictured. If the slope can change, you can construct hills that would favor the wheels over the disks. The increased moment of inertia can become a speed advantage if there are stretches where friction overtakes gravity (as pinewood derby racers all know).
we are assuming that dostribution of mass is uniform for both discs and wheels? Otherwise this equation will not hold true.
Great in theory. In reality, the difference in the inertia is negligible unless the load is nonexistenting. Ie, the kid on the tricycles has a lot more mass then the tire or disk.
Can u provide me the easier explanation or solution.....
Log in to reply
Actually, the momentum of inertia of disk is lower than that of wheel. Therefore, for the same energy, the disk is faster.
Log in to reply
But then 1/2 I omega^2 term should be larger right?
Log in to reply
@Kushal Thaman – Why? Should it be larger?
Every summer vacation on our family vacation we visit a science museum somewhere. Every single one of them has some sort of demonstration of this phenomenon.
The point is the wheels have more mass further away from the center of rotation, which makes them harder to turn, so the disks can get spinning more quickly.
This is good way of visualising the answer.
Then why we use wheels everywhere and not disks?
Log in to reply
I think it is because, in real life a disk would weigh more than a wheel due to the increased amount of material needed to achieve the same structural integrity. Just guessing though.
I agree with Patrik Ekenberg, Assume if have to remove mass from edges of wheel and have to transform it as a disc then strenght would be compromised because mass should be fixed and we can't borrow it from outside of wheel.
Imagine trying to break with disks which have very little friction in comparison to normal rubber tires ...RIP
Agreeing with other answers, and also sometimes we want the flywheel effect of a larger moment of inertia so when a wheel gets going, it keeps going despite gaps in force being applied. So if there's a power stroke, the wheel continues to the next power stroke, like on a bicycle or engine.
Is this still alright if the mass and the disk have same mass? Cause it means that we cannot reduce the problem to the center of mass.
A reason cyclists use disks is aerodynamics, not so? I would thought that might eventually also play a part where the air hits each spoke separately.
then why don't they make disks for cars instead of wheels ?
Because of non uniform mass distribution, the rolling inertia of wheel is more than of disk, therefore, wheel is left behind as compared to disk.
What i think of, is that the wheels will have more air resistance than the disks, because the disks are closed to the center.
Since the mass of the wheels would be much smaller than the total mass of the bike and its rider, this effect may very well be more significant than the effect of the moment of inertia of the wheels. It depends on the length of the hill though. On a short hill the bike will not gain much speed on its way down and thus not have much air resistance at all.
The moment of inertia of Disk for mass M and radius R is MR^2/2. Moment of inertia -MR^2
Inertia equation-> I (alpha) = (Torque) where alpha = D2(theta)/d2(time) -> Time is inversaly proportional to I So, disk will reach fast compare to Ring
I have a question: if he uses disks with same mass & mass distribution as the wheels, but the difference now is the disks can slip on the surface, then what would be the answer?
Your assumption contradicts! If both wheel and disk have the same mass, then the disk must have less mass density (solid has more volume than hollow!). You can only pick one to conserve, but not both.
Intuitively you just have to know the greater the entropy is in a compact geometry the greater the speed it is in uniform mass.
The difference is in the distribution of mass relative to the axle of the wheel or disk.
The wheel has its mass on the outside, while the disk has mass throughout.
Mass on the outside contributes more toward the moment of inertia of the object, so the wheel has a higher moment of inertia.
An object with higher moment of inertia takes longer to get in motion given equal forces.
So the wheel will take longer and therefore be the slower alternative.