Dynamic Geometry: P74
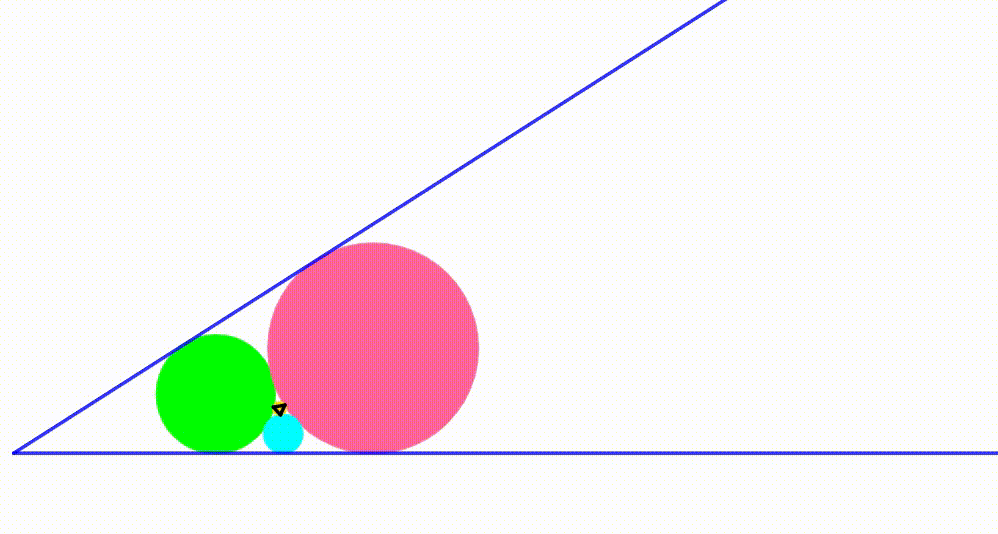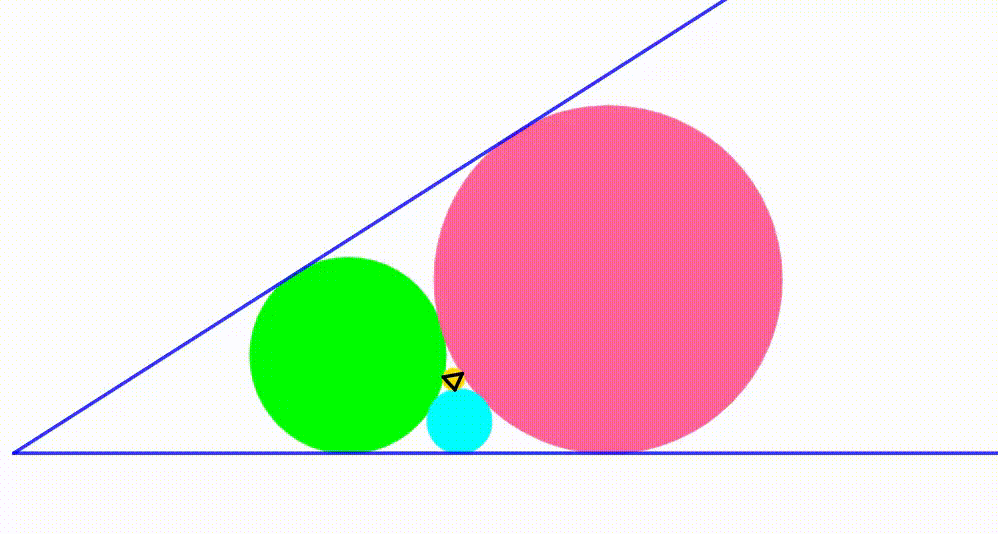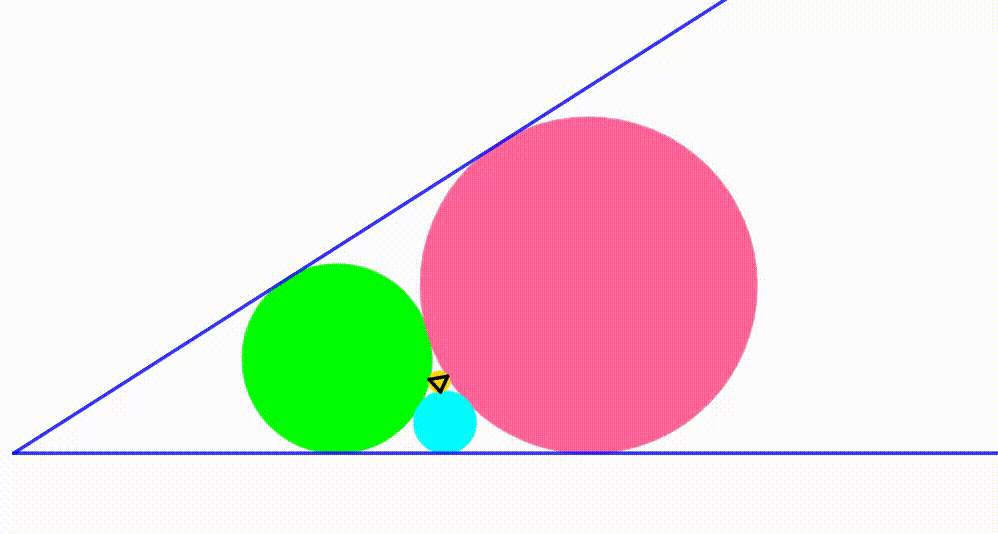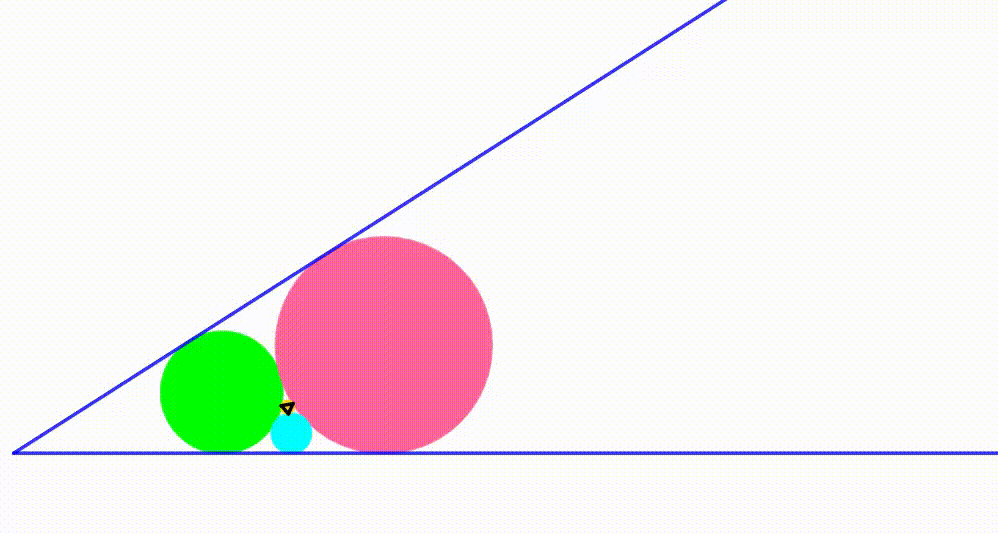 The diagram shows a blue angle which measures
tan
−
1
(
5
2
7
3
3
6
)
. Four circles (green, cyan, red and yellow) tangent to each other and internally tangent to the angle are moving freely. Using the tangency points between the yellow circle and the three other circles, we draw a black triangle. When the area of the black triangle is equal to
8
0
7
7
8
, the area of the cyan circle can be expressed as
q
p
π
, where
p
and
q
are coprime positive integers. Find
p
+
q
.
The diagram shows a blue angle which measures
tan
−
1
(
5
2
7
3
3
6
)
. Four circles (green, cyan, red and yellow) tangent to each other and internally tangent to the angle are moving freely. Using the tangency points between the yellow circle and the three other circles, we draw a black triangle. When the area of the black triangle is equal to
8
0
7
7
8
, the area of the cyan circle can be expressed as
q
p
π
, where
p
and
q
are coprime positive integers. Find
p
+
q
.
The answer is 89465.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
Great work !
@Valentin Duringer I have seen that arctan(336/527) almost in every question with the diagram above or similar diagram , is there something special about that angle ?
No, nothing special, I'm just using the same templates but I ask a different question !
It is special because tan θ = 5 2 7 3 3 6 ⟹ tan 2 θ = 2 4 7 ⟹ tan 4 θ = 7 1 . It is chosen so that we get a rational number as solution. Since tan 2 θ = sin θ 1 − cos θ , to get rational tan 2 θ , both sin θ and cos θ must also be rational. For all sin θ , c o s θ , and tan θ to be rational, it means that the right triangle has Pythagorean triple as side lengths. In this case they are 336-527-625 and 7-24-25.
Log in to reply
Well, I could have chosen any Pythagorean triple ;)
Log in to reply
But this is two levels. tan θ = 4 3 ⟹ tan 2 θ = 3 1 ⟹ tan 4 θ = 1 0 − 3 only one level.
Let the angle measuring tan − 1 5 2 7 3 3 6 be ∠ P O Q = θ , the centers of the green, red, cyan, and yellow circles be A , B , C , and D ; and their radii r 0 , r 1 , r 2 , and r 3 respectively, and A M and B N be perpendicular to O Q .
We note that ∠ B O N = 2 θ and tan 2 θ = sin θ 1 − cos θ = 2 4 7 . This means that similar △ A O M and △ B O N are 7 - 2 4 - 2 5 triangles. By similar triangles:
A M B N r 0 r 1 2 5 r 1 ⟹ r 1 = O A O B = O A O A + A B = 7 2 5 r 0 7 2 5 r 0 + r 0 + r 1 = 3 2 r 0 + 7 r 1 = 9 1 6 r 0
We can find r 2 using the equation, r 2 1 = r 0 1 + r 1 1 = r 0 1 + 4 r 0 3 ⟹ r 2 = 4 9 1 6 r 0 . And r 3 by Descartes' theorem ,
r 3 1 ⟹ r 3 = r 0 1 + r 1 1 + r 2 1 + 2 r 0 r 1 1 + r 2 r 3 1 + r 2 r 0 1 = 3 7 4
Let the triangle the yellow circle circumscribed be E F G . We can first find the areas of △ D E F , △ D F G , and △ D G E and then sum them up. We find that: the areas of △ D A B and △ D E F are given by:
⎩ ⎪ ⎨ ⎪ ⎧ [ D A B ] [ D E F ] = 2 ( r 0 + r 3 ) ( r 1 + r 3 ) sin ∠ A D B = 2 r 3 2 sin ∠ A D B ⟹ [ D E F ] = ( r 0 + r 3 ) ( r 1 + r 3 ) r 3 2 [ D A B ] = ( r 0 + r 3 ) ( r 1 + r 3 ) r 3 2 ( r 0 + r 1 + r 3 ) r 0 r 1 r 3 By Heron’s formula
Similarly for [ D F G ] and [ D G E ] , then
[ E F G ] ⟹ r 0 2 = [ D E F ] + [ D F G ] + [ D G E ] = ( r 0 + r 3 ) ( r 1 + r 3 ) r 3 2 ( r 0 + r 1 + r 3 ) r 0 r 1 r 3 + ( r 1 + r 3 ) ( r 2 + r 3 ) r 3 2 ( r 1 + r 2 + r 3 ) r 1 r 2 r 3 + ( r 0 + r 3 ) ( r 2 + r 3 ) r 3 2 ( r 0 + r 2 + r 3 ) r 0 r 2 r 3 = 2 3 8 1 6 9 9 9 2 r 0 2 + 1 1 4 4 3 7 3 6 0 8 0 r 0 2 + 2 9 8 8 4 9 1 6 3 2 r 0 2 = 1 2 6 8 0 8 9 1 8 9 4 4 r 0 2 When [ E F G ] = 8 0 7 7 8 = 8 0 7 7 8 × 1 8 9 4 4 1 2 6 8 0 8 9 = 2 3 6 8 1 5 7
And the area of the cyan circle A c y a n = π r 2 2 = ( 4 9 1 6 ) 2 × 2 3 6 8 1 5 7 π = 8 8 8 3 7 6 2 8 π . Therefore p + q = 8 9 4 6 5 .
Reference: Heron's formula