Evaluate

Let $x = 2y-3$ . We note that:

$\begin{aligned} 4y^2-22y + 25 & = 0 \\ 4y^2-12y + 9 - 10 y + 16 & = 0 \\ (2y-3)^2 - 5(2y-3) + 1 & = 0 \\ \Rightarrow x^2 - 5x + 1 & = 0 \\ x + \frac{1}{x} & = 5 \end{aligned}$

Now, we have:

$\begin{aligned} (2y-3)^3 + \frac{1}{(2y-3)^3} & = x^3 + \frac{1}{x^3} \\ & = \left(x + \frac{1}{x}\right) \left(x^2 + \frac{1}{x^2} - 1 \right) \\ & = \left(x + \frac{1}{x}\right) \left( \left[x + \frac{1}{x} \right]^2 - 2 - 1\right) \\ & = 5 (5^2 - 3) \\ & = \boxed{110} \end{aligned}$

$\begin{aligned} 4{y}^2-22y+25&=0\\4{y}^2-12y+10&=10y-15\\(2y-3)^3+\frac1 {(2y-3)^3}&={\left(2y-3+\frac{1}{2y-3}\right)}^3-3\left(2y-3+\frac{1}{2y-3}\right)\\&={\left(\frac{{(2y-3)}^2+1}{2y-3}\right)}^3-3\left(\frac{{(2y-3)}^2+1}{2y-3}\right)\\&={\left(\frac{4{y}^2-12y+10}{2y-3}\right)}^3-3\left(\frac{4{y}^2-12y+10}{2y-3}\right)\\&={\left(\frac{10y-15}{2y-3}\right)}^3-3\left(\frac{10y-15}{2y-3}\right)\\&=5^3-3.5\\&\Huge\color{#3D99F6}{=\boxed{110}} \end{aligned}$

If $4y^2-22y+25=0$ then, using the quadratic formula, $y=\frac{1}{4}(11\pm\sqrt{21})$ . Substitute $y=\frac{1}{4}(11+\sqrt{21})$ into the second expression and arrive at $55+12\sqrt{21}+55-12\sqrt{21}$ which equals $110$ .

I did this solution the painful way, here is my solution

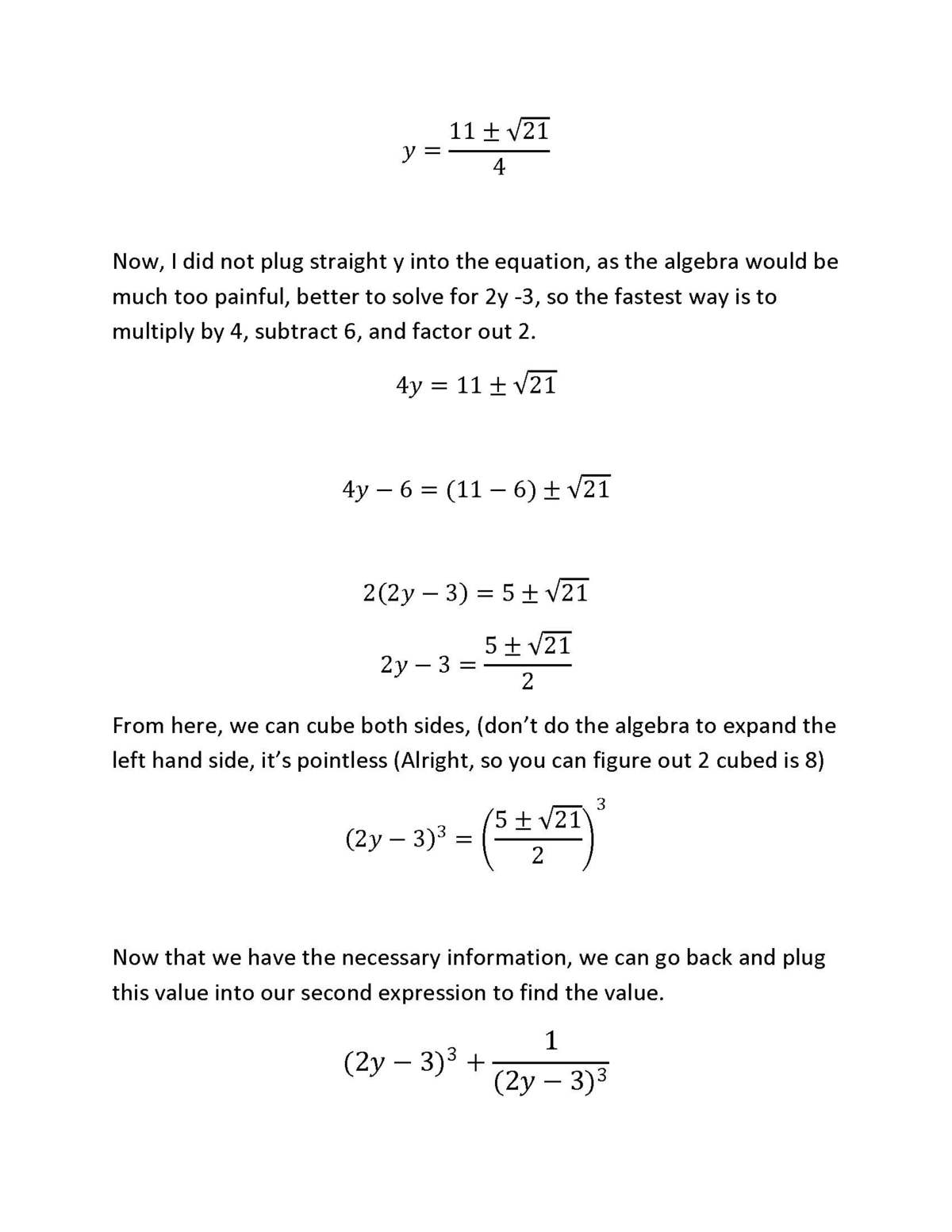
4y^{ 2 }-22y+25=0,\ y=\frac { -(-22)\pm \sqrt { (-22)^{ 2 }-\quad 4(4)(25) } }{ 2(4) } \Longrightarrow \frac { 11\pm \sqrt { 21 } }{ 4 } \ Substituting\quad into\quad equation\quad gives\quad answer\quad :\quad \boxed { 110 }

I did things the hard way. I inserted y values in smaller and smaller increments until my answers came close to zero. Ending up getting close when inserting the y value of y = 1.605 into the second problem. I guessed the answer from there until I got 110 on my last guess.

The answer is also 125. The parabola has 2 roots! quadratic equation: [22+/-sqrt(484-400)]/8=> y=1.60 and y=3.89. Since neither of the roots make the second equation undefined, there are two answers: (2y-3)^3+1/(2y-3)^3=> 110 when y=1.60 & 125 when y=3.89.