Fit that Curve; The End

Geometry Level 5

Find the largest positive integer $n$ such that we can fit infinitely many quartic curves through any $n$ points in $\mathbb{R}^2$ .

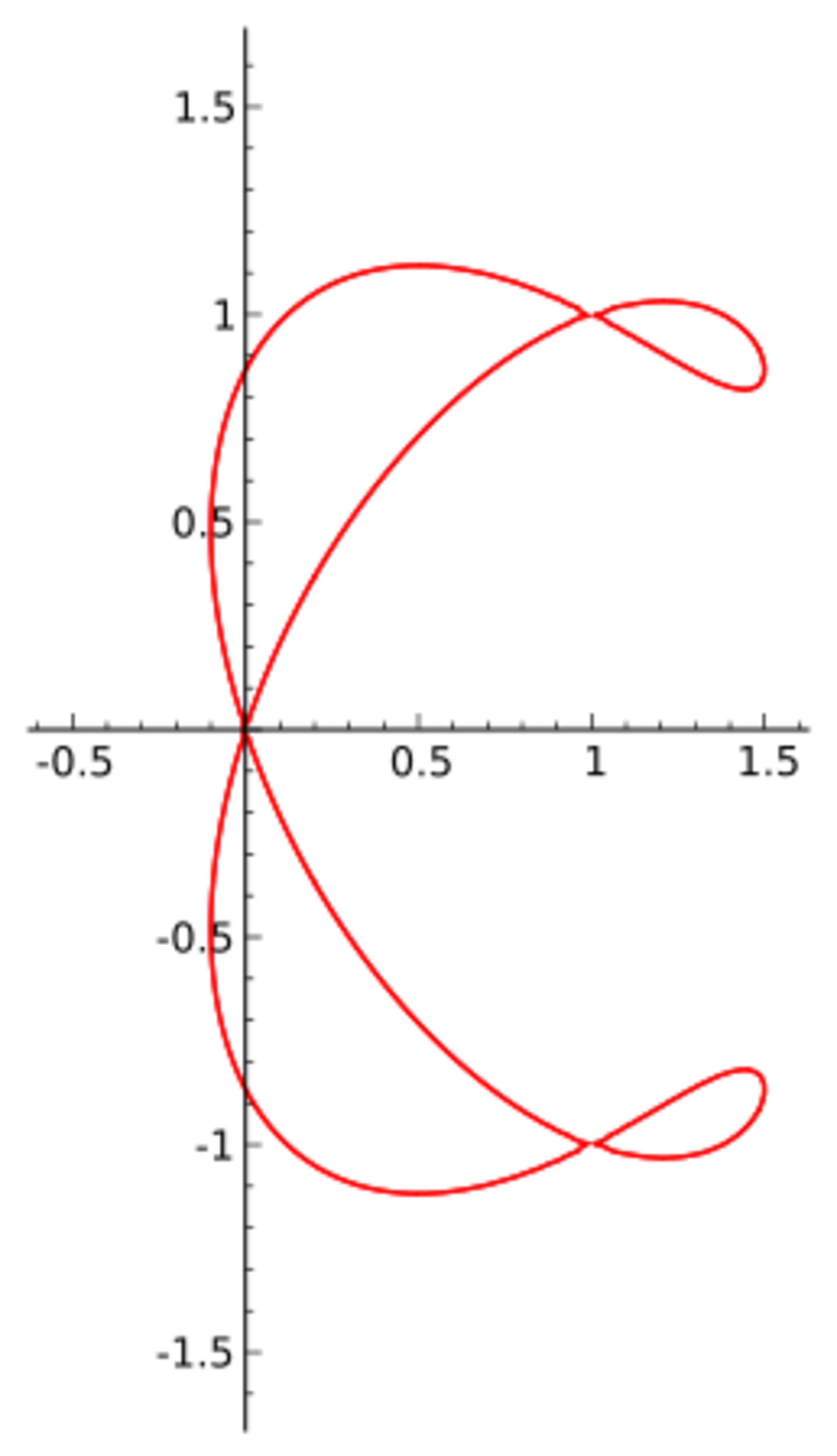
The figure shows a special quartic known as an Ampersand Curve

Picture credit: Krishnavedala, Wikimedia Commons

15 13 14 16 Does not exist

1 solution

Michael Mendrin
Mar 30, 2016

Given $\frac{1}{2}n(n+3)$ arbitrary distinct points, a $n$ th order plane curve can always be found to pass through all of them. Hence, with $\frac{1}{2}n(n+3)-1$ fixed points, the last point, being a variable, can be used to define an infinite family of $n$ th order plane curves passing through all the fixed points. For quartics, $n=4$ , and so the answer is $13$ .

Yes, that's a good way to think about it! (+1) For a complete solution, I suppose we would have to show that it is not always possible to fit infinitely many quartics through 14 given points.

Otto Bretscher - 5 years, 2 months ago

The argument for that would be similar to arguing that it's not possible to "fit infinitely many lines through 2 given points", unless we're talking non-Euclidean geometry. Whatever argument we can use to show that there's only a single straight line through 2 distinct given points, we can generalize for higher order algebraic planar curves.

Michael Mendrin - 5 years, 2 months ago

The situation is not quite analogous as it is impossible to fit more than one line through two distinct points while it may well be possible to fit infinitely many quartics through 14 distinct points... we are dealing with the subtle issues of "general position" that my countrymen Euler and Cramér were discussing in their correspondence in the 1740s (now known as the Cramér-Euler Paradox )

Otto Bretscher - 5 years, 2 months ago

@Otto Bretscher – Okay, I remember that now. Cubics sharing the same 9 points, for example. So, for quartics, they can intersect in 16 points, any 14 of them sharing those quartics, so that the solution is not unique. But I believe this wouldn't always be the case for "any" $n$ given points, i.e., only some sets of $n$ given points allow multiple $n$ th order curves to pass through all. So, what if we were to propose a problem like, "9 points are chosen at random inside an unit square---what's the probability that more than one cubic pass through all?" Hmm...lemmie think on that.

It should be noted that this doesn't arise with conics, since they at most can intersect at 4 points, but they require 5 points for an unique conic to pass through all.

Michael Mendrin - 5 years, 2 months ago

@Michael Mendrin – You are raising some subtle and interesting questions.

The fact remains that for a complete solution of this problem we need to show that there exist 14 points in the plane such that there is a unique quartic curve running through them.

Otto Bretscher - 5 years, 2 months ago

@Otto Bretscher – Otto, I hadn't been taking seriously your recent series of questions about n-order curves going through given points until just now. Suddenly, this has become VERY interesting. Thanks for bringing this to my attention! It's fun to even think about this.

Michael Mendrin - 5 years, 2 months ago

@Michael Mendrin – very interesting indeed!

Otto Bretscher - 5 years, 2 months ago

@Michael Mendrin Sir, could you please tell me how you cam up with that formula???

Aaghaz Mahajan - 2 years, 9 months ago

Fit that Curve; The End

The figure shows a special quartic known as an Ampersand Curve

Picture credit: Krishnavedala, Wikimedia Commons

1 solution

0 pending reports