Don't Just Guess, Try To Prove!

Geometry Level 2

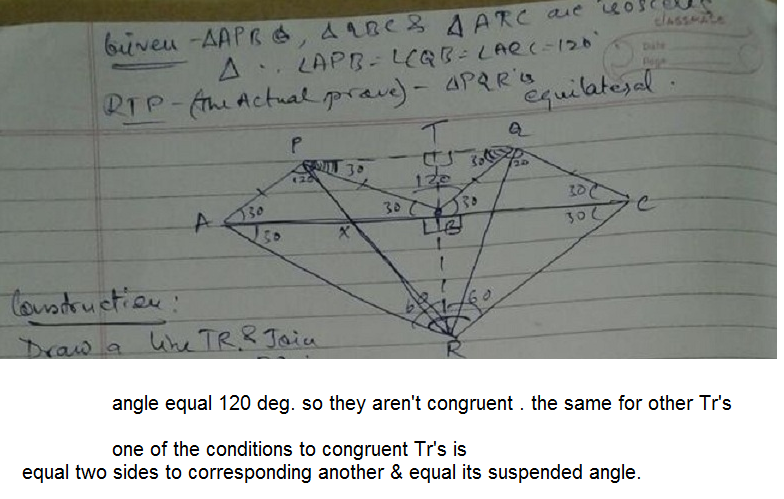
Let $AC$ be a line segment in the plane and $B$ a point between $A$ and $C$ . Construct an isosceles triangle $PAB$ and $QBC$ on one side of the segment $AC$ such that $\angle APB= \angle BQC=120^\circ$ and an isosceles triangle $RAC$ on the other side of $AC$ such that $\angle ARC=120^\circ$ . Find the measure of $\angle PRQ$ in degrees.

30^{\circ}

45^{\circ}

60^{\circ}

70^{\circ}

80^{\circ}

3 solutions

Ahmad Saad
Apr 30, 2016

Relevant wiki: Similar Triangles - Problem Solving - Medium

Extend $PB$ and $QB$ to meet $CR$ and $AR$ at points $M$ and $N$ respectively. Join $QM$ and $PN$ .

$APBN$ and $CQBM$ are rhombus , each divided into two equilateral triangles.

Figure above shows that $PN=PA=MR, QM=QC=NR$ and $\angle PNR = \angle QMR = 120^\circ$ , then the two triangles $PNR$ and $RMQ$ are congruent,

then $\angle NRP + \angle MRQ = 60^\circ$ .

$\angle PRQ = 120^\circ- 60^\circ =\boxed{ 60^\circ}$ .

In response to Mr. Sattik Biswas:

Very nice. But how can you say those are rhombus? And according to the problem point B will be the midpoint of AC. Though your solution is very impresive. Thank you.

Sattik Biswas - 5 years, 1 month ago

BQ//MC , QC//BM & PQ=MC ---> CQPM is a parallelogram having equal its four sides.

then, CQPM is a Rhombus . similarly for APBM is a Rhombus.

Note that the problem don't state that point "B" is a midpoint of segment AC , but state that point "B" is between "A" & "C" . That is meaning that position of point "B" is a randum on segment AC not line AC.

Thanks.

Ahmad Saad - 5 years, 1 month ago

Yes it is not written than M is the midpoint. But those two triangles are congruent, and hence AB and BC are equal, which implies M is the midpoint.

Sattik Biswas - 5 years, 1 month ago

@Sattik Biswas – Sorry, the problem didn't stated that the two triangles APB , BQC are congruent.

Ahmad Saad - 5 years, 1 month ago

@Ahmad Saad – No it is not stated but I can prove it.

Sattik Biswas - 5 years, 1 month ago

@Sattik Biswas – I have proven it in my solution.

Sattik Biswas - 5 years, 1 month ago

@Sattik Biswas – My reply have been attached to my previous solution.

Ahmad Saad - 5 years, 1 month ago

Triangle PAB and triangle QBC are congruent. By AAA criteria.

Sattik Biswas - 5 years, 1 month ago

Sorry, there isn't any thing to refere that in statment of problem or your proof.

You can present that to any mathematician or staff of this site.

Ahmad Saad - 5 years, 1 month ago

Khoa Đăng
Apr 30, 2016

Thank you for your solution. Could you please elaborate it.I mean it would have been nice if you could explain the steps. Thank you.

Sattik Biswas - 5 years, 1 month ago

Sattik Biswas
Apr 30, 2016

Here is the proof of it.

Moderator note:

This assumes that we are in the special case where B is the midpoint of AC, and doesn't constitute a rigorous solution.

Note that B is a point on AC, and not necessarily the midpoint of AC.

Calvin Lin Staff - 5 years, 1 month ago

Yes, but we can prove that.

Sattik Biswas - 5 years, 1 month ago

No, you are unable to prove that. B is any point on AC. E.g. B could be the point A (which leads to a degenerate case), but we still have $\angle PRQ = 60 ^ \circ$ .

If you assume it is the midpoint, you can find the numerical answer in this case. However, it doesn't represent a solution in all cases.

Calvin Lin Staff - 5 years, 1 month ago

@Calvin Lin – Got it. I am terribly sorry Calvin.

Sattik Biswas - 5 years, 1 month ago

Don't Just Guess, Try To Prove!

3 solutions

Moderator note:

0 pending reports