Passel Of Techniques

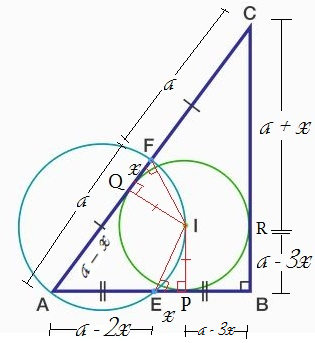
(1) As $AI$ is a bisector then, we can say that $\angle FAI=\angle IAE=\alpha$ .

(2) Now, $AEIF$ is a ciclic quadrilateral $\implies$ $\angle IEF=IFE=\alpha$ and $\Delta IFE$ is isosceles.

(3) $F$ and $E$ are midpoints then $FE \parallel BC$ and $\angle AEF=90^\circ$ implies $\angle AFE=90^\circ-2\alpha$ implies $\angle AIF=90^\circ-\alpha \implies \angle IFC = 90^\circ+\alpha$ .

(4) As $H$ is a intersection of the bisector $AI$ and $\Delta ABH$ is a triangle rectangle then $\angle AHB=90^\circ-\alpha \implies \angle AHC=90^\circ+\alpha$ .

(5) As $CI$ is a bisector of $\angle ACB=90-2\alpha$ then $\angle AIC=\angle ICB=45^\circ-\alpha$ and as $\angle AHC=90^\circ+\alpha=\angle IFC$ an share $CI \implies \Delta FIC \succeq \Delta ICH$ then $\angle FIC= \angle IHC= 45^\circ$ .

(6) As $\Delta FIC \succeq \Delta ICB$ then $IF=IH=IE$ then $H,F,E$ are cyclic then $\angle FIH$ is central and $\angle FEH=45º \implies \angle HEB=BHE=45^\circ$ then $\Delta HEB$ is isoceles and $EB=BH$ .

(7) $EB=BH$ . and for (5) $CF=CH$ then if $EB=x$ and $CH=y$ we have that

$4y^{2}-4x^{2}=x^{2}+y^{2}+2xy \implies 5x^{2}+2xy-3y^{2} = 0 = (5x-3y)(x+y)$ but $x+y$ are not 0 then $5x-3y=0 \implies 5x=3y$ .

Now, $\frac{BC}{AB}=\frac{x+y}{2x}=\frac{1}{2}+\frac{y}{2x}=\frac{1}{2}+\frac{5}{6}=\frac{16}{12}=\frac{4}{3}$ then $p+q=4+3=7$

Let $BC=a, AC=b$ and $AB=c.$ Construction : Draw $ID$ perpendicular to $AC.$
Then $ID=r,$ the inradius of $\triangle ABC.$ Observe $EF$ is parallel to $BC$ [Mid-point theorem] and hence $\angle AEF = \angle ABC = 90^\circ.$ Hence $\angle AIF = 90^\circ.$
Therefore ${ID}^2 = F D\times DA.$ If $a > c,$ then $F A > DA$ and we have $DA = s - a,$ and $FD = FA - DA =\dfrac{b}{2}-(s-a).$
Thus we obtain $r^2=\dfrac{(b+c-a)(a-c)}{4}$ but $r=\dfrac{c+a-b}{2}.$ From these two equations we get ${(c + a - b)}^2 = (b + c - a)(a - c).$ Simplification gives $3b=3a+c.$ Squaring both sides and using $b^2 = c^2 + a^2,$ we obtain $4c = 3a.$ Hence $\dfrac{BC}{BA} = \dfrac{a}{c} = \dfrac{4}{3}.$
Therefore, the sum of numerator and denominator $=4+3=\boxed{7}.$
Note: If $a ≤ c,$ then I lies outside the circumcircle of $\triangle AEF.$

Note:If $a ≤ x,$ then I lies outside the circumcircle of $\triangle AEF.$