Half, Half, Half
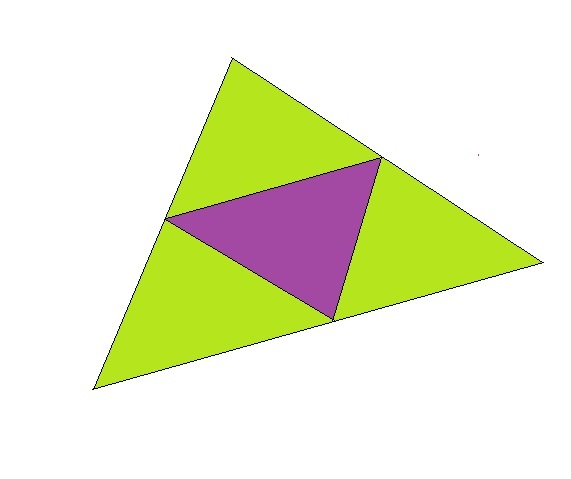
If we pick the 3 midpoints of the sides of any triangle and draw 3 lines joining them, will the new triangle be similar to the original one?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
4 solutions
very poor wiki. Where do you even explain above how similarity works -_-
According to the Mid point theorem, if we join the mid-points of two sides of the triangle, the resulting line segment is parallel to the third side of the triangle and is equal to 1 / 2 of the the third side.
Let us say that the larger triangle is Δ A B C and the smaller one is Δ D E F . Thus, applying mid point theorem to all three corresponding sides we arrive at:
D F A B = E F B C = E D A C = 2 1
Now using the SSS criterion for similar triangles we have proved that they are similar.
That's great! Want to add this problem to Wiki's example? ;)
Log in to reply
Looks like you won the race in posting the solution and your solution is a lot better, +1!
I don't know sir that's up to you, you may add it as a try it yourself in the wiki.
Log in to reply
Alright. See you in the next question then! LOL...;)
ABC be a triangle. DEF form another triangle such that D, E, F are midpoints of AC, BC, AB resp. Since, Lines, DE, FE, and FD are passing through midpoints, by mid-point theorem DE || AB, FE || AC, FD || BC. In such a case, AFED, BFDE, CEFD are all a parallelogram (opposite sides are parallel). So, ∠ A = ∠ E , ∠ F = ∠ C , ∠ B = ∠ D . So DEF and ABC are equiangular. So, two triangles are similar. A simple, elementary proof :)
In 'fancier' terms, it is a spiral similarity about centroid with ratio = − 2 1 ;).
Thank you. I can see my head spinning in 180 degrees now. ;)
Log in to reply
I have one related to this question. Have you tried? Converging Points ;)
Log in to reply
No, not yet. Yours is definitely way tougher than mine. LOL...;)
Log in to reply
@Worranat Pakornrat – It's only a little generalization of yours XD Same idea.
Consider points D & E to be the midpoints of A B & A C respectively, as shown above. Then the △ A D E will share one same angle and has 2 sides of half the ratio as the △ A B C . In other words, △ A D E is similar to △ A B C . As a result, the segment D E will be half of B C due to similarity ratio.
Hence, if we join the midpoints of △ A B C in similar fashion, all the three sides of △ D E F will be half to those of △ A B C . Therefore, the ratio among the three sides will be the same in both triangles; both triangles are similar.