Try not to use L'Hôpital!

$\frac{1}{x}-\frac{1}{\sin(x)}=\frac{\sin(x)-x}{x\sin(x)}=\frac{x-\frac{x^3}{3!}+o(x^5)-x}{x^2-\frac{x^4}{3!}+o(x^6)}=\frac{-\frac{1}{3!}+o(x^2)}{\frac{1}{x}+o(x)}$

$\therefore \lim_{x\to0}\left(\frac1x - \frac1{\sin x}\right) = \lim_{x\to0}\left(\frac{-\frac{1}{3!}+o(x^2)}{\frac{1}{x}+o(x)}\right)=\lim_{x\to0}\frac{ -\frac{1}{3!}}{\frac{1}{x}}= 0$

There are two approaches that I know of. The first approach is using the Squeeze Theorem, and the second approach is using the L'Hôpital Rule.

---

Squeeze Theorem:

I will only show that $\displaystyle\lim_{x\to0^+} \left(\frac1x - \frac1{\sin x}\right)=0$ . The same method can be applied to show that $\displaystyle\lim_{x\to0^-} \left(\frac1x - \frac1{\sin x}\right)=0$ , while changing the signs.

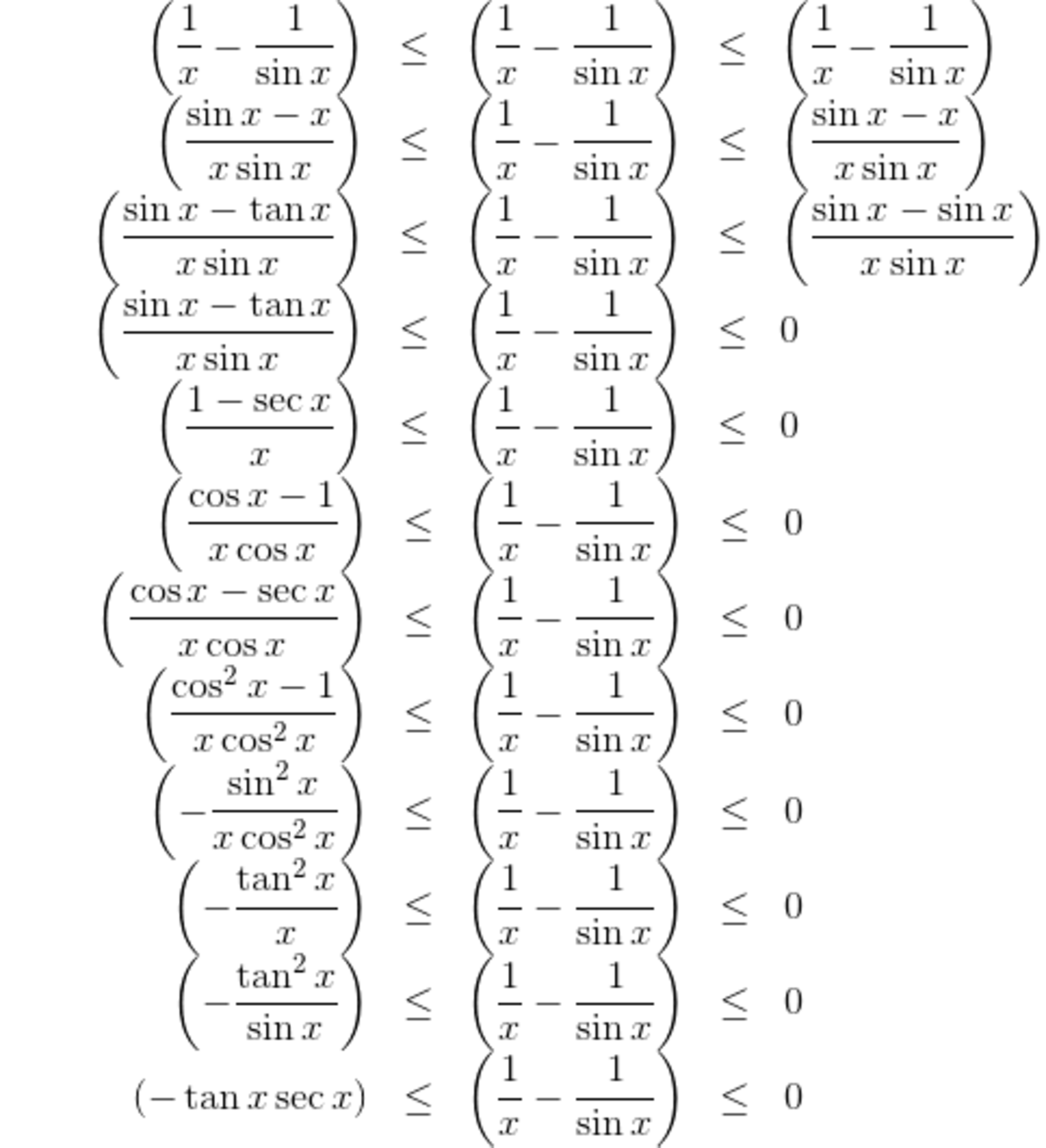
Substitute $x=0$ to the left hand side to obtain:

Therefore: $\lim_{x\to0^+}\left(\frac1x - \frac1{\sin x}\right) = 0$

---

L'Hôpital rule:

• Note that: $\left(\frac1x - \frac1{\sin x}\right) = \frac{\sin x-x}{x\sin x}$

• Since both the numerator and the denominator of the fraction is zero when $x=0$ , this rule can be used.

• Differentiate both the numerator and the denominator to obtain: $\frac{\cos x-1}{\sin x+x\cos x}$

• Since both the numerator and the denominator of the fraction is zero when $x=0$ , this rule can be used.

• Differentiate both the numerator and the denominator to obtain: $\frac{-\sin x}{\cos x+\cos x-x\sin x}$

• The denominator is not zero anymore when $x=0$ , so we can substitute $x=0$ : $\frac{-\sin0}{\cos0+\cos0-0\sin0}=0$

Therefore: $\lim_{x\to0}\left(\frac1x - \frac1{\sin x}\right) = 0$

---

Notes:

• In the squeeze theorem approach, I used these inequalities that are valid for $0<x<1$ : $\sin x<x<\tan x\\\cos x<1<\sec x$

• If instead, $0>x>-1$ , then: $\sin x>x>\tan x\\\cos x<1<\sec x$

• Note that the second inequality is unchanged.

Here's another approach:

Let $L=\displaystyle\lim_{x\to0} \left( \frac1x - \frac1{\sin x} \right)$ and let us assume that the limit exists.

Since the limit exists the left and right hand limits must both exist and equal to $L$ .

$\therefore L=\displaystyle\lim_{x\to0^{+}} \left( \frac1x - \frac1{\sin x} \right)..........(1)$

and

$\therefore L=\displaystyle\lim_{x\to0^{-}} \left( \frac1x - \frac1{\sin x} \right)..........(2)$

Replacing $x$ by $-x$ in $(2)$

$L=\displaystyle\lim_{x\to0^{+}} \left( -\frac1x + \frac1{\sin x} \right)..........(3)$

Adding $(1)$ and $(3)$

$2L=0$

$\therefore L=\boxed{0}$

$\mathbf{NOTE:}$ In order to use this method we must be sure that the limit exists and is finite.

Lim(x--0)[sin(x) - x]/xSin(x) = Lim[Sinx/xSinx - x/xSin] = Lim[Sinx/x 1/Sinx - 1/Sinx] = [ Lim Sinx/x * Lim (1/Sinx) - Lim (1/Sinx) ] = [ 1 Lim (1/Sinx - 1/Sinx) ] = 1* 0 = 0

I simply multiplied the whole term by x and as x tends to zero sinx/x =1 hence 1-1=0

Just for convenience to type, I leave out the lim operator.

[1/x - 1/sin(x)] = [sin(x) - x] / [x * sin(x)]

Using L'Hospital (remember there should be lim operator)

[sin(x) - x] / [x * sin(x)] = [cos(x) - 1] / [sin(x) + x * cos(x)] = [-sin(x) ] / [cos(x) + cos(x) - x * sin(x)]

by substituting x with 0

[-sin(0) / [cos(0) + cos(0) - 0 * sin(0)] = 0 / [1 + 1 - 0] = 0 / 2 = 0