Hemisphere Mechanics |20-09-2020|

Numerical solution this time. Let the coordinates of the hemisphere and disc be respectively:

$x_h = s$ $y_h = 0$ $x_d = s + R\sin{\theta}$ $y_d = R\cos{\theta}$

Let the reaction force radially outward acting on the disk be $N$ . Drawing a free body diagram and applying Newton's second law gives:

$m\ddot{x}_h = -N\sin{\theta}$ $m\ddot{x}_d = N\sin{\theta}$ $m\ddot{y}_d = N\cos{\theta}-mg$

Crunching out derivatives, plugging them above and re-writing the equations in a matrix form gives:

$\left[\begin{matrix} m &0&\sin{\theta} \\ m&mR\cos{\theta}&-\sin{\theta} \\ 0&-mR\sin{\theta}&-\cos{\theta} \end{matrix}\right]\left[\begin{matrix}\ddot{s}\\ \ddot{\theta}\\N\end{matrix}\right] = \left[\begin{matrix}0\\ mR\dot{\theta}^2\sin{\theta}\\mR\dot{\theta}^2\cos{\theta} -mg\end{matrix}\right]$

$\implies \left[\begin{matrix}\ddot{s}\\ \ddot{\theta}\\N\end{matrix}\right] = \left[\begin{matrix} m &0&\sin{\theta} \\ m&mR\cos{\theta}&-\sin{\theta} \\ 0&-mR\sin{\theta}&-\cos{\theta} \end{matrix}\right]^{-1} \left[\begin{matrix}0\\ mR\dot{\theta}^2\sin{\theta}\\mR\dot{\theta}^2\cos{\theta} -mg\end{matrix}\right]$

$s(0) = \dot{s}(0) = \theta(0) = 0$ $\dot{\theta}(0) = 10^{-4}$

Taking $m=R=1$ and $g=10$ the following simulation code is prepared:

 1
 2
 3
 4
 5
 6
 7
 8
 9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57

clear all
clc

% Initialisation:
R         = 1;
g         = 10;

% Initial states:
s(1)      = 0;
theta(1)  = 0;
dtheta(1) = 1e-4;
ds(1)     = 0;

% Time step and initialisation:
dt        = 1e-5;
t(1)      = 0;

% Initial reaction force:
Rf        = g;

% Index initialisation:
k         = 1;


% Loop running till contact is lost:
while Rf >= 0

    Th          = theta(k);
    dTh         = dtheta(k);

    % Writing out the A and b matrices:
    A           = [1 0 sin(Th);1 R*cos(Th) -sin(Th);0 -R*sin(Th) -cos(Th)];
    b           = [0;R*sin(Th)*dTh^2;R*cos(Th)*dTh^2-g];

    % Solving for dds, ddtheta and N:
    S           = inv(A)*b;
    dds         = S(1);
    ddtheta     = S(2);
    N(k)        = S(3);
    Rf          = N(k);

    % Numerical integration for velocities:
    ds(k+1)     = ds(k) + dds*dt;
    dtheta(k+1) = dtheta(k) + ddtheta*dt;

    % Numerical integration for position:
    s(k+1)      = s(k) + ds(k+1)*dt;
    theta(k+1)  = theta(k) + dtheta(k+1)*dt;

    % Time and index increment:
    t(k+1)      = t(k) + dt;  
    k           = k+1;
end

ANSWER = cos(theta(end))

%ANSWER = 0.732023082136735

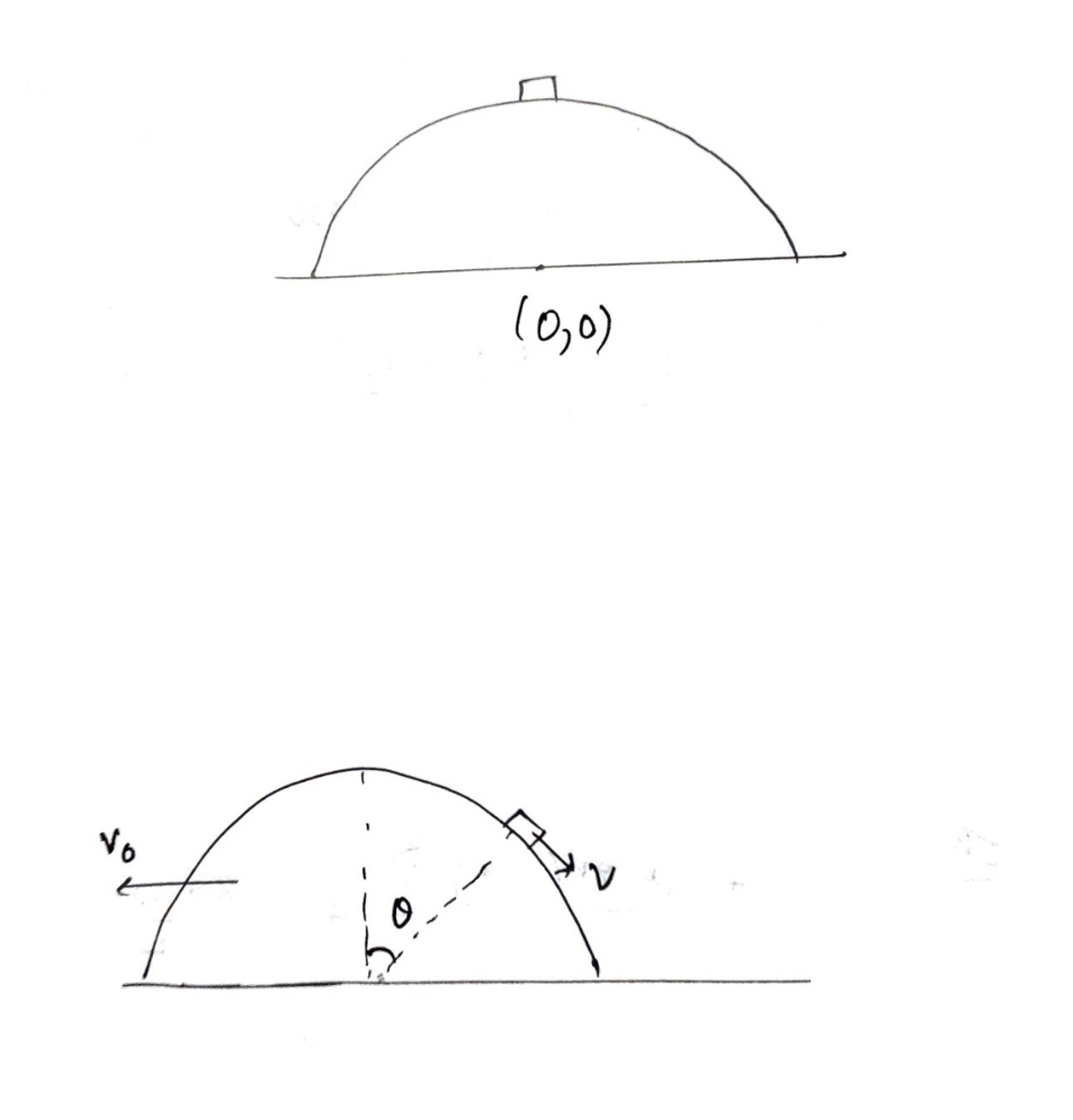
The velocity of that small disc $v$ is with respect to hemisphere frame .
And the velocity of that $v_{0}$ of that hemisphere is in ground frame.

So, $F_{x} =0$
So let's conserve momentum in $x$ direction $0=-mv_{0}+m(v \cos \theta -v_{0})$ After this conserving energy as always $mgR=\frac{1}{2}mv_{0}^{2}+\frac{1}{2}m(v_{0}^{2}+v^{2}+2vv_{0} \cos (π- \theta))+ mg(R-R \cos \theta)$
And the last equation normal to the inclined plane when it losses contact normal reaction will be zero $N=0$
Which gives the equation $\frac{mv^{2}}{R}=mg \cos \theta$
After solving these 3 equations patiently gives $\cos \theta= \sqrt{3}-1, 2,-1-\sqrt{3}$
The only reasonable value of $\cos \theta$ seems to be $\large \boxed{cos \theta=\sqrt{3}-1}$