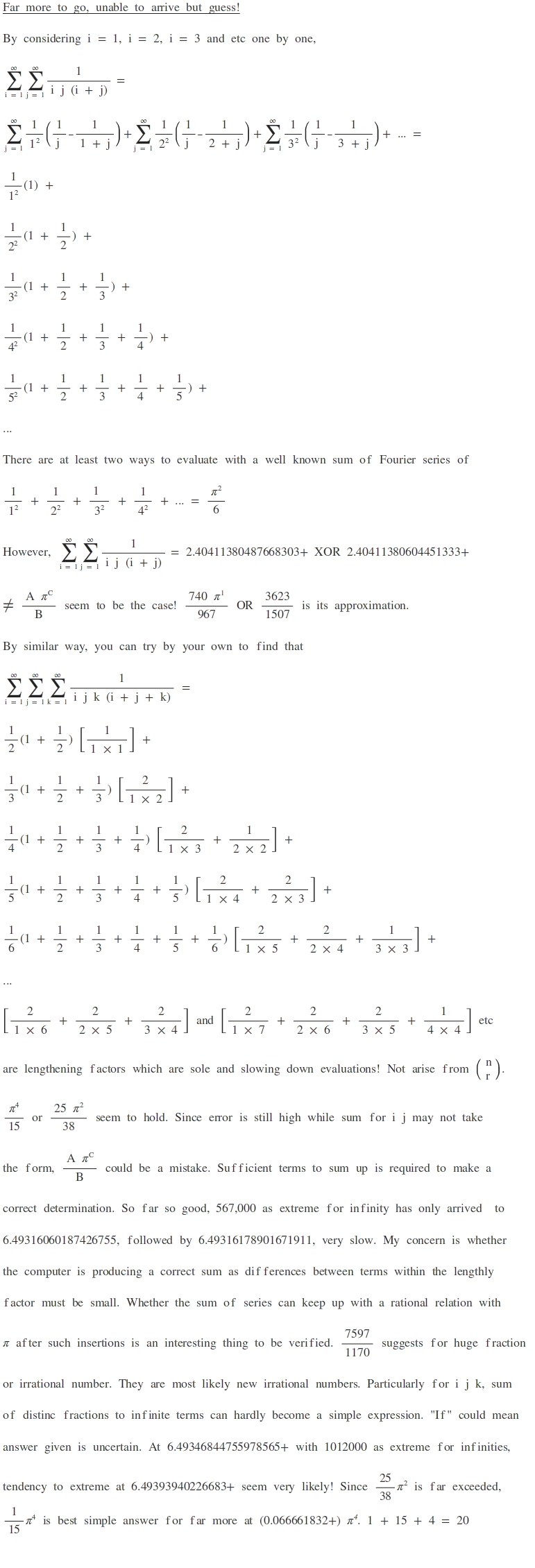
Highly Nested Summation!

We'll start generalizing our Bonus Problem to calculate $S$ by substituting $n=3$ .

We first notice that:

$\begin{aligned} \displaystyle P(n) &= \sum_{i_1=1}^\infty \cdots \sum_{i_n=1}^\infty \dfrac{1}{i_1i_2 \dotsm i_n} \int_0^1 x^{i_1 + i_2 + \ldots + i_n-1} dx \\ &= \int_0^1 \dfrac{1}{x} \left( \sum_{i_1=1}^\infty \dfrac{x^{i_1}}{i_1} \right) \cdots \left( \sum_{i_n=1}^\infty \dfrac{x^{i_n}}{i_n} \right) dx \\ &= \int_0^1 (-1)^n \dfrac{(\ln(1-x))^n}{x} dx \end{aligned}$

We now use the substitution $t=1-x$ in the above integral to get:

$\begin{aligned} \displaystyle P(n) &= (-1)^n \int_0^1 \dfrac{(\ln(t))^n}{1-t} dt = (-1)^n \int_0^1 (\ln(t))^n \left( \sum_{r=0}^\infty t^r \right) dt \\ &= (-1)^n \sum_{r=0}^\infty \int_0^1 t^r (\ln(t))^n dt \end{aligned}$

In view of the integration by parts formulaes, we see that:

$\int_0^1 t^r (\ln(t))^n dt = (-1)^n \dfrac{n!}{(r+1)^{n+1}}$

Therefore:

$P(n) = (-1)^n \sum_{r=0}^\infty (-1)^n \dfrac{n!}{(r+1)^{n+1}} = n! \sum_{r=0}^\infty \dfrac{1}{(r+1)^{n+1}}$

$\large \Longrightarrow \boxed{P(n) = n! \cdot \zeta(n+1)}$

where $\zeta(s)$ denotes the Riemann-Zeta Function. To calculate the value of $S$ , substitute $n=3$ to obtain:

$\large S = 3! \cdot \zeta(4) = 6 \cdot \dfrac{\pi^4}{90} = \dfrac{\pi^4}{15}$

So $A=1, \ B=15, \ C=4, \quad A+B+C=\boxed{20}$