Infinite Product
( 1 − 2 2 1 ) ( 1 − 3 2 1 ) ( 1 − 4 2 1 ) ( 1 − 5 2 1 ) ⋯ = ?
The answer is 0.5.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
5 solutions
Moderator note:
Wonderful work! That's one way to incorporate factorials into your solution.
1 - (1/n^2) =( n^2 - 1)/n^2 n^2 - 1 = (n+1) (n-1) Therefore 1- (1/n^2) = (n+1/n) (n-1/n) Solve partial products separately then multiply, you get: Lim R--->infinity R+1/2 And 1/R Multiplying we get (R+1/R) × (1/2)= 1/2
Moderator note:
This is the neatest solution. Wonderful work!
Bonus question : Evaluate the product k = 2 ∏ ∞ k 2 4 k 2 − 1 .
In your second step (where you wrote out all the terms of the products), there appears to be a minor typo.
The second term in the second product should be 3 4 and not 5 4 .
@Calvin Lin , if my understanding of products is correct, then the one in the Challenge Master note cannot be "evaluated" since it diverges. This can be verified by noting that 4 k 2 − 1 > k 2 ∀ k ∈ Z ≥ 2
Log in to reply
While the product cannot be evaluated (even though it could telescope product cancel), you do not provide a sufficient reasoning.
For example, ∏ k 2 k 2 + 1 does converge, even though k 2 + 1 > k 2 .
Log in to reply
Fair enough. I guess a better reasoning would be to use the standard method of testing the related series ∑ k = 2 ∞ lo g ( k 2 4 k 2 − 1 ) which diverges thus implying that the related product diverges too. Right?
Moderator note:
Yes, this is telescoping product approach. Nice standard approach.
We see that each term can be factored with difference of squares: (1- 2 1 )(1+ 2 1 )(1- 3 1 )(1+ 3 1 ) ...
Evaluating each term results in: ( 2 1 )( 2 3 )( 3 2 )( 3 4 )( 4 3 )...
Aside from the first term, all other terms cancel out, leaving us with 2 1
perfect and smart solution
The expression:
k = 2 ∏ ∞ k 2 k 2 − 1 = k = 2 ∏ ∞ k 2 ( k − 1 ) ( k + 1 ) = 2 ˙ 2 1 ˙ 3 ˙ 3 ˙ 3 2 ˙ 4 ˙ 4 ˙ 4 3 ˙ 5 ˙ 5 ˙ 5 4 ˙ 6 ˙ . . . = 2 ˙ 2 1 ˙ 3 ˙ 3 ˙ 3 2 ˙ 4 ˙ 4 ˙ 4 3 ˙ 5 ˙ 5 ˙ 5 4 ˙ 6 ˙ . . . = 2 1
Moderator note:
Where did the 6 cancel out with? Even you have shown more initial terms, there will always be a number in the numerator of the last fraction that you can't cancel out with.
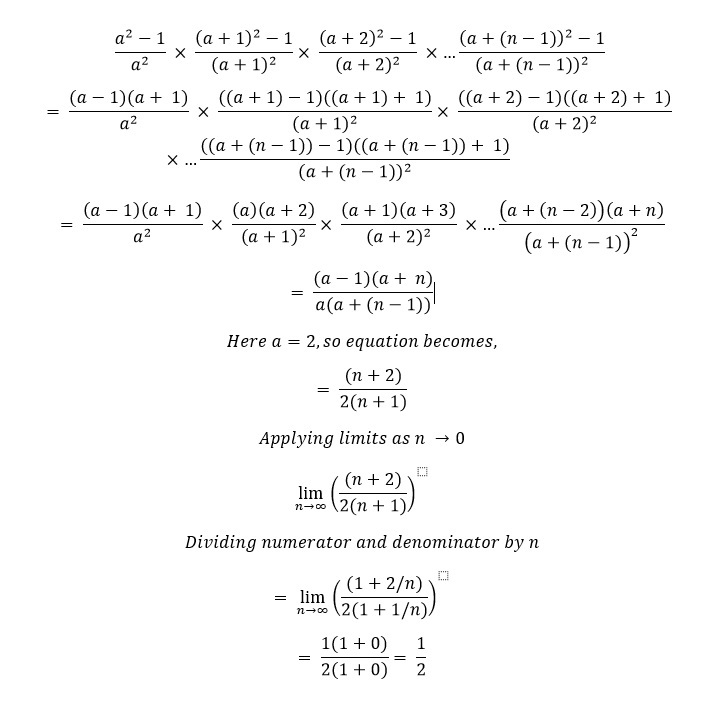
Let us define the general term for this product.
T n = ( n + 1 ) 2 ( n + 1 ) 2 − 1 = ( n + 1 ) 2 n ( n + 2 )
Now, Product to n terms,
P n = 2 2 × 3 2 × . . . × ( n + 1 ) 2 ( 1 × 2 × . . . × n ) × ( 3 × 4 × . . . × ( n + 2 ) )
or,
P n = 2 ( ( n + 1 ) ! ) 2 n ! ( n + 2 ) ! = 2 × ( n + 1 ) ! n ! × ( n + 1 ) ! ( n + 2 ) ! = 2 1 × n + 1 1 × ( n + 2 )
Thus,
P n = 2 n + 2 n + 2
So, product of infinite terms, P = n → ∞ lim P n = n → ∞ lim 2 n + 2 n + 2
Dividing both numerator and denominator by n = 0 , we get P = n → ∞ lim 2 + n 2 1 + n 2 = 2 1 = 0 . 5