Integrating Various Functions
∫ 0 ∞ x e − x sin 2 x d x = ?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Alternatively, we can also use differentiation under integration by considering the function F ( t ) = ∫ 0 ∞ x e − t x sin x d x
Log in to reply
That's unusual. You would get F ′ ( t ) = t ( t 2 + 2 2 ) 2 . How are you suppose to find its antiderivative then? Keep in mind that F ′ ( t ) is undefined when t = 0 .
Log in to reply
Typo i meant sinx.
Log in to reply
@Harsh Shrivastava – Well i have not tried my method but think that it can work.
Log in to reply
@Harsh Shrivastava – Do give it a try. We want to find the value of F ( 1 ) right? So, we need to find F ( t ) first, which is an antiderivative of F ′ ( t ) . Try integrating the function F ′ ( t ) = t ( t 2 + 2 2 ) 2 from the range of 0 to t and see what happens.
Log in to reply
@Pi Han Goh – Oh wait. Ignore what I said. I've found a way to avoid this error. @Harsh Shrivastava you're right. Would you like to post the solution?
Log in to reply
@Pi Han Goh – Bit (buzy+dead) at the moment.
You may post.
Log in to reply
@Harsh Shrivastava – Slack is still waiting for you.....
What are those examples of Maclaurin series. Can you spare me?
Log in to reply
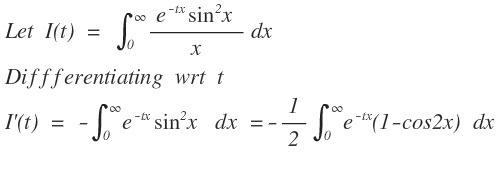 now we know that :-
now we know that :-
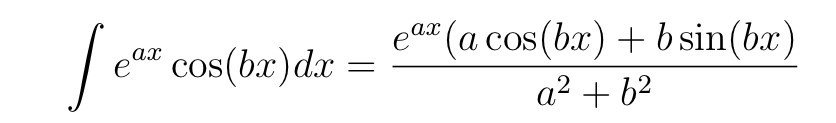 So
So
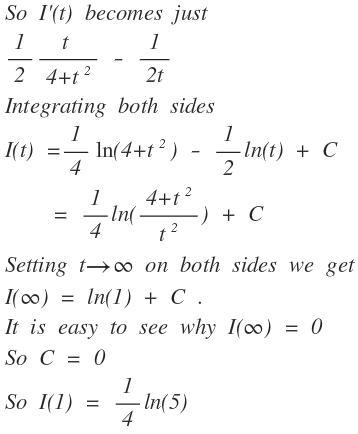
In line 2, its Differentiating and NOT d i f f f e r e n t i a t i n g
Log in to reply
Go fff**k yourself.
Log in to reply
I am only kidding....hope you did not mind
Let the integral be I . Then we have:
I = ∫ 0 ∞ x e − x sin 2 x d x = ∫ 0 ∞ 2 x e − x ( 1 − cos 2 x ) d x By Maclaurin series: = ∫ 0 ∞ 2 x e − x ( 1 − n = 0 ∑ ∞ ( 2 n ) ! ( − 1 ) n ( 2 x ) 2 n ) d x = ∫ 0 ∞ 2 x e − x ( n = 1 ∑ ∞ ( 2 n ) ! ( − 1 ) n + 1 ( 2 x ) 2 n ) d x = ∫ 0 ∞ n = 1 ∑ ∞ ( 2 n ) ! ( − 1 ) n + 1 ( 2 x ) 2 n − 1 e − x d x = n = 1 ∑ ∞ ( 2 n ) ! ( − 1 ) n + 1 ∫ 0 ∞ ( 2 x ) 2 n − 1 e − x d x = n = 1 ∑ ∞ ( 2 n ) ! ( − 1 ) n + 1 2 2 n − 1 Γ ( 2 n ) = n = 1 ∑ ∞ ( 2 n ) ! ( − 1 ) n + 1 2 2 n − 1 ( 2 n − 1 ) ! = n = 1 ∑ ∞ 2 n ( − 1 ) n + 1 2 2 n − 1 = 4 1 n = 1 ∑ ∞ n ( − 1 ) n + 1 4 n = 4 ln ( 1 + 4 ) = 4 ln 5