Integration Grandmaster - P14
Let
A = ∫ a b ( x − a ) 4 + ( x − b ) 4 1 d x
Submit ⌊ 1 0 0 0 0 A ⌋ for a = 1 and b = 2 .
The answer is 37922.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
A = ∫ 1 2 ( x − 1 ) 4 + ( x − 2 ) 2 d x = ∫ − 2 1 2 1 ( u + 2 1 ) 4 + ( u − 2 1 ) 4 d u = 2 ∫ 0 2 1 ( u + 2 1 ) 4 + ( u − 2 1 ) 4 d u = 2 ∫ 0 2 1 2 u 4 + 3 u 2 + 8 1 d u = ∫ 0 2 1 u 4 + 2 3 u 2 + 1 6 1 d u = ∫ 0 2 1 ( u 2 + ( 2 2 − 1 ) 2 ) ( u 2 + ( 2 2 + 1 ) 2 ) d u = ∫ 0 2 1 2 1 ( u 2 + ( 2 2 − 1 ) 2 1 − u 2 + ( 2 2 + 1 ) 2 1 ) d u = 2 1 [ 2 − 1 2 tan − 1 2 − 1 2 u − 2 + 1 2 tan − 1 2 + 1 2 u ] 0 2 1 = ( 2 + 2 ) tan − 1 ( 2 + 1 ) − ( 2 − 2 ) tan − 1 ( 2 − 1 ) = 2 tan − 1 1 + 1 2 + 1 − 2 + 1 + 2 tan − 1 1 − 1 2 + ‘ 1 + 2 − 1 = 2 π ( 1 + 2 ) ≈ 3 . 7 9 2 2 3 7 7 9 6 Let u = x − 2 3 ⟹ d u = d x Since the integrand is even
Therefore ⌊ 1 0 0 0 0 A ⌋ = 3 7 9 2 2 .
Note: I've updated my solution to reflect Mark Hennings' feedback below. Previously, I mentioned that when a = b , the integral is trivially 0. This is a false assertion because the integrand not a proper integral, so the property ∫ 0 0 f ( z ) d z = 0 does not hold.
If a = b , then the integral becomes ∫ a a 2 1 ( x − a ) 4 1 d y = y = x − a 2 1 ∫ 0 0 y 4 1 d y . But this is (clearly) not a proper integral as it is undefined when y = 0 , and it diverges. Hence, the integral in question is only finite when a = b .
So I will only consider the case where a = b . Let us transform the integrand to make it an even function. That is, let y = x − 2 a + b , A = ∫ ( a − b ) / 2 ( b − a ) / 2 ( y + 2 b − a ) 4 + ( y + 2 a − b ) 4 1 d y Let c = 2 b − a . The integral simplifies to A = ∫ − c c ( y − c ) 4 + ( y + c ) 4 1 d y = 2 ∫ 0 c ( y − c ) 4 + ( y + c ) 4 1 d y because the integrand is an even function. Let's try to simplify the integrand even further, A 0 0 0 0 = = = = = ∫ 0 c y 4 + 6 c 2 y 2 + c 4 1 d y ∫ 0 c ( y 2 + 3 c 2 ) 2 − 8 c 4 1 d y ∫ 0 c y 2 + [ c ( 2 + 1 ) ] 2 1 ⋅ y 2 + [ c ( 2 − 1 ) ] 2 1 d y 4 2 c 2 1 [ − c ( 2 + 1 ) 1 tan − 1 ( c ( 2 + 1 ) y ) + c ( 2 − 1 ) 1 tan − 1 ( c ( 2 − 1 ) y ) ] y = 0 y = c 4 2 c 3 1 [ − 2 + 1 1 tan − 1 ( 2 + 1 1 ) + 2 − 1 1 tan − 1 ( 2 − 1 1 ) ] Apply partial fractions Rationalize the denominators of each of the fractions above gives 2 ± 1 1 = 2 ∓ 1 ⟹ tan − 1 ( 2 ± 1 1 ) = 4 π ± 8 π We can further simplify A to A = 4 2 c 3 1 ⋅ 4 ( 2 + 2 ) π = 2 π ⋅ ( b − a ) 3 1 + 2 The answer is ⌊ 1 0 4 ⋅ 2 π ( 2 + 1 ) ⌋ = 3 7 9 2 2 .
Indeed, if we take the limit as b → a + , the integral does diverge to infinity, b → a + lim A = b → a + lim 2 π ⋅ ( b − a ) 3 1 + 2 → ∞ .
“When a = b , then the integral is trivially zero.” This is not true, and certainly not trivially. “When a = b , the integral is undefined” is correct. Note that the integration range tends to 0 as b → a , but the integrand diverges to ∞ . Indeed, as b → a , the integral diverges to ∞ .
Log in to reply
I've been staring at your comment for over 2 hours now. I must be missing something obvious here...
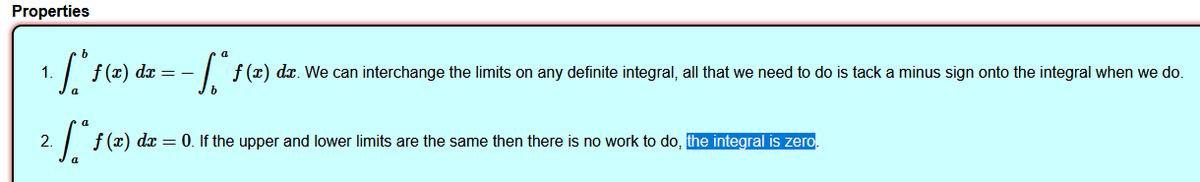
(I) : I agree that b → 0 + lim ∫ 0 b x 1 d x → ∞ .
But I believed the following 2 points are true:
(II) : b → a + lim ∫ a b x 1 d x = 0 for a = 0 .
(III) : ∫ 0 0 x 1 d x = 0 .
(IV) : So for the original integral, if we let a = b , then it becomes A = ∫ a a ( x − a ) 4 2 d x = ∫ 0 0 y 4 2 d y = 0
"based on my corollaries above".
So either my corollaries are not all correct, and that I need to punish myself, or we're using different semantics/definitions here.
(V) : To clarify, for a < b , my interpretation of ∫ a b f ( x ) d x is the difference between the areas found for ( y > 0 , a < x < b ) and ( y < 0 , a < x < b ) .
(VI) : For example, ∫ 0 3 π / 2 sin x d x = 2 − 1 = 1 because the area of region above and below the x -axis for 0 < x < 2 3 π are 2 and 1, respectively.
(VII) : On the other hand, we can both agree that ∫ 0 1 x d x = 3 2 .
(VIII) : And, since the integrand x is only defined for x ⩾ 0 , then there is no "region formed" for x < 0 . Thus, ∫ − 1 0 x d x = 0 , Or more generally, ∫ p q x d x = 0 for all negative numbers p and q ....
(IX) : But, this disagrees with WolframAlpha's output . I guessed WolframAlpha doesn't strictly restrict the integrand to a real value output.
If you could pinpoint and rectify my fallacy (plural?) above, please let me know.
By the way, love your solution, I don't know how you managed to motivate that substitution x = a cos 2 θ + b sin 2 θ , but it's simply elegant.
Log in to reply
The text you are quoting from is assuming that f is well-defined. If the book is about Riemann integration, it is assuming that f is bounded. Basically, there is nothing to say about trying to integrate an infinite function on an interval of zero width - it is undefined.
That said, the physicists are quite happy to have a "function" that is infinite over an interval of zero width, and zero elsewhere, but has integral 1 - consider the Dirac delta "function". As a mathematician, I regard the Dirac delta function more precisely as a distribution: the linear map from continuous functions on R to R that sends the function f to f ( 0 ) .
The physicists like it as a "function".
- It makes notation simpler, if the maths dodgier.
- If is the "limit" as n → ∞ of the function that is equal to 2 n for − n 1 < x < n 1 , and zero elsewhere. Thus it is a good idealisation of a quantity that is extremely large over a very small range, but nonetheless has measurable effect - the electrostatic potential of an atom in a lattice, for example.
- Many equations using it can be solved exactly, which could not for a more realistic function
Log in to reply
@Mark Hennings – Wow, I'm not astute at all, because I failed to notice that the link I've shared above only applies to DEFINITE integrals. This is a monumental failure on my part.
I've rectified my solution.
Thank you for your swift and detailed response as usual. I didn't realize that the Dirac delta "function" is also related to this conversation (indirectly).
Also, thank you for your response to the clever substitution x = a cos 2 θ + b sin 2 θ . You're literally a godsend.
Log in to reply
@Pi Han Goh – Replace "definite" with "proper", and it will be a better correction.
@Mark Hennings – On a related note, I finally discovered why I failed to solve the limit below.
Question: x → 0 lim x 0 ∫ sin x sin ( t 1 ) cos ( t 2 ) d t = 0
My wrong attempt: Set x = 0 and realize that the numerator is in the form ∫ 0 0 , which I wrongly concluded that it must equal to 0, so I then used Lhopital rule followed by Fundamental theorem of calculus part 1, which lead me to wrong answer of "limit does not exist."
Once again, you're truly a sagacious teacher.
The solution is here (second page) . If you can't view the solution for whatever reason, press this link .
You might like this Instagram page though.... but they don't have the same type of interaction as Brilliant.
The substitution x = a cos 2 θ + b sin 2 θ is a pretty standard one for these problems. It is the composition of the substitutions y = x − a and y = ( b − a ) sin 2 θ . The first of these makes the integral a function of b − a , and the second isolates b − a from the integration.

The substitution x = a cos 2 θ + b sin 2 θ gives us A ( a , b ) = ( b − a ) 3 1 ∫ 0 2 1 π sin 8 θ + cos 8 θ sin 2 θ d θ = ( b − a ) 3 1 6 ∫ 0 2 1 π ( cos 2 θ − 1 ) 4 + ( cos 2 θ + 1 ) 4 sin 2 θ d θ = ( b − a ) 3 8 ∫ 0 2 1 π cos 4 2 θ + 6 cos 2 2 θ + 1 sin 2 θ d θ = ( b − a ) 3 4 ∫ − 1 1 u 4 + 6 u 2 + 1 d u = 2 ( b − a ) 3 1 ∫ − 1 1 ( u 2 + 3 − 8 1 − u 2 + 3 + 8 1 ) d u = 2 ( b − a ) 3 1 ∫ − 1 1 ( u 2 + ( 2 − 1 ) 2 1 − u 2 + ( 2 + 1 ) 2 1 ) d u = 2 ( b − a ) 3 1 [ 2 − 1 1 tan − 1 ( 2 − 1 u ) − 2 + 1 1 tan − 1 ( 2 + 1 u ) ] − 1 1 = ( b − a ) 3 2 [ ( 2 + 1 ) tan − 1 ( 2 + 1 ) − ( 2 − 1 ) tan − 1 ( 2 − 1 ) ] = ( b − a ) 3 2 [ 8 3 π ( 2 + 1 ) − 8 1 π ( 2 − 1 ) ] = 2 ( b − a ) 3 π ( 2 + 1 ) We want A ( 1 , 2 ) = 2 1 π ( 2 + 1 ) and ⌊ 1 0 0 0 0 A ( 1 , 2 ) ⌋ = 3 7 9 2 2 .