Its just a collision
A ball of mass falls vertically from a height and collides with a block of equal mass moving horizontally with a velocity on a horizontal surface. The coefficient of kinetic friction is while the coefficient of restitution between the block and ball is . There is no friction acting between the block and the ball. The velocity of the block just after the collision decreases by
Find
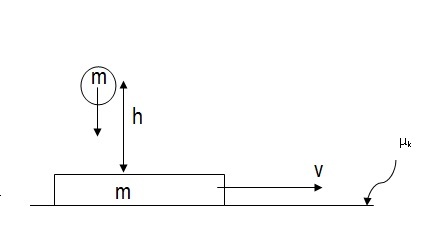
Details and Assumptions
Take acceleration due to gravity as
The block doesn't bounces off the ground after collision.
Try my set
The answer is 8.7.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.

I don't know whether my solution is correct or not, feel free to report any flaw.
I will place the values later in the answer.
velocity of ball after collision is e 2 g h
Impulsive force from normal causes change in momentum of the ball.
J N = N . d t = f i n a l − i n i t i a l m o m e n t u m .
As after collision velocity of ball is in opposite direction so
N . d t = m e 2 g h − ( − m 2 g h )
( after replacing value of e )
N . d t = 1 . 5 m 2 g h ................................... ( 1 )
The impulsive frictional force (denoted as f ) will cause change in momentum of block.
J f = f . d t = − m Δ v
(minus sign because velocity decreases)
Using
f = μ k N
μ k N . d t = − m Δ v
From ( 1 )
μ k × 1 . 5 m 2 g h = − m Δ v
putting values
Δ v = − 0 . 2 × 1 . 5 2 0 × 4 2 . 0 5
∣ Δ v ∣ = ∣ K ∣ = 8 . 7