Just Think
Evaluate:
( 3 . 5 ) 2 + 5 × 6 . 5 + ( 4 . 5 ) 2 ( 4 . 5 ) 2 − 5 × 6 . 5 + ( 3 . 5 ) 2
The answer is 0.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
9 solutions
how do you get 1? can't process it.
Log in to reply
oh.. tnx! got it anyway!! nice move sir! thanks!
Log in to reply
I am not sir if you want to know who are(sir's)-
@Sandeep Bhardwaj @DEEPANSHU GUPTA @Agnishom Chattopadhyay @Krishna Ar @Krishna Sharma @Satvik Golechha @Piyushkumar Palan @Sanjeet Raria and many others
Log in to reply
@U Z – I am a moderator. What does Sir mean?
Log in to reply
@Agnishom Chattopadhyay – A guru or a teacher
Log in to reply
@U Z – it doesn't always mean teacher, at times it is used to show respect
who it can solve this problem on less than 10 seconds without calculator....¡me!
Log in to reply
.....that was not on rsponse of Garrick
Nice solution, @megh choksi
Log in to reply
What was your method? @Anuj Shikarkhane
great method Megh
Numerator=(0.9x5)^2-(5x1.3x5)+(0.7x5)^2 =25(0.81-1.3+0.49) = 0
4.5 4.5-5 6.5+3.5*3.5 =20.25-32.5+12.25 =0
@Anuj Shikarkhane I just copied the title of your problem because I thought it was suitable for this problem
Try it and thank you
General formula:
(A - B) ^2 = A^2 - 2AB + B^2 So we have:
5 X 6.5 = 32.5
4.5 X 3.5 X 2 = 31.5
Need 1 less for the (5 X 6.5), so we can take 1 out from (5 X 36.5)
Now we have:
(((4.5)^2 - 31.5 + (3.5)^2 - 1)/ Original denominator
= ((4.5 -3.5)^2 -1)/ Original denominator
= ((1)^2 - 1)/ Original denominator
=0
Take the numerator -
First note that:
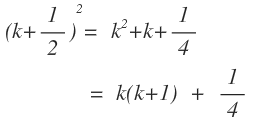So from this
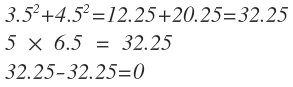
Since the numerator = 0, we have the whole fraction equalling 0.
I think you've made a small arithmetic error. In the second paragraph both answers should be 32.5, not 32.25.
BDMAS rule.................
1.multiple 5*6.5=32.5
2.find (4.5)^2 and (3.5)^2 which are 20.25 and 12.25 respectively
3.Solve numerator first i) numerator=0
Therefore ans is 0
x= 3.5, = ( X + 1 ) 2 − 5 ( x + 3 ) + x 2 / n o n e e d f o r m a t h = x 2 + 2 x + 1 − 5 x − 1 5 + x 2 = 2 x 2 − 3 x − 1 4 = ( x+2)(2x-7) = ( 3.5 +2) ( 7 -7) = 0= since numerator is zero answer is 0
Add and subtract 1 the numerator 4.5^{2} - 5 x 6.5 +3.5^{2}
then it becomes (4.5-3.5)^{2} -1 =1-1 =0
decimals not accepted is a loophole numerator is less than denominator(so answer can be 0 or 1) also 5^2+4^2-5*6=1 while denominator equals 61 so the obvious answer is 0
Numerator: (4.5)^2 - 5 X 6.5 + (3.5)^2 = 20.25 - 32.5 + 12.25 = 32.5 - 32.5 = 0
Since numerator = 0, fraction = 0.
={(4.5-3.5)^2-1}/{(4.5+3.5)^2+1} =0
Multiple the expression by 4 4 to eliminate the non-integer terms. ( 3 . 5 ) 2 + 5 ( 6 . 5 ) + ( 4 . 5 ) 2 ( 4 . 5 ) 2 − 5 ( 6 . 5 ) + ( 3 . 5 ) 2 ∗ 4 4 Distribute the 4's as 2's inside the squares and distribute between the two middle factors. = ( 3 . 5 ∗ 2 ) 2 + 5 ∗ 2 ( 6 . 5 ∗ 2 ) + ( 3 . 5 ∗ 2 ) 2 ( 4 . 5 ∗ 2 ) 2 − 5 ∗ 2 ( 6 . 5 ∗ 2 ) + ( 3 . 5 ∗ 2 ) 2 = ( 7 ) 2 + 1 0 ( 1 3 ) + ( 7 ) 2 ( 9 ) 2 − 1 0 ( 1 3 ) + ( 7 ) 2 Expand the squares and multiply out. = 4 9 + 1 3 0 + 8 1 8 1 − 1 3 0 + 4 9 = 2 6 0 0 = 0
Let a = 4 . 5
Therefore,
( a − 1 ) 2 + ( a + 0 . 5 ) ( a + 2 ) + ( a 2 ) ( a 2 ) − ( a + 0 . 5 ) ( a + 2 ) + ( a − 1 ) 2
Simplifying we get:
( a 2 − 2 a + 1 ) + ( a 2 + 2 . 5 a + 1 ) + ( a 2 ) a 2 − ( a 2 + 2 . 5 a + 1 ) + ( a 2 − 2 a + 1 )
The numerator can be simplified to:
( a 2 − 2 a + 1 ) + ( a 2 + 2 . 5 a + 1 ) + ( a 2 ) − 4 . 5 a + a 2
And since a = 4 . 5
Therefore: ( a 2 − 2 a + 1 ) + ( a 2 + 2 . 5 a + 1 ) + ( a 2 ) − a 2 + a 2 ( a 2 − 2 a + 1 ) + ( a 2 + 2 . 5 a + 1 ) + ( a 2 ) 0
Therefore, the expression = 0
1 − ( 3 . 5 ) 2 + 5 × 6 . 5 + ( 4 . 5 ) 2 2 × 5 × 6 . 5
= 1 − 5 × 6 . 5 3 . 5 × 3 . 5 + 1 + 5 × 6 . 5 4 . 5 × 4 . 5 2
= 1 − 1 . 3 0 . 4 9 + 1 . 3 0 . 8 1 + 1 2
= 1 − 1 + 1 2
= 0 . □