This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
Update : The concern raised with this comment has been dealt with.
I don't think the question ever mentioned that the squares go till infinity. There are only 4 squares each (parallel and slanted) in the figure given.
However, your answer is luckily correct as the number of both types of squares were same in this problem.
The correct solution to this problem would be:
By geometry, we see that the sides of the parallel and slanted squares are in GP with common ratio 2 1 . Also, the side length of the biggest parallel square is a while that of the biggest slanted square is 2 a . Thus for n squares each of the two types, we have
A 2 A 1 = a 2 ( 2 1 + 8 1 + 3 2 1 + ⋯ n terms ) a 2 ( 1 + 4 1 + 1 6 1 + ⋯ n terms ) = 2 for every positive integer n
n = 4 or n = ∞ (sum of infinite GP) is just a trivial case here so as long as the number of both types are equal. (Yes, I know that infinity is not a number. Maybe my argument of comparison is wrong. But I just wanted to make you clear that the problem never mentioned about an infinite number of squares.)
In gp the infinite one and the finite one's ratio is preserved as it is multiple privative....so not completely a matter of luck as the number of squares is the same
Log in to reply
Provided that number of slanted squares is however, not equal to parallel ones, you would have had a very different answer from the actual one.
Log in to reply
There are 3 dots at the centre which ensure infinity
Sorry its "multiplicative"
That's right absolutely
There are three dots at the centre....which ensure infinity
Log in to reply
Gosh! I think I need to check my eyesight for the good. Sorry then, your solution is correct.
Log in to reply
Your solution is a more rigorous one and is more appreciated....
Log in to reply
@Niladri Dan – Your solution also gives the idea of the problem too. Do you know how to format your solutions with L a T e X ?
Log in to reply
@Tapas Mazumdar – No I do not know so
Log in to reply
@Niladri Dan – You should see this note . It would be helpful if you want to learn L a T e X .
It holds for any condition
By the way I saw the 3 dots a little ago...😁😁😀😀😀😜😜😜
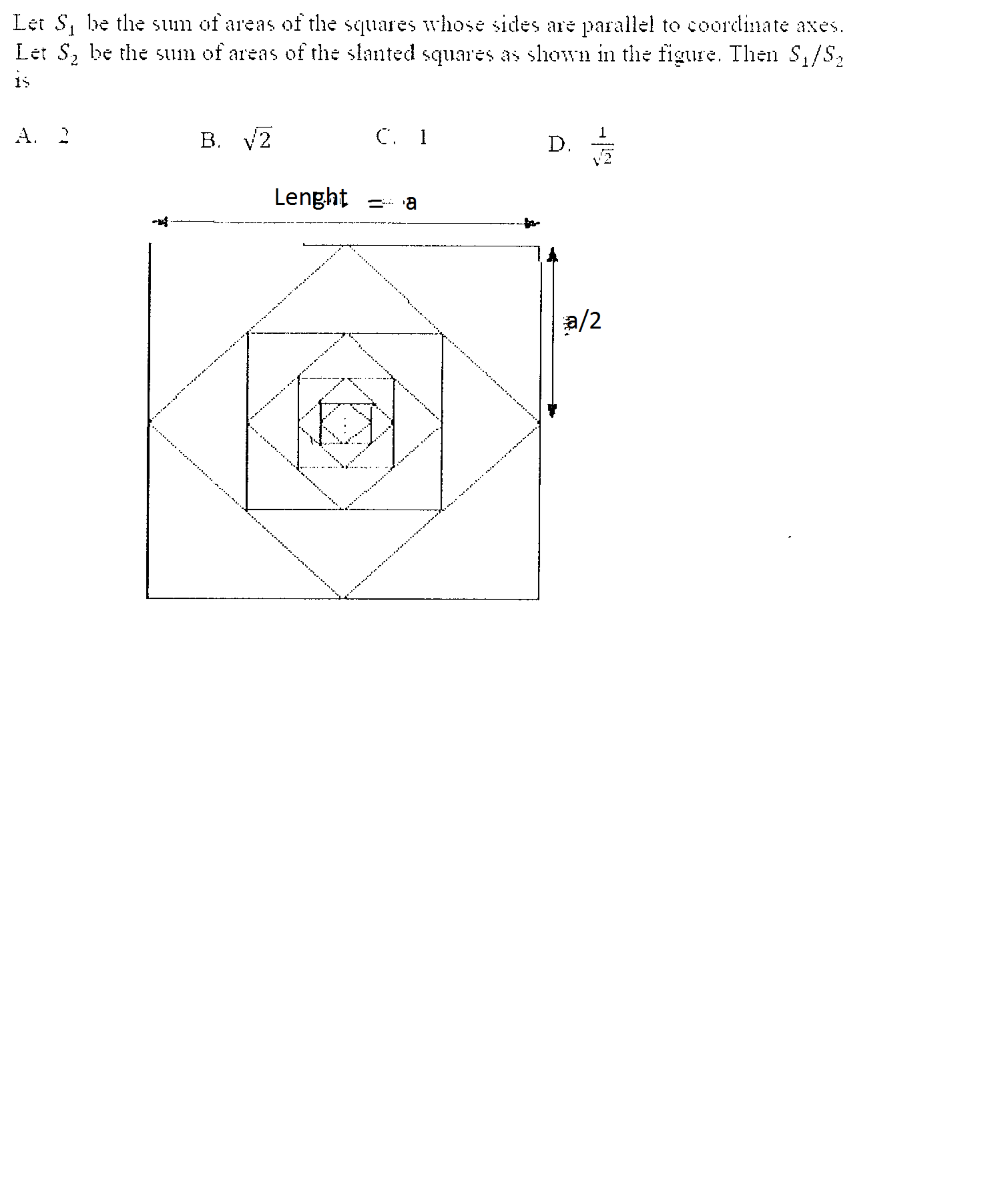
The sum will be an infinite gp with the first term varying... Sum of inf. gp= a/(1-r) such that r<1 here r will be same by rotation symmetry....What will vary is a. a1=a (say) therefore a2=(((a1)^(0.5))*√2 /2)^2=a1/2 Therefore S1/S2=a1/a2=2