This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
good one sir @Chew-Seong Cheong
But how did you find voltage across R as u already subtracted the voltage then why did you again did it Would u please clarify Mr. @Chew-Seong Cheong
Log in to reply
After deducting the 2V source, it is replaced by a short circuit. The equivalent circuit has only a 4V source at the place of the 6V source. The voltage across R of 1.5V means that on the equivalent circuit.
Log in to reply
Oh thanks sir And would you please tell me this problem W h a t i s t h e l a r g e s t i n t e g e r v a l u e o f n l e s s t h a n 1 0 0 0 s u c h t h a t n = b a − 1 b 2 + a 2 N o t e : a , b a r e n o n n e g a t i v e
please tell me the answer and solution sir @Chew-Seong Cheong
Log in to reply
@Mehul Chaturvedi – I didn't solve this problem.
Log in to reply
@Chew-Seong Cheong – why its easy
Log in to reply
@Mehul Chaturvedi – I didn't think I could solve it and I didn't want to lose points. The question has been posted by Calvin Lin. You shouldn't post it again.
Log in to reply
@Chew-Seong Cheong – Yeas i've deleted it sir and got the answer as 5
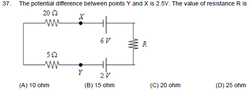
Since the 6 V and 2 V voltage sources are in opposite directions, the resultant equivalent voltage source is 6 V − 2 V = 4 V . Since V X Y = 2 . 5 V Then the voltage across R , V R = 4 V − 2 . 5 V = 1 . 5 V . Then we have:
2 0 + 5 R = 2 . 5 1 . 5 ⇒ R = 5 3 × 2 5 = 1 5 Ω