Losing contact
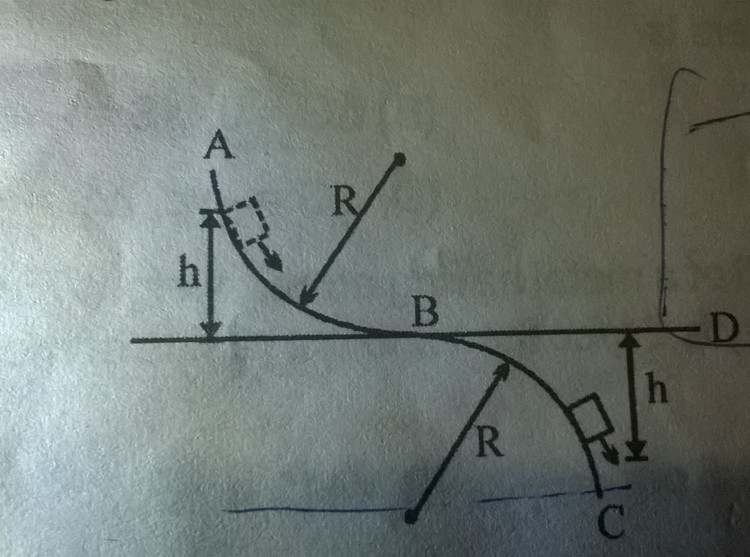 The figure above shows a smooth track in a vertical plane, consisting of two circular arcs
and
of same radius
The common tangent to the arcs,
is horizontal. A small block placed on the track at a height
(in centimeters) above
leaves the track at exactly the same depth below. Find
The figure above shows a smooth track in a vertical plane, consisting of two circular arcs
and
of same radius
The common tangent to the arcs,
is horizontal. A small block placed on the track at a height
(in centimeters) above
leaves the track at exactly the same depth below. Find
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Sorry, have taken r in place of R.