Not Just Geometry! It's Physics!

Classical Mechanics Level 2

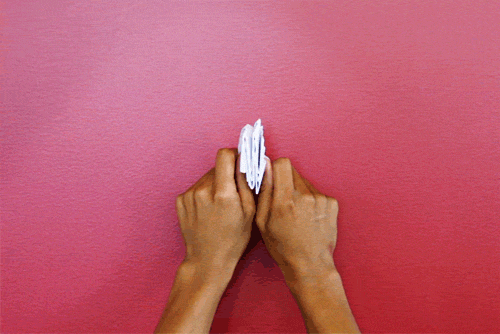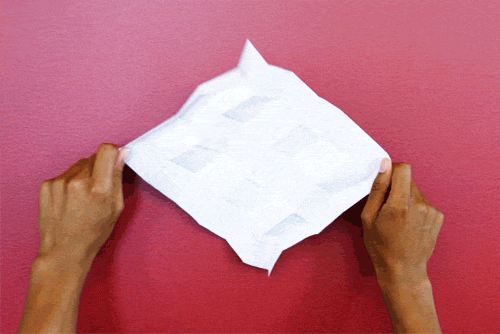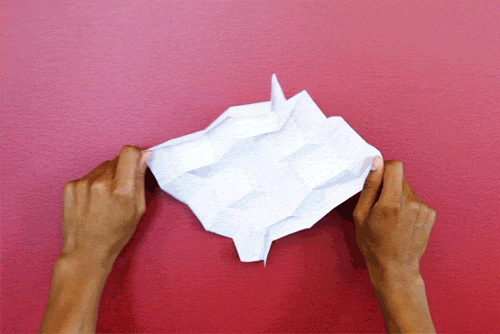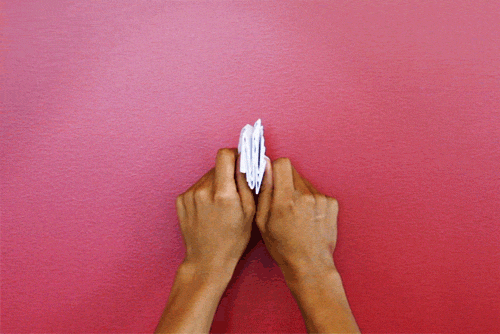
Consider the animation above, where the rectangular paper is folded and then unfolded repeatedly. They are both done by controlling the opposite vertices of the rectangular paper in a single move.

Assume that the repeating polygons have the same areas and are symmetric. Also assume that horizontal parallel lines are perpendicular to the paper's edges. Which of the following best illustrate the interior creased polygons (including those bounded by the paper's edges) in the animation after unfolding the paper?

Clarification:

Mix of quadrilaterals refer to class of 4-sided polygons not specified in the options.
A trapezoid is a quadrilateral with a pair of parallel sides. It is also known as a trapezium in UK.

All parallelograms Some squares and trapezoids Mix of quadrilaterals All squares Some squares and parallelograms Some trapezoids and parallelograms All trapezoids Mix of multiple-sided polygons

1 solution

Michael Huang
Jan 28, 2017

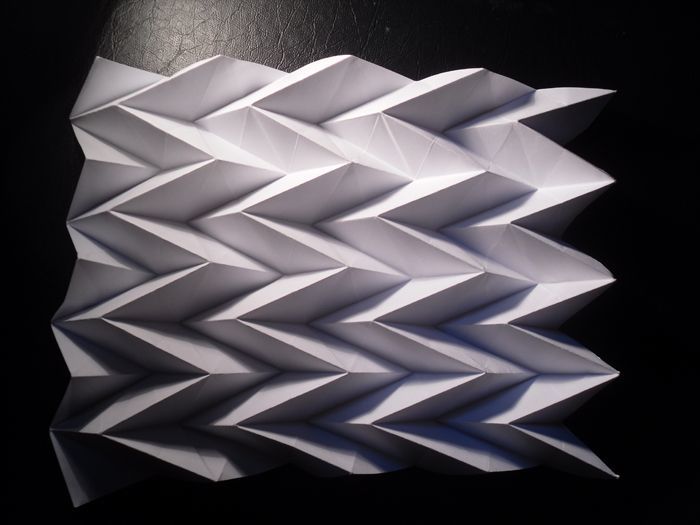
The movement of the creases is due to the inner vertices, which can be visualized as the motors during the map-opening and closing. Simply,

As you open the map, the inner vertices expand the flaps relative to the angles.
As you close the map, the inner vertices close the flaps.

The parallel zig-zag lines create the movement about the inner vertices while controlling two ends. Here is the actual creases of the paper, which form the tessellations of parallelograms and trapezoids (this is included as shown in the animation):

Moving halfway, we have the following:

If suppose the lines are orthogonal, then there is less rigidity and movement of the creases. In this case, the inner vertices do not perform any work on the creases. Here are the following real-life examples that show why right angles folds wouldn't work:

Consider human eyebrows . Then, the structure around the eyebrows do not involve $90^{\circ}$ angles. If these angles were to happen, then the eyebrows won't appear very nice at all.
Chinese lantern folds aren't orthogonal because they are made to form the smooth structure and appearance of the lantern.

For more rigorous and extensive mathematical and scientific proof, here are the articles: (1) , (2)

I actually folded up a standard letter sheet into 4 x 6 rectangles, and while holding the opposite corners of the creased letter sheet, I was able to [sort of] replicate what the video shows. There's more to this than just a matter of being able to perform this. I think we're looking at the mechanical ease of ensuring that such a creased sheet will not only reliably open up, but reliably close as well. I think Miura origami techniques are used to explore other possible geometries that would also work but work more reliably. And, as we know, solar panels in space need to be excruciatingly reliable.

Edit: This is the effect that is intended, which is clearer than what's in the video

The way I folded my letter will not do this, and that is the point of using Miura origami. But this reminds me of a problem I posted a while ago, which is related to this

Spider on Artsy Lampshade

Michael Mendrin - 4 years, 4 months ago

When you scroll up and down on the first diagram (the one with the blue and brown creases on), it seems to ripple! Nice optical illusion there!

Freddie Hand - 4 years, 4 months ago

I know... So cool...

Zoe Codrington - 2 years, 9 months ago

I hope that I adjusted the problem and make the wording more specific, noticing that the correct rate is extremely low. XD

Michael Huang - 4 years, 4 months ago

Here's a critical distinction between these Miura type folds and ordinary pleated folds. With the latter, first all the folds come together in one direction before folds in the other direction starts. With the former, folds come together in both directions at the same time. I think if you intend folds that come together in both directions at the same time, then you probably need to specify that for an unique answer. The video provided, in fact, really looks more like the former, where all the folds in one direction is done first before folds in the other direction begins. As I said, experimenting with ordinary pleated folds, I could duplicate the video, and that's not really in the spirit of the more esoteric Miura folds.

I've seen better videos of a Miura folded sheet coming apart and together much more clearly, but unfortunately they also give away the geometry much more clearly as well! Such as this one.

Miura folds

Michael Mendrin - 4 years, 4 months ago

Oh. I see. I could have been very careful with the geometry and interpretation of the valley, mountain and pleated folds.

At least it is fun knowing about the special folds. :)

Michael Huang - 4 years, 4 months ago

@Michael Huang – Yeah it's a fun subject, for sure

Michael Mendrin - 4 years, 4 months ago

@Michael Huang I suggest you clarify the statement that "the repeating polygons have the same area and are symmetric." As I understood that, the solution given does not satisfy those conditions. The trapezoid does not look like a symmetric figure and does not look like it has the same area as the parallelogram.

Thomas Raffill - 3 months ago

That question was asked few years ago. Since it was chosen Problem of the Week , the "Edit question" was taken away. Unfortunately, as I am the user, not the staff, I don't have a way to do so.

In fact, the areas wouldn't matter as it's the angles that make the difference.

Michael Huang - 3 months ago

@Michael Huang – @Michael Huang Thanks for that reply! This was a very interesting problem, and I learned something. Probably what you intended is something like "Each row has frieze symmetry" but looks like nobody has yet filled in the wiki about that https://brilliant.org/wiki/frieze-patterns/

Thomas Raffill - 3 months ago

Not Just Geometry! It's Physics!

1 solution

0 pending reports