Magnetism Series #1
Two particles of equal mass m and having unlike charges of modulus q each are placed in free space a distance r apart. A uniform and constant magnetic field of induction B is established everywhere perpendicular to the line joining the particles and the particles are released. If the magnetic field is sufficient to avoid collision of the particles, find the minimum separation between the particles.
Details and Assumptions
1)
m
=
1
0
0
2)
r
=
2
3)
B
=
3
4)
ϵ
0
=
4
The problem is taken from my Physics Book.
The answer is 1.746.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
@Karan Chatrath
the charge in the first quadrant is positive and the charge in the second quadrant is negative.
Both will attrach each other.
So the charge of 1st quadrant will experience a force toward right side which is +x direction.
Log in to reply
I don't know how else to explain to you. A force to the right would mean that charges repel, which is not so.
Log in to reply
@Karan Chatrath
I also don't know how should I explain you. Leave it.
By the way try my new problems. And if possible try to post solution.
You are a real Legend of Physics.
Log in to reply
@Talulah Riley
–
@Karan Chatrath
Oh I was thinking 2nd quadrant as 1st quadrant and 2nd quadrant as 1 quadrant.
Now everything is clear.
Thanks for bearing the pain for such a stupid mistake.
@Karan Chatrath
sir can we generalise this result? I am facing difficulty while doing that.
I will show my attempt if you reply me yes?
Thanks ina advance.
Log in to reply
I don't think it can be generalised. If the magnetic field is itself time-varying But you may shw or spatially varying, this problem becomes quite difficult. But you may show your attempt.
Log in to reply
@Karan Chatrath
Sir generalisation means just don't put values and solve till the end.
Here below I have posted my attempt, but the end when I put the values I didn't get the answer.
@Karan Chatrath
please check the solution, because at the end when I put the value I didn't get the answer
Please correct me if I am wrong.
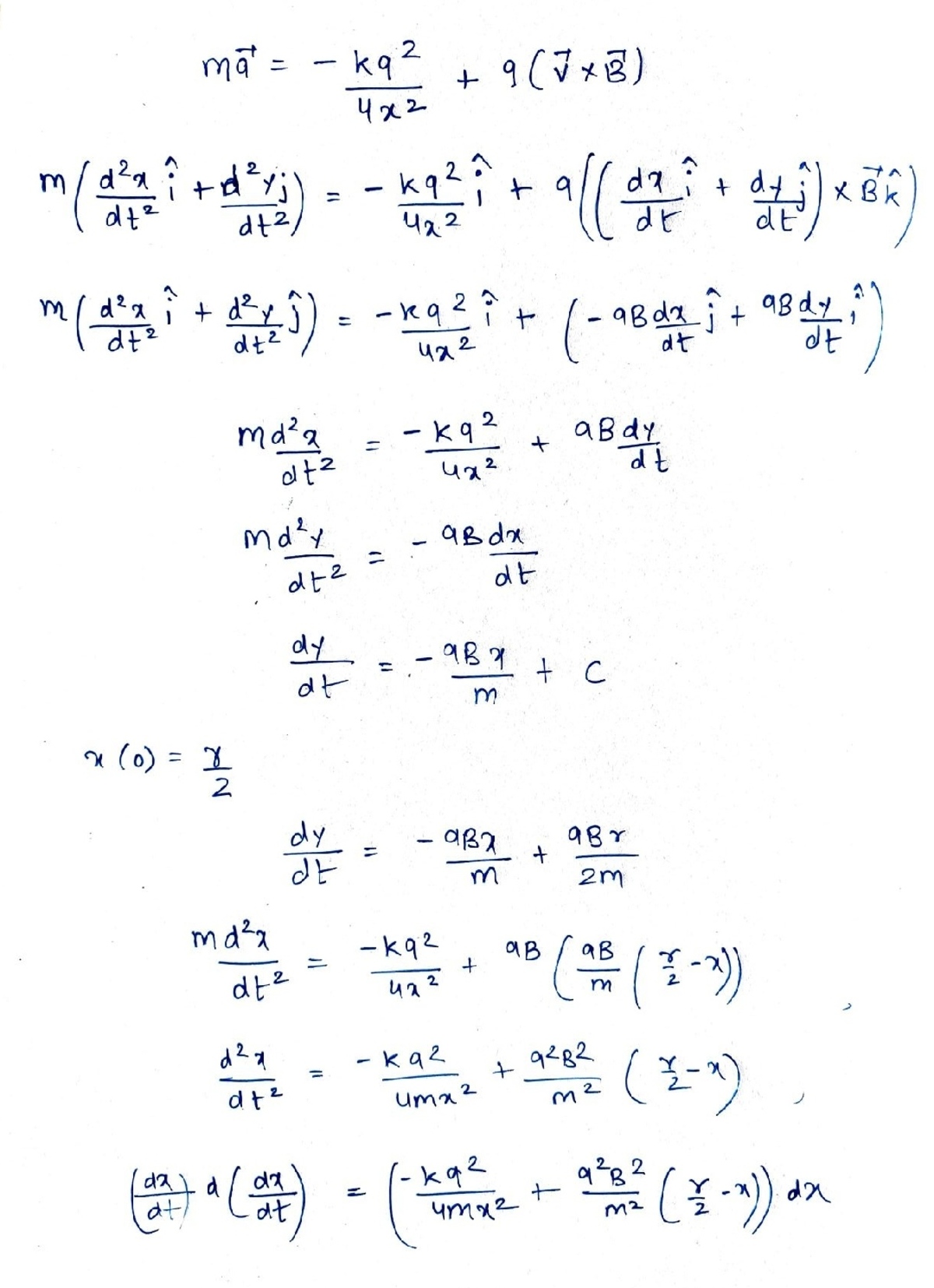
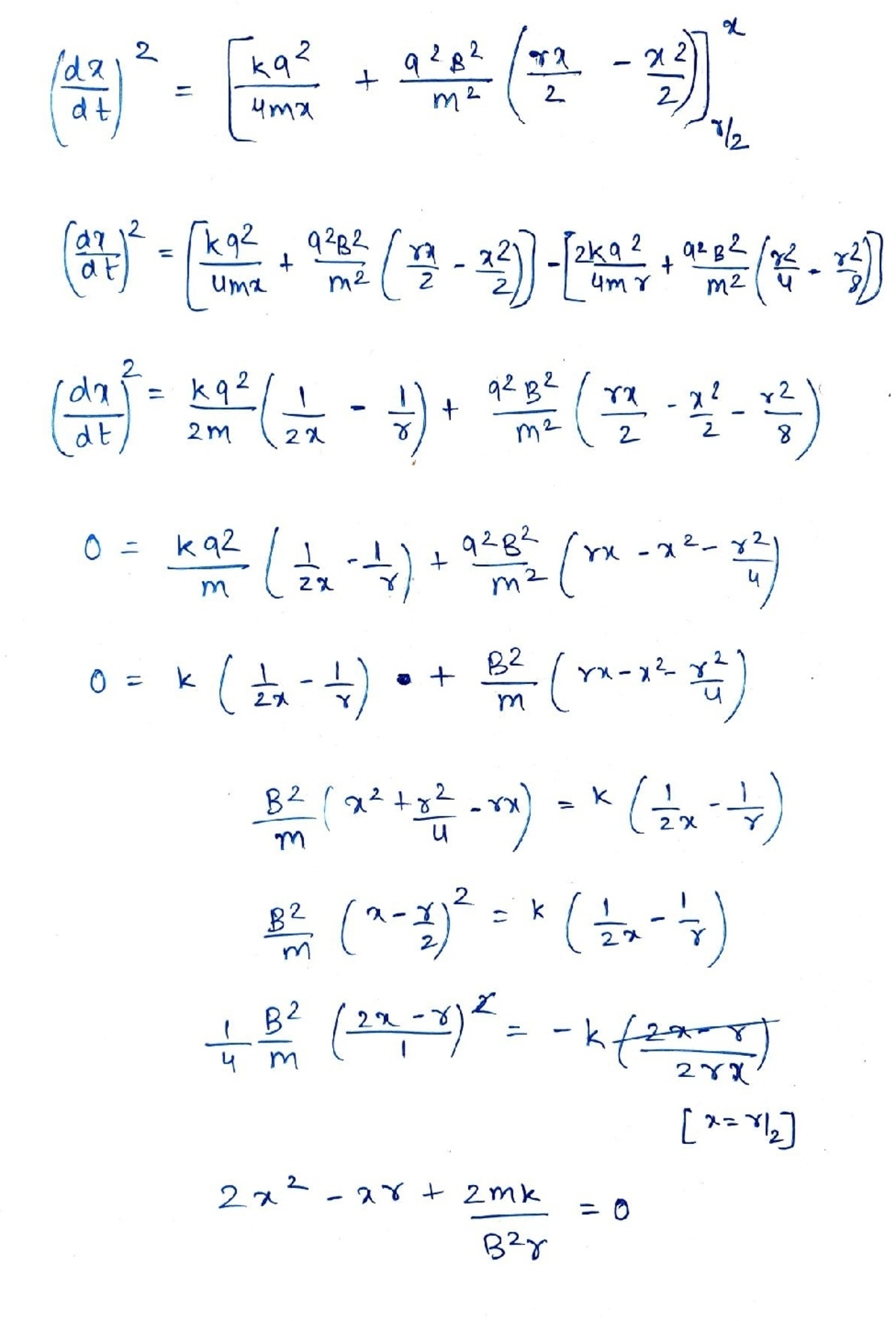
Thanks in advance.
Log in to reply
When you integrate x ˙ d x ˙ you make a small mistake there. See if you can spot it.
Log in to reply
@Karan Chatrath
But still it will not make any sense, because at the end we have put
d
t
d
x
=
0
.
?
Log in to reply
@Talulah Riley
–
@Karan Chatrath
sir you didn't give me reply after this.
Please reply whenever you get free.
Log in to reply
@Talulah Riley – Yes, it is because it is not easy to spot the mistake. After a cursory glance, I think you should recheck the steps after applying the limits of integration on the RHS.
Nice one. I just ran a numerical simulation for this. The path of the two particles is plotted below for q = 2 0 until t = 5 0 . No matter which q value is chosen, the minimum separation is always ≈ 1 . 7 4 6 .
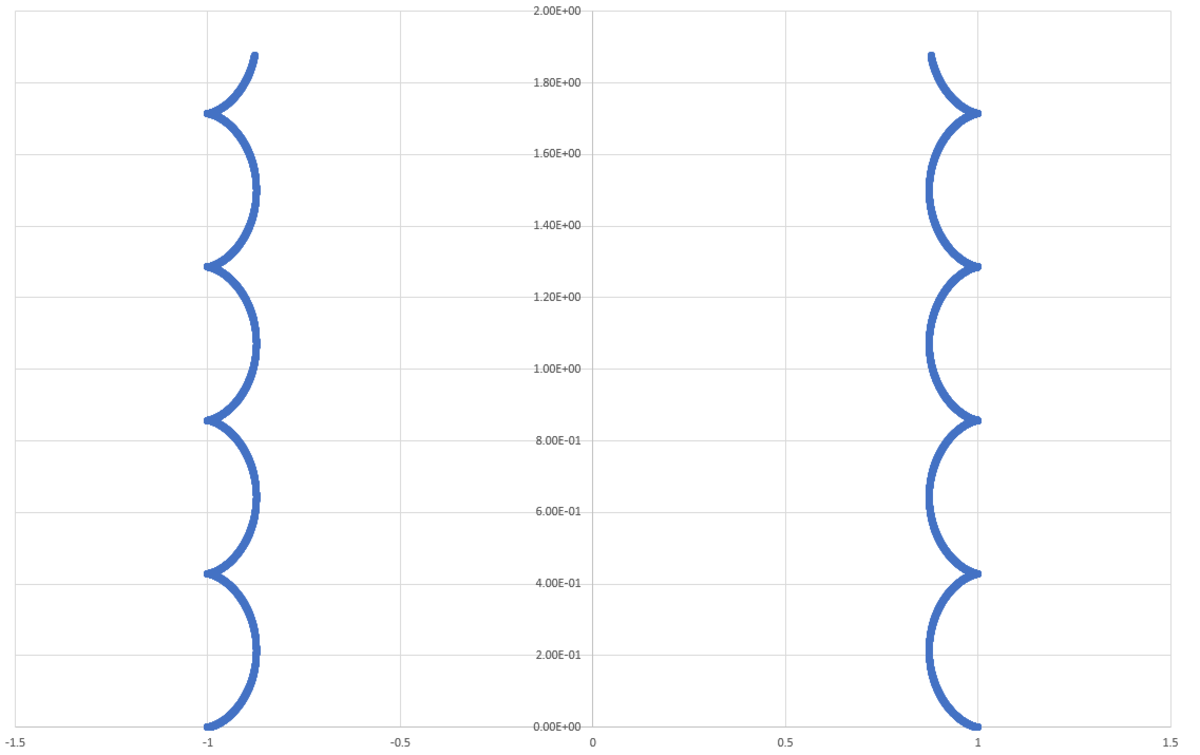
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 57 58 59 60 61 62 63 64 65 66 67 68 69 70 71 72 73 74 75 76 77 78 79 80 81 82 83 84 85 86 87 88 89 90 91 92 93 94 95 96 97 98 99 100 101 102 103 104 105 106 107 108 109 110 111 112 113 114 115 116 117 118 119 120 121 122 123 124 125 126 127 128 129 130 131 132 133 134 135 136 137 138 139 140 141 142 143 144 145 146 147 148 149 150 151 |
|
@Steven Chase
Nice solution.
Hope anyone will post a anayltical solution.
@Steven Chase
Would you like to solve the problem analytically?
The code seems me very big.
Log in to reply
This problem is actually a more difficult version of that charge separation problem you posted earlier. I don't think I will try to solve analytically, given that the numerical solution is so easy
Log in to reply
@Steven Chase
The problem has a answer given in my book.i have set the numerical value for you.
It means that anayltical solution should exist.
Log in to reply
@Talulah Riley – @Steven Chase The problem with the book is that, solutions are not available.
Log in to reply
@Talulah Riley – Have you tried Googling the problem text, or portions of it?
Log in to reply
@Steven Chase – @Steven Chase Yeah, but nothing comes.
@Steven Chase
So what are you thinking sir?
I have done whatsapp this physics question to my physics teacher but he is not bothered to reply me.
Log in to reply
I think I've done everything I'm going to do. Maybe somebody else will post an analytical solution later
@Steven Chase I have posted a new problem.
Guidelines for an analytical solution. For some reason, I cannot post a solution in the regular way. Would like to know your thoughts on it. I have left out the number crunching and evaluations.
The motion of each charge is symmetric about the Y axis. Therefore the electric force on the charge in the first quadrant is always along the negative X direction. Applying Newton's second law in 2-D gives:
m a = − 4 x 2 K q 2 i ^ + q ( v × B )
Derivation of the above is straightforward. Simplifying the above equation gives two coupled differential equations as such:
x ¨ = − 4 m x 2 K q 2 + m B q y ˙ y ¨ = − m B q x ˙
Integrating the second equation:
y ˙ = − m B q x + C
Where C is the integration constant. Applying initial conditions x ( 0 ) = 1 gives:
y ˙ = m B q ( 1 − x )
Substituting the above result in the equation for acceleration along x gives:
x ¨ = − 4 m x 2 K q 2 + m 2 B 2 q 2 ( 1 − x )
⟹ x ¨ = f ( x )
The RHS is purely an expression in terms of x . Now:
x ¨ = x ˙ d x d x ˙ = f ( x ) ⟹ x ˙ d ( x ˙ ) = f ( x ) d x
Integrating both sides and applying initial conditions:
∫ 0 x ˙ x ˙ d ( x ˙ ) = ∫ 1 x f ( x ) d x ⟹ 2 x ˙ 2 = ∫ 1 x f ( x ) d x
The minimum separation between particles occurs when x ˙ = 0 . The integral on the RHS evaluates to a non-linear expression where the numerator is a cubic equation. Solving for x ˙ = 0 essentially means solving for the roots of that resulting cubic equation. Note that x = 1 is a solution of that equation as the particle is initially at rest. Using this, the cubic equation can be converted into a quadratic equation. The solution needs to be multiplied by 2 to obtain the minimum separation.
Log in to reply
@Karan Chatrath
you should post a solution, because the hardwork you have done is really appreciable, instead of this post it as a solution.
By the way how did you
x
(
0
)
=
1
Thanks in adavnce.
Log in to reply
Thanks! But I am not able to post a solution despite solving the problem correctly. I don't know why. Maybe it is a bug in the website. Anyway, x ( 0 ) = 1 is the initial condition. The particles are separated initially by two units. See the plot shared by @Steven Chase
Log in to reply
@Karan Chatrath
–
@Karan Chatrath
Ohh, The problem in my book is not in numerical so I was expecting the distance should be
2
r
.
I forgot that i have posted the problem by setting numerical value.
By the way, the electric force should be in positive direction because both the charge will attract, why you have written negative sign??
Log in to reply
@Talulah Riley – If both charges will attract, then the charge in the 1st quadrant, which is positive will experience a force along the negative X direction.
Log in to reply
@Karan Chatrath – @Karan Chatrath Aree kaisa bhaiya jo 1 quadrant pe hai uspe +x direction pe force lagega, aur jo 2nd Quadrant oe hai uspe -x pe lagega na?
Log in to reply
@Talulah Riley – Finally managed to post an explanation. And no, the charge on the 1st quad is positive and in the secind quad is negative. Think about it..
@Steven Chase
Sir above I have posted my anayltical solution without putting values.
At the end when I put the numerical values I didn't get the answer.
Can you take a look?
Guidelines for an analytical solution.
The motion of each charge is symmetric about the Y axis. Therefore the electric force on the charge in the first quadrant is always along the negative X direction. Applying Newton's second law in 2-D gives:
m a = − 4 x 2 K q 2 i ^ + q ( v × B )
Derivation of the above is straightforward. Simplifying the above equation gives two coupled differential equations as such:
x ¨ = − 4 m x 2 K q 2 + m B q y ˙ y ¨ = − m B q x ˙
Integrating the second equation:
y ˙ = − m B q x + C
Where C is the integration constant. Applying initial conditions x ( 0 ) = 1 gives:
y ˙ = m B q ( 1 − x )
Substituting the above result in the equation for acceleration along x gives:
x ¨ = − 4 m x 2 K q 2 + m 2 B 2 q 2 ( 1 − x )
⟹ x ¨ = f ( x )
The RHS is purely an expression in terms of x . Now:
x ¨ = x ˙ d x d x ˙ = f ( x ) ⟹ x ˙ d ( x ˙ ) = f ( x ) d x
Integrating both sides and applying initial conditions:
∫ 0 x ˙ x ˙ d ( x ˙ ) = ∫ 1 x f ( x ) d x ⟹ 2 x ˙ 2 = ∫ 1 x f ( x ) d x
The minimum separation between particles occurs when x ˙ = 0 . The integral on the RHS evaluates to a non-linear expression where the numerator is a cubic equation. Solving for x ˙ = 0 essentially means solving for the roots of that resulting cubic equation. Note that x = 1 is a solution of that equation as the particle is initially at rest. Using this, the cubic equation can be converted into a quadratic equation. The solution needs to be multiplied by 2 to obtain the minimum separation.