Maximum Tension
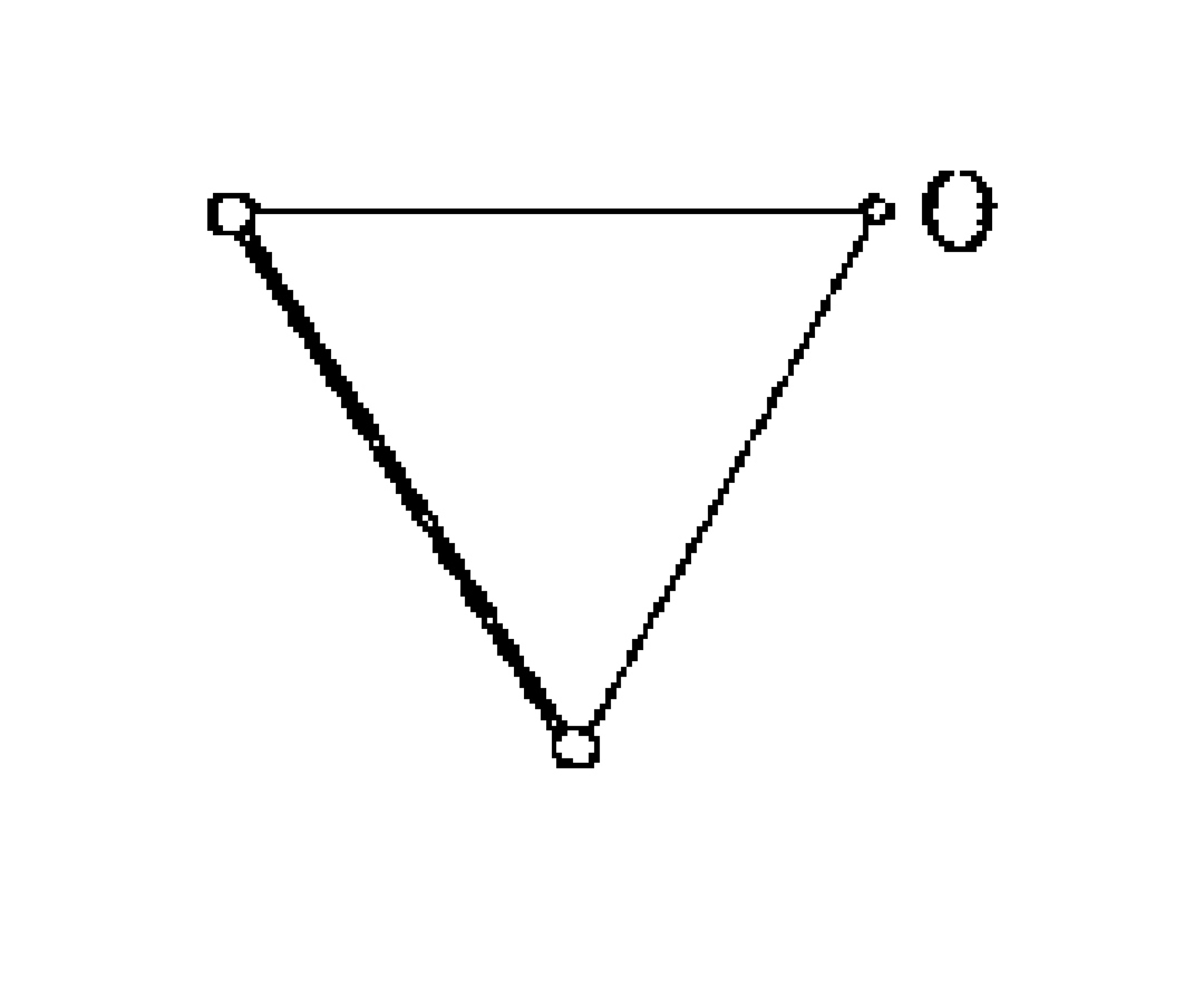
Particle-like balls each of mass
m
are affixed at both the ends of a light rigid rod of length
l
. The composite body thus formed is suspended from a nail O with the help of two light inextensible cords affixed on each ball.
Length of each cord is also
l
.
Now arrangement is pulled aside bringing one ball in level with the nail and keeping both the cords straight and
then released.
Find maximum tension
T
m
a
x
in a cord in the subsequent motion.
Answer comes in the form of T m a x = α m g
Type your answer as α = ?
Details and Assumptions
1)
Gravity is acting in downward direction and it is
g
.
The answer is 2.063.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
@Mark Hennings Thank you so much for solution.
@Mark Hennings
Sir I didi understand that line (thrust in the rod is
R
) and taking a suitable scalar product to eliminate (R)
Can you please describe that?
Thanks in advance.
Log in to reply
Take the scalar product of the equation with ( cos ( 3 1 π − θ ) sin ( 3 1 π − θ ) ) . The term in R then disappears. Since the strings make angles of 6 0 ∘ with the rod, the other inner products are nice.
Log in to reply
@Mark Hennings Thanks , sir what does thrust force mean?
Log in to reply
@Talulah Riley – The opposite of tension. If a rod is stretched, it exerts a force "inwards" at its ends. If the rod is in compression, it exerts a thrust force "outwards" at its ends.
@Mark Hennings if you don't mind,in the first line(at the last)of solution there is m missing .
[Sketch of a solution]
First thing one finds is that the each particle is constrained to move along the circle of radius l centered at point O . Hence, the whole system has one degree of freedom represented by one independent coordinate - for example, angle between vertical and center of mass of two particles. Accordingly, reaction forces must invalidate potential motion in all other directions for each particle. These conditions generate two equations of motion per particle: one for tangential and one for normal component of acceleration. The latter must include centrifugal force due to circular trajectory and thus requires knowledge of speed which can be obtained using conservation of energy principle. In the end, one gets cord tension as a function of angle and by means of differentiation finds its maximum.
@Uros Stojkovic
very nice.
Thanks for the sketch.
By the way can you please show me these steps.
If latex takes very much time you can write using pen and paper.
Thanks in advance
Log in to reply
Here are my original notes from yesterday. However, I highly encourage you to try for yourself to reconstruct solution from my sketch first.

This problem is from the Chinese Physics Olympiad (Semi Finals).
@Kushal Thaman Bro did you have qualified INPHO??
Log in to reply
Are you doing olymiad physics?
Log in to reply
@Krishna Karthik Y E S
Log in to reply
@Talulah Riley – Did you qualify? I doubt anyone else can if you can't tbh. India's pretty competitive though, then again.
Log in to reply
@Krishna Karthik
–
@Krishna Karthik
There are basically 3 levels of physics olympiad.
The 3rd level is international level.
In India you have to compete 2 level to get selected in 3rd level.
I qualified first level last year but not able to qualified 2nd level.
Hope this year I will qualify 2nd level.
I do not really understand the solution, but is it equivalent to treat the system as a pendulum using the center of the rod as the COM and then using trigonometry to find what the tension in that pendulum would be and then converting to tension in the actual rope?
If θ is the angle the trailing string makes with the horizontal, then the front string makes the angle θ + 3 1 π with the horizontal, so the system has kinetic energy T = m ℓ 2 θ ˙ 2 and potential energy V = − m g ℓ sin θ − m g ℓ sin ( θ + 3 1 π ) = − 3 m g ℓ cos ( θ − 3 1 π ) . Thus conservation of energy tells us that T + V = − 2 1 3 m g ℓ , and hence that θ ˙ 2 = ℓ 3 g [ cos ( θ − 3 1 π ) − 2 1 ] θ ¨ = − 2 ℓ 3 g sin ( θ − 3 1 π ) Thus the system oscillates between θ = 0 and θ = 3 2 π . If the tension in the trailing string is T and the thrust in the rod is R , we obtain the equation of motion of the trailing particle: m ℓ ( − cos θ sin θ ) θ ¨ + m ℓ ( sin θ cos θ ) θ ˙ 2 = T ( sin θ cos θ ) + R ( sin ( 3 1 π − θ ) − cos ( 3 1 π − θ ) ) + m g ( − 1 0 ) Taking a suitable scalar product to eliminate R , we deduce that − 2 1 m ℓ 2 θ ¨ + 2 1 3 m ℓ θ ˙ 2 2 1 3 T T = 2 1 3 T − m g cos ( 3 1 π − θ ) = 4 1 m g [ 1 0 cos ( θ − 3 1 π ) + 3 sin ( θ − 3 1 π ) − 3 ] = 2 3 1 m g [ 1 0 3 cos ( θ − 3 1 π − θ 0 ) − 3 ] where θ 0 = tan − 1 1 0 3 , which is smaller than 3 1 π . Thus the maximum value of T is α m g , where α = 2 3 1 ( 1 0 3 − 3 ) = 2 . 0 6 3 7 0 7 2 3 5 and this maximum value is achieved when θ = 3 1 π + θ 0 . Note that the tension in the leading string is just the same as the tension in the trailing string, but half a cycle of oscillation out of phase.