May the Potential Energy be with you!
A chain of mass 5 kg of uniform density is kept above the surface of the Earth in the form of a quarter circle of radius π m as shown in the figure. Find the total potential energy of the chain at this position, in joules, upto 2 decimal places.
Details and assumptions.
-
There is no air resistance.
-
Take Acceleration due to gravity = 10 ms − 2
-
Potential energy at the surface of the Earth = 0
This problem is not original, but the graph is plotted and edited by me.
I will be very glad and grateful if you post a solution.
The answer is 100.00.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
4 solutions
@Lil Doug Sir, can you please explain the following things? Thank you for your solution!
-
How did you differentiate it and the dx is coming on both sides?
-
What is written after the d in d [ ? ] = ( d x ) 2 + ( d y ) 2
-
What is λ ?
-
Which chapter is the question, I don't understand it fully, I want to read the concept.
Log in to reply
@Vinayak Srivastava Then how did you posted the problem??
Log in to reply
I found it somewhere, and the answer was given, I didn't know haw to solve.
I think you should start with a standard book either resnick halliday or concepts of physics.
@Vinayak Srivastava
Here is you answer
1)
d
x
is not coming in both sides. Consider it as my mistake.
2)
It is written
d
l
length of that element, think about Pythagoras theorem.
3)
λ
is linear mass density
4)
the question is of Centre of mass
Log in to reply
Sir what is linear mass density?
Log in to reply
@Vinayak Srivastava
λ
=
l
l
e
n
g
t
h
o
f
o
b
j
e
c
t
M
t
o
t
a
l
m
a
s
s
o
f
a
n
y
o
b
j
e
c
t
Basically mass per unit length.
Hmmm... I actually think the chapter should be in energy. Are you sure it's in centre of mass?
Log in to reply
@Krishna Karthik ya bro.
Log in to reply
@Talulah Riley – Which book is it in? Pathfinder?
Log in to reply
@Krishna Karthik – @Krishna Karthik for what thing you are talking about?
Log in to reply
@Talulah Riley – The above problem.
Log in to reply
@Krishna Karthik – @Krishna Karthik I Don't know bro. It is posted by vinayak, why are you asking me
Log in to reply
@Talulah Riley – Just thought that you knew since you said it's from centre of mass. Anyway, nevermind.
Nice one. I did it the exact same way.
I think this problem is too advanced for you, at this current stage. You should first learn a few things before you attempt a problem with such involvement in calculus, if I may be so bold to point out.
Why did you delete your problem about conservative force-fields? Was it wrong? I calculated the curl fo the force field. Is it an irrational field?
For a quarter circular ring, center of mass lies at ( π 2 R , π 2 R ) .
Therefore, potential energy
ϕ = m g y ϕ = m g π 2 R ϕ = π 2 m g R ϕ = π 2 ( 5 ) ( 1 0 ) ( π ) ϕ = 1 0 0 J
Thanks! Can you explain the first line please? :)
Log in to reply
Center of mass is basically the point where the mass of a system can be considered to be concentrated. Potential energy can be found using center of mass. For quarter circular ring, it's at a point I mentioned. For more, you could visit this Wikipedia page . :)
Log in to reply
Is there an easier way to calculate center of mass, as I am very new at integration, like using some geometry, etc.?
Log in to reply
@Vinayak Srivastava – Actually semicircular ring has at π 2 R (memorise it), and so it's same for quarter circular by symmetry.
@Aryan Sanghi There is nothing different method in your problem.
Log in to reply
Ok,You are right. But,from a beginner's perspective or competitive perspective (Where you have less time ) it's quite useful.
Log in to reply
@Kriti Kamal but waht if you didn't know that center of mass of ring lies at that?
Log in to reply
@Talulah Riley – Actually, a person would normally remember as in many problems, semicircular center of mass ( 0 , π 2 R ) is used. And, quarter circular center of mass is a direct result of this. :)
Log in to reply
@Aryan Sanghi – @Aryan Sanghi but i don't remember.
Log in to reply
@Talulah Riley – You are a genius,but I am not.
Log in to reply
@A Former Brilliant Member – @Kriti Kamal who said I am a genius?
i have posted the solution,but i am quite unconfident about it, actually gravitational potential energy=-GmM/r and in your question it is given that the potential of ground is zero,so we should find the relative p.e. However,it will change the answer slightly. @Vinayak Srivastava , @Krishna Karthik , @Lil Doug .
Log in to reply
Yeah; looks to me as though your solution's correct. What's wrong with using m g h as gravitational potential energy?
Log in to reply
@Kriti Kamal
for small distance we generally use mgh only.
But the perfect formula for gravitational potential energy is -GMm/r only.
Log in to reply
@Talulah Riley – That is true. Of course, in our "Earth" reference frame this is ignored. In fact, the effects of gravitational force actually decrease as distance gets larger, but this is negligible for small distances. So for this question, it's fine to use m g h .
Log in to reply
@Krishna Karthik – You are right in a manner,but here in question answer is required to correct over two decimal places,which we can't obtain from mgh
Log in to reply
@A Former Brilliant Member – Seriously? A difference in height of 3 meters brings about differences in gravitational acceleration less that 0.0001 meters per second squared. The potential energy will be perfectly fine to find. The gravitational force is negligibly different.
Log in to reply
@Krishna Karthik – Yes,but you should try out yourself and then think gravitational accleration is changed by 0.0001 but potential energy will of order 0.02
actually potential energy is defined as negative of work done by conservative. And here is gravitational force which is equal GmM/r^2,by just using slight integration,you can find that gravitational potential energy = -GmM/r。Mgh is just an approximation,when h<<<Radius of source mass. You can read it from here
Log in to reply
@A Former Brilliant Member – I know that m g h is an approximation. But for differences of 3 meters, assuming it is at sea level, the difference is extremely small.
I realise that the gravitational force changes with distance between the two bodies, but m g remains almost the exact same between sea level and 3 m above sea level.
Log in to reply
@Krishna Karthik – Ok,thanks for your response.
@A Former Brilliant Member – Come on... discussing such a triviality is like saying "at 3m/s you have ignored the effects of special relativity".
@Kriti Kamal , I think I can really understand your solution, thanks! Is it normal in these kinds of problems, or you thought of it yourself?
Log in to reply
actually,it is normal in such questions and you will get the thought process when you will practice such questions.
。。。。。。。。
As you have to calculate the potential energy of the whole ring,but it is quite clear that different parts of rings are at different places from ground.So,you have to find each and then add all.And since ring is continuous and a well known geometric entity.So,you can directly integrate.
As you learn rotational mechanics and centre of mass,then these types of problems become common to you.
You can start with HCV or Resniky halliday.
Log in to reply
Ok, I will do these books when I am done with school and NSEJS specific coaching books. Thanks!
@Vinayak Srivastava ,why you have deleted the your new problem?
Log in to reply
I mean both the answer and question are correct,then why?
Log in to reply
I am not sure of the answer.
Let us solve the problem in general.
Let the mass of the chain be m , radius of the quarter circle be r , acceleration due to gravity be g .
Consider a small element of the chain of length d l = r d α at a point at an angular position α from the base line, which is the surface of the earth. Height of this point above the earth's surface is h = r sin α .
Length of the chain is l = 2 π r , so that the mass per unit length is π r 2 m .
Mass of the element is d m = l m × r d α = π 2 m d α ,
and it's potential energy is
d ( P . E . ) = π 2 m g r sin α d α
So the potential energy of the entire chain is
P . E . = ∫ 0 2 π π 2 m g r sin α d α = π 2 m g r
Now for the given problem, m = 5 , g = 1 0 , r = π
Hence the required potential energy is
P . E . = π 2 × 5 × 1 0 = 1 0 0 Joules.
Sir I am unable to see the lines of fractions, is it happening with you also? Also, there is a typo in last line. Thanks for your solution!
Nice one.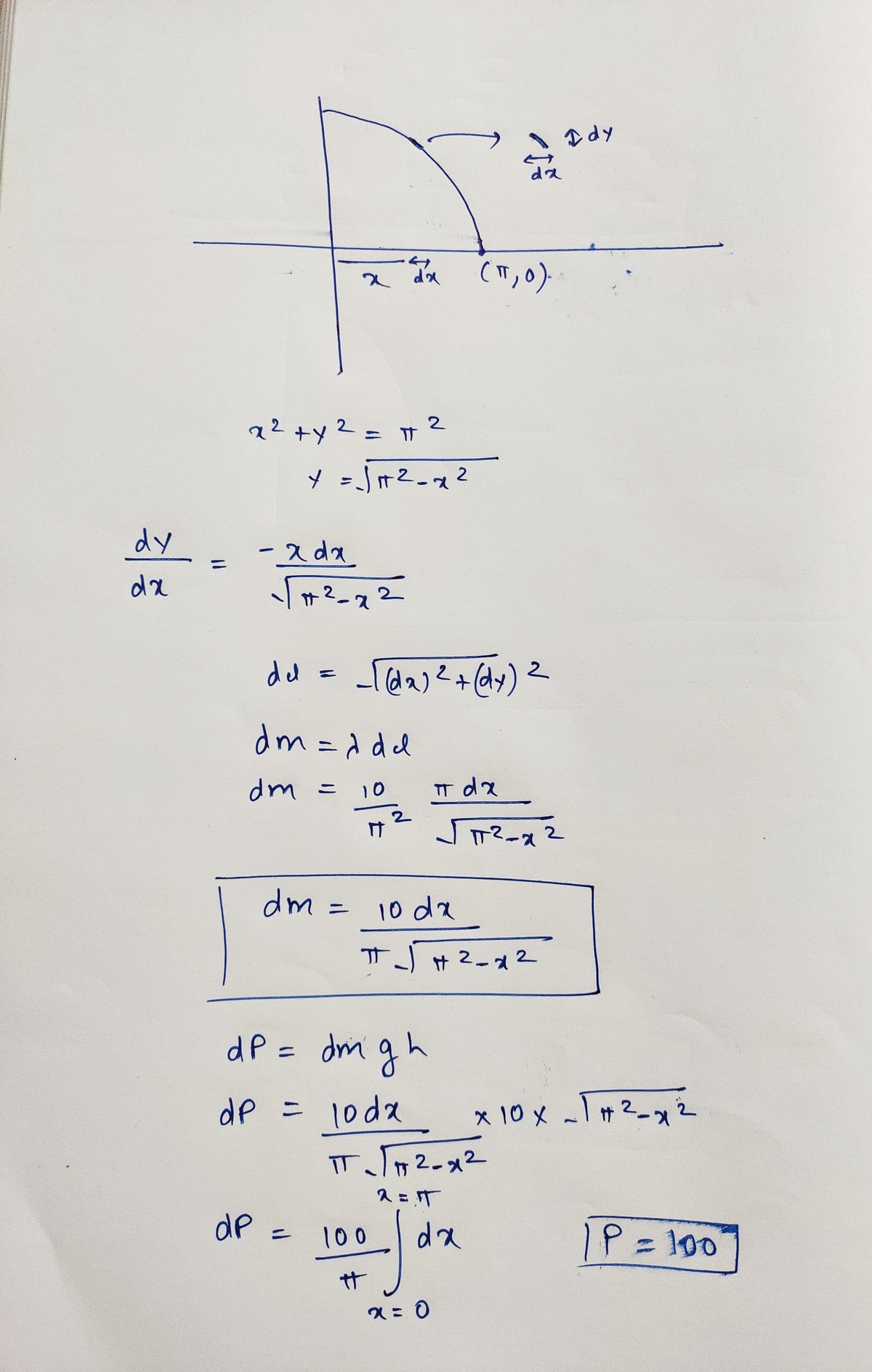
Consider everthing in SI units in my solution.