NSEJS 2015 Problem 2
Consider a parallelogram with adjacent sides 2 0 m and 3 0 m . If one of the diagonal of the parallelogram is 4 0 m .
Find the length of the other diagonal.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
7 solutions
so isnt this an over-rated question because of such easy options ....
Log in to reply
I'd say so. I only used this method because I expected to eliminate one or two obviously incorrect answers. When I eliminated 3, I saw that my work was done.
Even I used same approach.
I attempted this question in my NSEJS paper.Its from 2015 paper right ?
really nice solution brother .. nice approach .. HATS OFF ... i never got this idea
I use the law of cosines:
400^2 = 30^2 +20^20 - 2 (30)(20)cosC C = 104.5°
This is the measurement for the angles not cut by the 40m diagonal.
Then : 180° - 104.5° = 75.5° is the measure of the other pair of angles.
Then I use the law of cosine again to find the length of the other diagonal.
C^2 = 30^2 + 20^2 - 2 (30)(20)cos (75.5) C = sqrt (1000) = 10 sqrt (10)
- I apologize for the format but I am working from my cell phone.
1 8 0 − 1 0 4 . 5 = 7 5 . 2 ?
I think none of us remember cos 1 0 4 . 5 ∘ .
Log in to reply
Let a=20 =AD=BC and b=30=AB=CD and diagonal be x=BD=40 and let the missing diagonal be y Here by cosine rule a^2+b^2-(2ab)cos A= x^2 We know that cosD = -cosA , therefore a^2+b^2+2abcosA=y^2 Therefore adding the two equ we get 2(a^2)+2(b^2)=x^2+y^2 Putting the values for a,band x we get 2(400)+ 2(900)=1600 +y^2 2600-1600= y^2 Therefore y^2= 1000
Log in to reply
Can you please use latex code ?
Can't we use the parallelogram law? Sum of the squares of the sides of the parallelogram = Sum of the squares of the diagonals.
I used it and got the right answer;
20^{2} + 20^{2} + 30^{2} + 30^{2} = 40^{2} + x^{2}
2600= 1600 + x^{2}
x^{2}= 1000
x= 10 sqrt{10}

I used co-ordinate geometery to solve the problem,
After I had plotted the quadilateral(As shown in the image), I had to determine the value of co-ordinates of point D,
2 0 2 = ( x 1 − 0 ) 2 + ( y 1 − 0 ) 2
4 0 0 = ( x 1 ) 2 + ( y 1 ) 2
Now,
4 0 2 = ( x 1 − 3 0 ) 2 + ( y 1 − 0 ) 2
1 6 0 0 = ( x 1 ) 2 + 9 0 0 − 6 0 ( x 1 ) + ( y 1 ) 2
Placing values,
7 0 0 = 4 0 0 − 6 0 ( x 1 )
x 1 = − 5
And, y 1 = 3 7 5
Now co-ordinates of point C,
x 2 = 3 0 − 0 + ( − 5 ) , y 2 = 0 − 0 + 3 7 5
x 2 = 2 5 , y 2 = 3 7 5
Now we just need to find the distance AC,
( A C ) 2 = ( 2 5 − 0 ) 2 + ( 3 7 5 − 0 ) 2
A C = ( 6 2 5 + 3 7 5 )
A C = 1 0 0 0
A C = 1 0 1 0
It will look better if you turn the image by 9 0 ∘ .
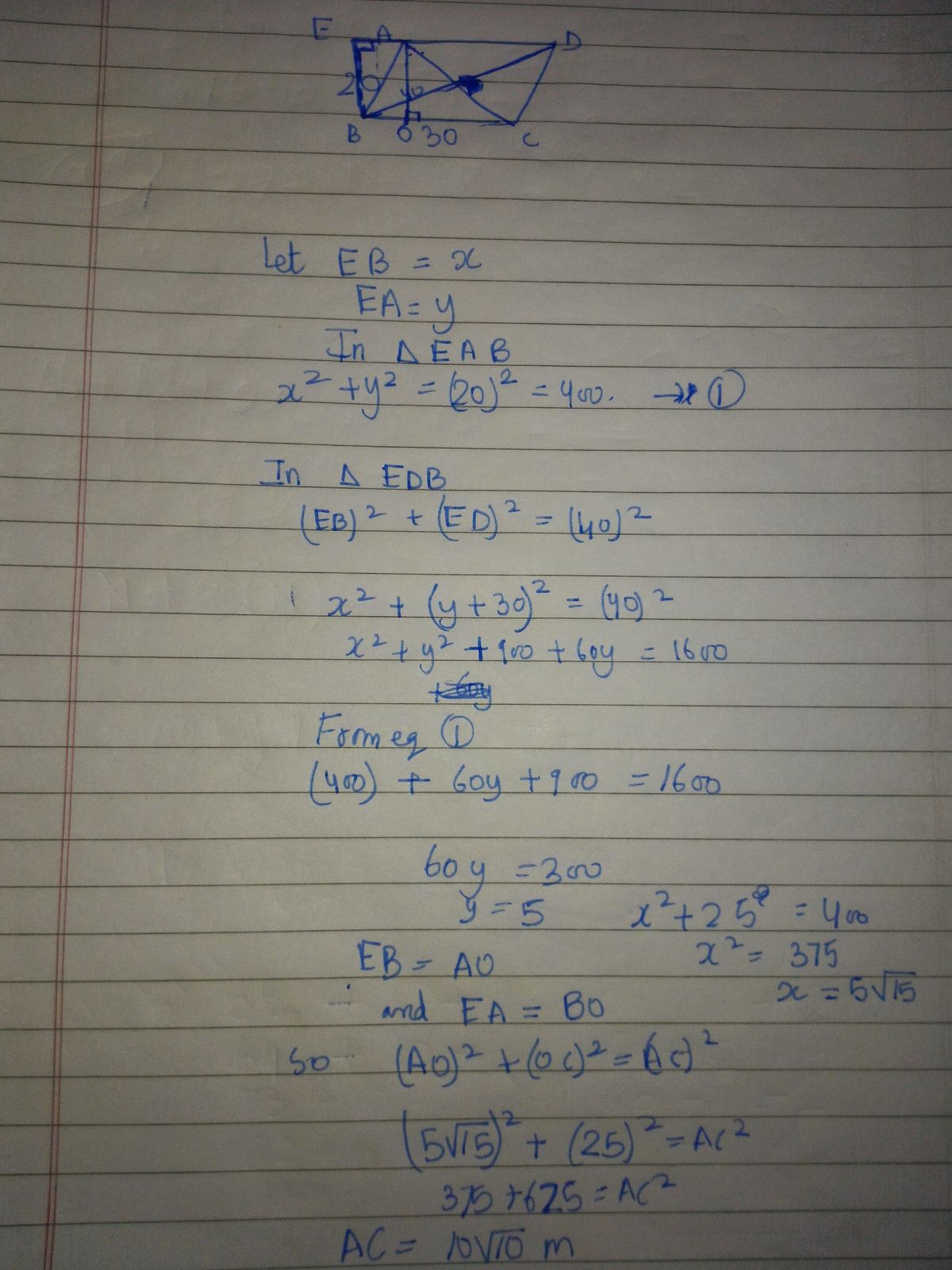

I just used process of elimination:
There will be a triangle formed with side lengths of 20, 30, and x; where x is the length of the missing diagonal. The Triangle Inequality says that the sum of the two shorter sides is greater than the length of the longest side.
100 5 is not the answer, because 100 5 > 20 + 30
5 2 is not the answer, because 30 > 20 + 5 2
10 1 0 is a possible solution, because 10 1 0 < 30 + 20
50 is not the answer because 20 + 30 = 50.
Since a, b, and d are impossible; the answer must be 1 0 1 0