One more of those

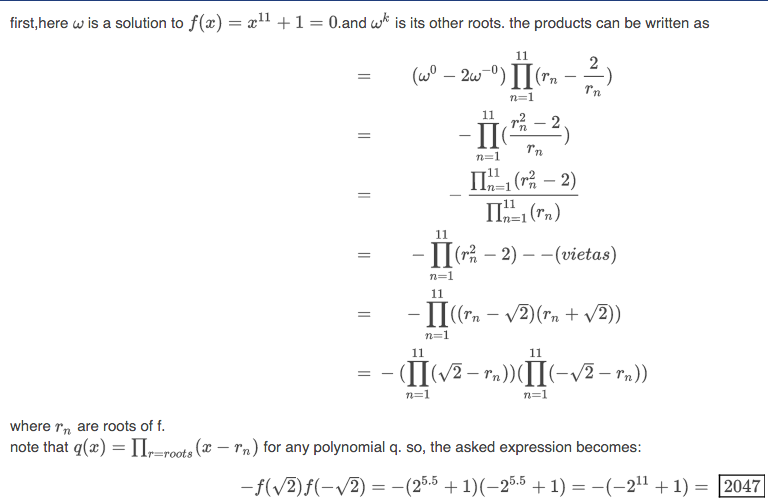
Keep in mind throughout that $w^{22}=e^{2\pi{i}}=1.$

Since the even powers of $w$ are the 11th roots of unity, we have $z^{11}-1=\prod_{k=0}^{10}(z-w^{2k})$ . We plug in $z=2$ and find $2^{11}-1=2047=\prod_{k=0}^{10}(2-w^{2k})=\prod_{k=0}^{11}(2-w^{2k})=\prod_{k=0}^{11}w^k\prod_{k=0}^{11}(2w^{-k}-w^{k})$ $=w^{\frac{11\times12}{2}}\prod_{k=0}^{11}(w^k-2w^{-k})=\prod_{k=0}^{11}(w^k-2w^{-k})=\boxed{2047}$