Only 4 Square Roots
If x + x + x + x + 2 0 1 8 = 2 0 1 8 , what is x ?
The answer is 4070306.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Is it intuitive to put x + a = a 2 ? How do you come to know what substitution to do?
Log in to reply
I remembered how Ramanujan came up with his formula for infinite nested radicals.
Log in to reply
Can u share a link of which problem you are talking about
Log in to reply
@Keshav Kasat – You can read this wiki for information on nested radicals.
After seeing your solution, I now kind of feel embarrassed for using a CAS to solve the problem…
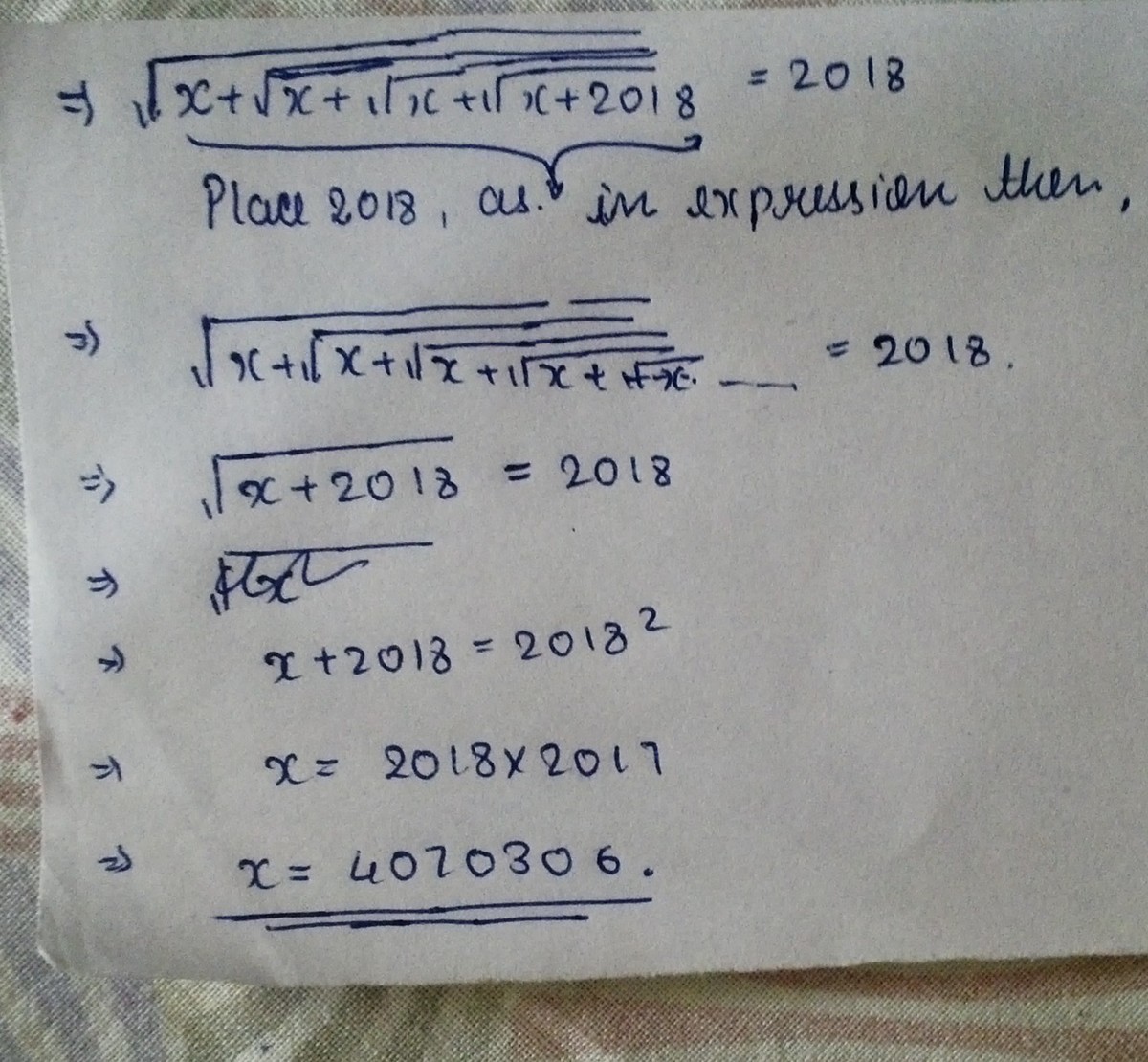
Consider the following:
y = x + x + x + x + a = x + x + x + a 2 = x + x + x + a = x + x + a = x + a = a Putting x + a = a 2
Implying that if x + x + x + x + a = a , then x + a = a 2 ⟹ x = a 2 − a . For a = 2 0 1 8 ⟹ x = 2 0 1 8 2 − 2 0 1 8 = 4 0 7 0 3 0 6