Oscillating Circuit
In an oscillating circuit , the coil inductance is equal to L and the capacitor have capacitance
C
1
and
C
2
. The capacitor were charged to a voltage
V
, and then the switch
S
w
was closed.
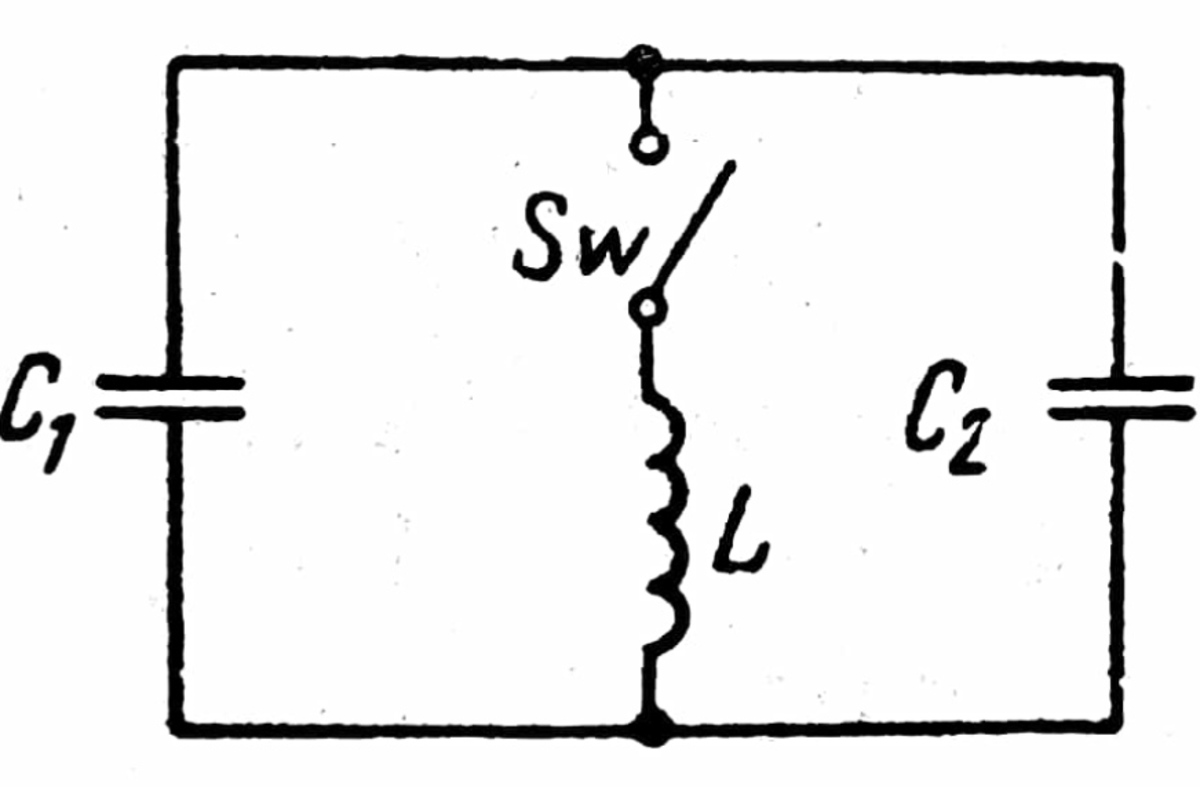
Find the peak value
(
I
m
a
x
)
of current flowing through the coil in Ampere.
Details and Assumptions
1)
L
=
2
.
5
×
1
0
−
3
H
2)
C
1
=
2
×
1
0
−
6
F
3)
C
2
=
3
×
1
0
−
6
F
4)
V
=
1
8
0
V
5)
The wire used in the circuit have
0
resistance.
The problem is not original.
The answer is 8.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
1 solution
@Steven Chase please help me in this problem, I will share my opinion if you say yes.

If I use a small angle approximation, I get the following:
D m i n = 2 ℓ cos ( q 2 1 6 π ϵ 0 m ℓ v 2 )
Log in to reply
@Steven Chase
Sir answer according to book
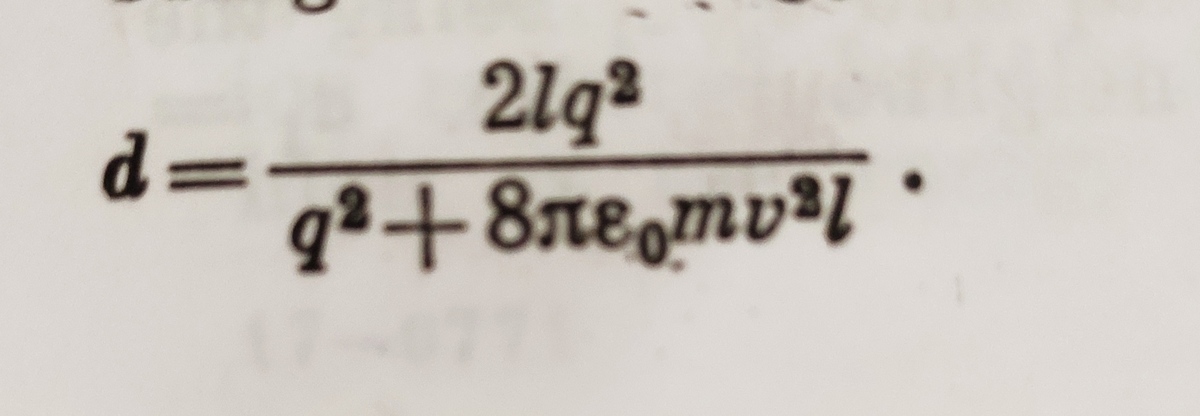
Please share your whole approach.
I will share the solution of the book, if you say.
I think you solution will be correct because I believe in you more than the book.
Log in to reply
Keeping in mind that the argument of the cosine function is small, my answer is equivalent to this. If you do a two-term Taylor expansion of the cosine, and then do a little more algebraic manipulation, you get the same answer as the book. The solution is fairly involved, so I will post it tomorrow. I am going to bed soon.
Log in to reply
@Steven Chase
–
@Steven Chase
ok sir Thanks. This is the book solution, which is very small and it seems me incorrect also. In your opinion it is correct or not??
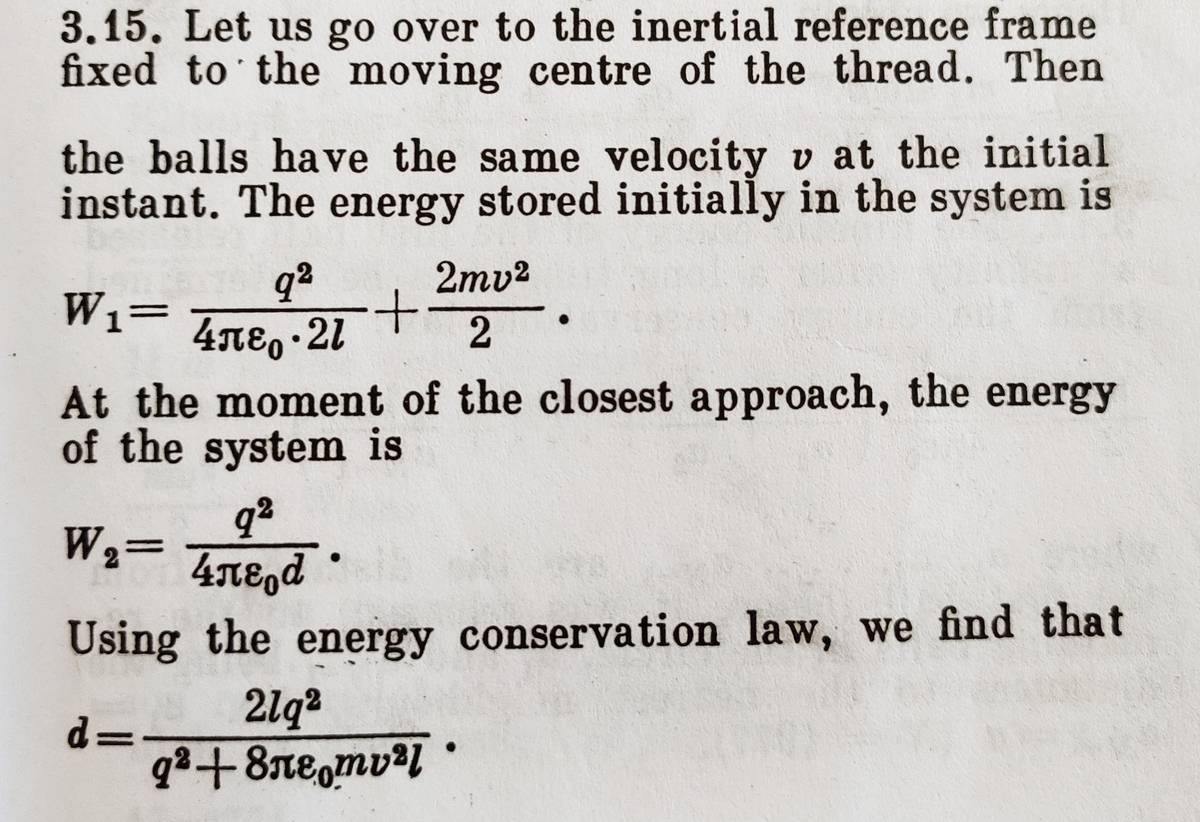
Log in to reply
@A Former Brilliant Member – Yeah, it looks right. That approach is simpler than mine, but they both yield the same answer (just in different forms)
Log in to reply
@Steven Chase
–
@Steven Chase
but in the solution how can we write
2
2
m
v
2
I do not think that both the ball have equal velocity equal to v. After that the instant when string is pulled with velocity v. And in this system in my opinion in any time both balls can't have velocity equal to v.
Please correct me if I am wrong
Log in to reply
@A Former Brilliant Member – That's what I thought originally. But then I read more closely and saw that they are using a reference frame which coincides with the center of the string. And from that point of view, the masses are moving initially.
Also, do you mind if I use the image from that 6th-order LC problem to post a follow-up problem?
Log in to reply
@Steven Chase
–
@Steven Chase
, no
I will be happy
@Steven Chase – @Steven Chase please share your solution of that question. Is it necessary that when the closest approach comes the velocity in y direction will be zero. ????
Log in to reply
@A Former Brilliant Member – I have posted my solution in the notes section. Referring back to the book solution, on closest approach, the charges are at rest with respect to the middle of the string. And since the middle of the string is moving upward, I suppose that means the charges are as well.
@Steven Chase
sir i have learned that how to solve a single integral using midpoint theorem .
before solving double integral i want to solve differential equation.
Which method is best for solving differential equation ?
I have taken a simple 1st order differential equation from my maths book .
x
d
x
d
y
+
y
=
x
3
y
4
how can i solve this ?
Thanks in advance .
Log in to reply
You can isolate the derivative term and then numerically integrate
Log in to reply
@Steven Chase sir btw how can i plot α vs θ in excel α = 2 sin 2 θ 3 − 1
@Steven Chase
sir can you please solve the above differential equation and share the code with me after that i will solve 1st differential equations myself .
i wii take your code as an example.
thanks in advance
Log in to reply
@A Former Brilliant Member – If you have an analytical solution, you can use my initial conditions and check my result
You have to be careful with this one, because the function can blow up very easily if not initialized in a sensible way.
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52 53 54 55 56 |
|
Log in to reply
@Steven Chase
Thanks sir.
BTW I have noticed that sometimes you write 1000% and sometimes you write 100%
What is logic behind that?
Log in to reply
@A Former Brilliant Member – Sometimes I want to print once for every hundred simulation steps, and sometimes I want to print once for every thousand.
Log in to reply
@Steven Chase
–
@Steven Chase
sir can you post a RLC problem.
I will try my best to solve using Python.
Thanks in advance
@Steven Chase
sir are you free now??
I want to ask you 1-2 simple doubts of kinematics.
Thanks in advance.
Combined capacitance:
C = C 1 + C 2
The energy in the circuit transfers back and forth between the capacitance and the inductance:
2 1 L I m a x 2 = 2 1 C V 2 I m a x = L C V 2 ≈ 8 . 0 5