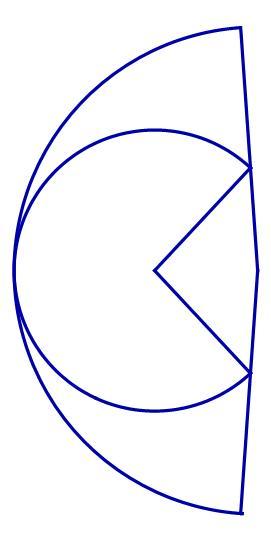
Pac man cannibal

If the red pac-man has radius 1, and $2\theta$ is the angle of its mouth, then the yellow pac-man's radius is $\frac{1}{2\cos{\theta}+1}$

The ratio of the areas simplifies to $(\frac{1}{2\cos{\theta}+1})^{2}\frac{180-2\theta}{180-\theta}$ which is too messy to give an exact analytical solution.

Wolfram|Alpha says this is minimized if $\theta=46.58985859^{\circ}$ and so $2\theta=\boxed{93.17991718^{\circ}}$ and Geometer's sketchpad agrees.

A picture of this looks funny because the yellow pac-man's mouth is open more than 180 degrees.