Patrick's equation
Find the sum of all solutions to the equation
( x 2 + 5 x + 5 ) x 2 − 1 0 x + 2 1 = 1 .
The answer is 2.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
15 solutions
i forgot case 3 hahhaha
Log in to reply
me too hahaha
Same here .
Ugh I did as well
Me too. Even I forgot the third case.
Me too. I forgot to consider it at my first attempt. Then i got it in my second attempt.
hehe, same thing godammit
Me too, damit
me also forgot.. ha ha ha
Log in to reply
I missed 3 r d case too :-)
hahaha first time i missed case 3 too
Hmm i did this problem in a different way but I cant seem to find out why I cant get the 3rd case. What I did was to Lg both sides to give Lg(x^2+5x+5)^x2-10x+21=Lg1
Bring down the power of the Lg to give (x^2-10x+21)(Lg(x^2+5x+5))=0
factorize the exponentials, (x-7)(x-3)(Lg(x^2+5x+5))=0 From this we can see that x=7 or x=3. Also, Lg(x^2+5x+5)=0, so x^2+5x+5=1 Solve x^2+5x+5=1 gives (x+4)(x+1)=0, thus x=-4 or x=-1
So where did I go wrong? Please help thank you! :)
Log in to reply
When you take the Lg and bring down the exponent, the function inside the Lg must have absolute value, e.g. Lg |x^2 + 5x + 5| and then equating this Lg | ... | to 0 will give you (x^2 + 5x +5) can be equal to both +1 and -1. Solve for both cases and use the conditions for absolute value to eliminate x = -2 or plug this solution into the equation to double check. Honestly, I forgot the -1 case, too. :)
Gerry you did not do anything wrong. Using the logs just makes the third case more difficult to see. Note that if the base is negative then its log is a family of complex numbers. The natural log of negative one is i π times the odd integers. We are only interested in real numbers here, so at that point you should just go back to the original form of the equation and contemplate it.
Add modulus sign inside the log
I would love to help you, but I'm not very introduced in logaritms and I don't know how to use them well, sorry
Oh, I also missed the 3rd case, too.
can you please explain the 3rd point again, why it would be -1 ?
But the question is given as x^2 + 5x + 5
Log in to reply
when that equation is equal to 1 sub. 1 from LHS
How can u make sure that there are only 3 cases ?
Log in to reply
Let' s assume that a b = 1 . Since a = 0 there are two possibilities a > 0 or a < 0 .
Case 1( a < 0 ) . In this case a b = ( − 1 ∗ ∣ a ∣ ) b = e b ∗ π i ∣ a ∣ b . According to this, the result can be a positive real number if and only if b ∗ π = 2 n π where n is an integer number. Therefore b must be an even number. Now we have that a b = ∣ a ∣ b = 1 . Taking logarithms we obtain b ∗ ln ∣ a ∣ = 0 . Then b = 0 or a = − 1 .
Case 2 ( a > 0 ). This case is even easier. Since a b = 1 taking logarithms we obtain that b ∗ ln a = 0 . Then b = 0 or a = 1 .
That is why there are only 3 possibilities: a = − 1 and b an even number; b = 0 and a = 0 ; or a = 1 and b any real number.
By solving it using logarithm.
god!!!!!!!!!! forgot to add the values -3 nd -2
i cant understand third point
Log in to reply
It is quite simple: ( − 1 ) 2 = ( − 1 ) ∗ ( − 1 ) = 1
( − 1 ) 3 = ( − 1 ) ∗ ( − 1 ) ∗ ( − 1 ) = − 1
So because the equation has a solution (-1) must be raised to an even number and this happens when -3 is the solution, in this case (-1) is raised to 60.
i forgot the third case. crap.
aarrggh, i forgot the third case
darn...i forgot case 3..
haha. me too
I too missed the third case in first attempt XD
How can you say that only -3 gives a solution to the third case?
why can't we say that x^2 -10*x + 25 = -4
solving we get x=5
Log in to reply
But sir,did you mean x^2-10x+25=0? And how have you come up with x^2-10x+21=-4? Where does the -4 come from?
C H E E R S
Just remembered the third case in time :P
Forgot case 3 damn
I didn't miss the third case :P
Probably the best question.i hav ever seen.it can be given as a object type question. Easy yet difficult.this is what i call a tricky question.
Completely forgot case 3!
Haha,u r wrong,-3 dosent satisfy d equation lol.
All fools check : the third case give two values which are garbage as they dont satisfy d equation
Did it in a similar way.
Isn't there a 4th case when base is 1 and exponent is -1?
Log in to reply
X is common in both, the base and the exponent. So if u equate the base to 1, there is no guarantee that exponent could me made equal to -1, because u are fixing the value of x while equating the base to 1. And anyways, it would not be a different case as 1 to the power anything, is always one. So it would come in the second case. Hope that i am clear in my explanation :)
That is covered in "The base is 1", where we didn't have to add a restriction to the exponent. In particular, the exponent could be -1 (or anything else).
A very simple and elegant problem. I solved it the same way!!!
@Rajdeep Bharati Please keep comments on a solution relevant to the particular solution, instead of commenting about it elsewhere.
This solution is correct. It is based on the same cases which lead to a b = 1 . I'm not sure what you mean by "Shouldn't they have neglected some cases?".
Why are those the only three cases?
Log in to reply
I guess the problem should have said that the answers have to be whole numbers in that situation only three cases are possible and the third one was ignored by most of us. Yes a seemingly easy problem but as someone said about an exam "I agree this is an easy exam, it is easy to fail" I missed it. Congratulations for devising such a question.
how did you get x^2 + 5x +4 & x^2 + 5x +6 when it's x^2 + 5x +5 ?
Log in to reply
You have to make the Lefthand side equal to zero. In one case, you try to find (x² + 5x + 5) = 1, in the other you want (x² + 5x + 5) = -1 and the exponent be even.
Anyone Knows from where this problem is picked??
Log in to reply
From Brilliant :) Once you understand the underlying principle, it's not hard to create questions like this.
how do you know that the third case is possible?
I forgot ( − 1 ) k , k is even number.
As far as I know: x = ( 1 6 + e 2 k π / c − c ) / 1 5 and x = ( 1 6 + e 2 k π / d − d i ) / 1 5 for any integer k and nonzero reals c and d are all solutions!
The first leads to ( e 2 k π i / c ) c = 1 ;
the second leads to ( e 2 k π / d ) d i = 1 .
why taking the natural log of both sides doesn't give me -3 as a solution ??
Log in to reply
Likely because you/the caculator required the natural log to be positive, whereas the solution of -3 arises when x 2 + 5 x + 5 = − 1 .
Read the solution for why this happens.
Be careful bro, you must Forget -2
There are only four solutions. Wrong answer. The roots are -4, -1, 3 and 7 which gives a total of 5.
Log in to reply
What happens when you put in ( x = - 3 )? What does the LHS look like?
What a great problem. I leaned alot.. I am delighted i found the first 2 cases!
T T missed case 3 - -"
Everyone seems to miss case 3 including me D:
why not -2?
@Jordi Bosch This is incomplete. You also need to check the condition in Case 1 when the base as well as the exponent is equal to 0 . However in this scenario, it won't affect the solution, but for future reference, completeness of the solution is required.
But it's x2+5x+5!
Consider the cases when a^b = 1
This can only occur when a=1, b=0, and a=-1 where b is even.
Consider case one, where a=1
This implies that: x^2 + 5x + 5 = 1 x^2 + 5x + 4 = 0 (x + 4)(x + 1) = 0 So we now have two solutions, x=-4 and x=-1
Consider case two, where b=0
This implies that: x^2 - 10x +21 = 0 (x - 7)(x - 3) = 0 This gives two solutions, x=7, and x=3
Consider case three, where a=-1, and b is even
This implies that: x^2 + 5x + 5 = -1 x^2 + 5x + 6 = 0 (x + 3)(x + 2) = 0 Which gives x=-3 and x=-2 However, we discard x=-2 as it gives rise to an odd value of b (45)
This leaves us with the final solutions: -4, -1, 7, 3 and -3 which sum to 2
why we discard -2
Log in to reply
because at -2 x 2 − 1 0 x + 2 1 is not even and -1 raised to an odd power results in -1 not 1
Easy solution to understand :)
Sum of root three quadratic equations is equal to 2
There's also a case when x²+5x+5=i AND x²-10x+21=4n to consider. But it yields no solutions.
x 2 + 5 x + 5 = 1 yields solutions -1 and -4
x 2 + 5 x + 5 = − 1 and since the exponent must even, this yields solution: -3 (not -2)
x 2 − 1 0 x + 2 1 = 0 yields solutions 7 and 3
Thus, − 1 + − 4 + − 3 + 7 + 3 = 2
i cant understand second point
Log in to reply
for second solution to be valid, x 2 − 1 0 x + 2 1 must be an even number because ( − 1 ) e v e n n u m b e r = 1 but ( − 1 ) o d d n u m b e r = − 1 . That is why only x = − 3 is a valid solution in the second case.
why not (-2)?
Log in to reply
-2 makes the power odd which results in -1 not 1
why not -2?
I forgot the -3
There are three scenarios which solve the problem:
- The exponent is 0, as anything (except 0 itself) raised to 0 is 1
- The base is 1, as positive 1 raised to any value is always 1
- The base is -1 and the exponent is even
Case 1:
x 2 − 1 0 x + 2 1 = 0
( x − 7 ) ( x − 3 ) = 0
x = 7 , x = 3
The base is non-zero for both of these roots, so these are two solutions to the problem.
Case 2:
x 2 + 5 x + 5 = 1
x 2 + 5 x + 4 = 0
( x + 4 ) ( x + 1 ) = 0
x = − 4 , x = − 1
Case 3:
x 2 + 5 x + 5 = − 1
x 2 + 5 x + 6 = 0
( x + 3 ) ( x + 2 ) = 0
x = − 3 , x = − 2
We need to test these two roots in the exponent. Exponent must be even.
( − 3 ) 2 − 1 0 ( − 3 ) + 2 1 = 9 + 3 0 + 2 1 = 6 0
This one works!
( − 2 ) 2 − 1 0 ( − 2 ) + 2 1 = 4 + 2 0 + 2 1 = 4 5
This does not work.
So our only valid solution in Case 3 is x = − 3
Result
− 4 − 1 + 7 + 3 − 3 = 2
I like this solution the most because it 'solves' each scenario rather than just 'concluding' the answer.
we have three choices choice one: x2+5x+5 =1 choice two: x2−10x+21=0 choice three: x2+5x+5=-1 and x2−10x+21 is even the first one have two solutions: 7 , 3 the second one have two solutions: -1,-4 the third one have one solution: -3 7+3 + -1 + -4 + -3 = 2 the solution is 2
x 2 + 5 x + 5 can take values 1 and -1(if x 2 − 1 0 x + 2 1 is even). x 2 + 5 x + 5 is 1 at -4 and -1 and -1 at -3 and -2 ,but x 2 − 1 0 x + 2 1 is not even at -2 so the solution from here are -4,-3,-1.
x 2 − 1 0 x + 2 1 can be zero ⇒ at 7 and 3
sum = 7 + 3 − 4 − 3 − 1 = 2
(x^{2}+5 \times x+5)^{x^{2}−10 \times x+21}=1
There 2 cases in which the equation would be zero. That is either, the power {x^{2}−10 \times x+21} is zero or when the base number (x^{2}+5 \times x+5) is one or negative one.
When x^{2}−10 \times x+21= 0, x= 7,3 When x^{2}+5 \times x+5= 1, x= -4, -1 When x^{2}+5 \times x+5= -1, x= -3, -2
However, in the last case above ( when the base is equal to negative one) -2 is invalid. This is due to the fact that when -2 is substituted into the power, the power would be an odd number. If the power was a odd number, the whole equation would be -1^{odd}= -1.
This leaves 5 solutions. They are: 7, 3, -4, -1 and -3. 7+3-4-1-3=2
enclose maths in \ ( and \ )
see jordi bosch's answer.
I had to plug in the equation into wolfram to see what the answer was and realized I was missing 1^any number=1 and (-1)^even number=1 solutions.
Put the power as 0 because the answer on the is one.. so, 2 is the answer..
Missed the third case in first attempt but got it in the second
This is the second problem of the same type I think with in 9 months! This time I did it correctly, using -1, and solving for 0, +1, -1.
I solved the problem the standard way by taking natural logs of both sides. Eventually this comes out to: ( x − 3 ) ( x − 7 ) ln ( x 2 + 5 x + 5 ) = 0 . We want part of this to be zero. Either we can accomplish that by either making ( x − 3 ) or ( x − 7 ) zero, or we could make the natural log equal to zero, by making the argument of the logarithm equal to 1. That becomes another quadratic equation for the latter, and you can obtain 1 as the argument with x = − 1 or x = − 4 . That gives 3 , 7 , − 1 , − 4 . Also, if we make the . However, we're not done yet. What if ( x 2 + 5 x + 5 ) gives -1, and x 2 − 1 0 x + 2 1 ) is even? Then, we can still satisfy the equation. Set ( x 2 + 5 x + 5 ) equal to 1 and factor it. Here, you can get either − 2 or − 3 . Only − 3 works because it makes x 2 − 1 0 x + 2 1 even, and − 1 raised to an even exponent results in 1 . − 2 makes x 2 − 1 0 x + 2 1 odd. Finally, the sum of all solutions 3 + 7 − 1 − 4 − 3 = 2 .
First make x^2-10x+21=0.then find solutions of this which are 7 and 3 Next make x^2+5x+5=1 find solutions of this which are -1 and -4 At last make x^2+5x+5=-1 find solutions which are -2 and -3. -2 doesn't satisfy this condition. Add 7, 3,-1,-4 and -3 you will get the answer I.e. 2

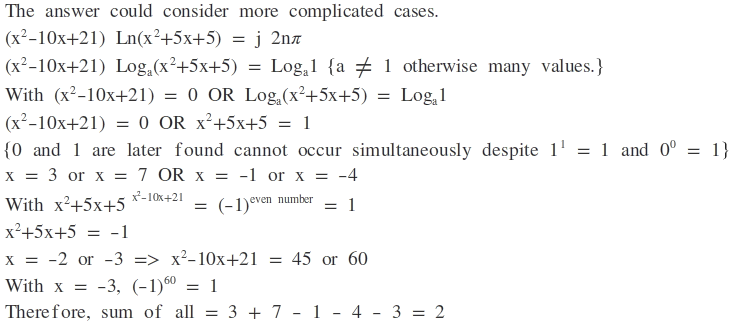
We must consider three cases:
The exponent equals to 0 .
Solving x 2 − 1 0 x + 2 1 we get as a solutions 7 and 3 .
The base is 1 .
Solving x 2 + 5 x + 4 we get as a solutions − 1 and − 4
The base is − 1 and the exponent is even.
Solving x 2 + 5 x + 6 we get as a solutions − 3 and − 2
We put the values on the exponent equation and only − 3 gives an even exponent.
So the sum of all solutions is: 7 + 3 − 1 − 4 − 3 = 2