Pull the Pulley

Classical Mechanics Level 4

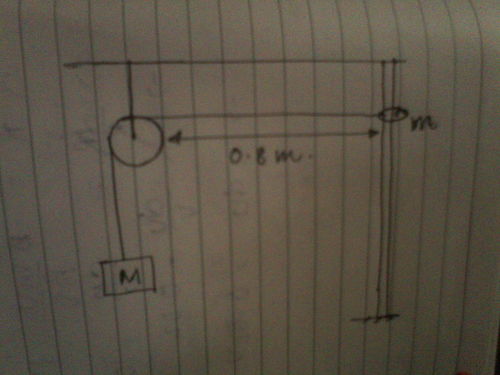A ring of mass m=0.3kg can slide freely on smooth vertical rod. A light and inextensible string is connected to the ring and passes over a smooth and fixed pulley as shown in the figure. The distance of the pulley from the rod is 0.8m and the other end of the string is connected to a block of mass M=0.5kg . The ring is held in level with pulley and then released from rest. Determine the distance (in meter) by which the ring moves down before coming to rest for the first time.

The answer is 1.5.

3 solutions

Niranjan Khanderia
May 5, 2014

P.E. lost by .3kg moving down by H meter = P.E. gain by .5kg. ... $P.E. =m* g* h$
From the sketch, .5kg is moved up by the diagonal string minus initial distance .8. $.3 * g* H = .5 * g* { sqrt(H^2 + .8^2) - .8}$ .......Gives $H = 1.5m$

but why would the 0.5 kg move up ... i mean the block is heavier than the ring ... so cant the ring go up and the block move down ?

Rishikesh Patil - 7 years, 1 month ago

Bcoz the rod is fixed..so it can't come forward..so the ring will go down and. Mass will come up...nd u can see it in that way too..that's it's not just normal tension..it's the component of tension..so u can't say..that which is heavier..will go down

vandana kumari - 2 years, 9 months ago

I support #Rishikesh Patil. How will the ring move down when it is lighter than mass at other end?

Anandhu Raj - 6 years, 4 months ago

There is no vertical force acting on the ring other than gravity ,initially so it will move down. @Anandhu Raj

satvik pandey - 6 years, 4 months ago

Don't we have to take care of tension on string? @satvik pandey

Anandhu Raj - 6 years, 4 months ago

@Anandhu Raj – Initially tension acts in the horizontal direction. @Anandhu Raj

satvik pandey - 6 years, 4 months ago

what I had in mind was that the ring will get locked at the resting position as mass M > m
so technically the tension in the string would be T=(Mg-ma) since m is at rest so T=Mg and this is the force which is pulling the ring towards the pulley if friction free system is assumed so i dont see how this small ring mass moves down

Junaid Chaudhry - 6 years, 4 months ago

Kaustubh Deshpande
Apr 30, 2014

0.3 g l=0.5*((0.8^2+l^2)-0.8) solve for l you will get l=1.5

Can u explain elaborately ?

Rishikesh Patil - 7 years, 1 month ago

The explanation is given above.

Niranjan Khanderia - 7 years, 1 month ago

Vandana Kumari
Aug 18, 2018

These kind of sums has a general formula too rather than solving by regular physics..the formula is 2mMa/M^2-m^2

Pull the Pulley

The answer is 1.5.

3 solutions

0 pending reports