A good geometry problem
In the diagram below, angle is a right angle. Point is on , and bisects angle . Points and are on and , respectively, so that and . Given that and , find the integer closest to the area of quadrilateral .
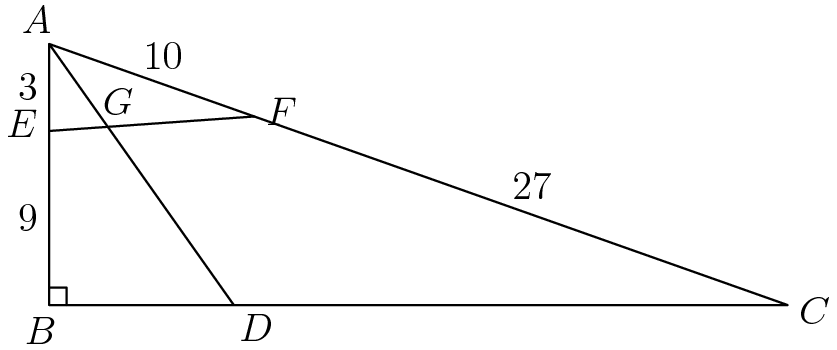
The answer is 148.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
By the Pythagorean Theorem, B C = 3 5 . Letting B D = x we can use the angle bisector theorem on triangle A B C to get x / 1 2 = ( 3 5 − x ) / 3 7 , and solving gives B D = 6 0 / 7 and D C = 1 8 5 / 7 .
The area of triangle A G F is 1 0 / 3 that of triangle A E G , since they share a common side and angle, so the area of triangle A G F is 1 0 / 1 3 the area of triangle A E F .
Since the area of a triangle is 2 a b sin C , the area of A E F is 5 2 5 / 3 7 and the area of A G F is 5 2 5 0 / 4 8 1 .
The area of triangle A B D is 3 6 0 / 7 , and the area of the entire triangle A B C is 2 1 0 . Subtracting the areas of A B D and A G F from 2 1 0 and finding the closest integer gives 1 4 8 as the answer.