Can We Can Calculate Its Diagonal?

Geometry Level 2

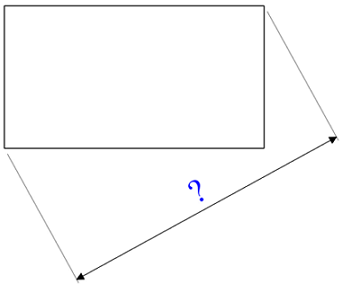
A rectangle has perimeter and area, $32\text{ m}$ and $56\text{ m}^2$ respectively. Find the length of its diagonal?

12\text{ m}

14\text{ m}

15\text{ m}

16\text{ m}

2 solutions

Sonveer Yadav
Mar 16, 2016

Let $'l'$ and $'b'$ be the length and breadth of the rectangle.
perimeter = $2\times (l+b)$ = 32.
$\Rightarrow l+b = 16 \rightarrow (1)$ Also, we have area = $l\times b = 56$ . squaring both the sides of eq (1) we get, ${(l+b)}^2 = 256$ .
$l^2 + b^2 + 2ab = 256$ . $\Rightarrow l^2 + b^2 + 2\times 56 = 256$ .
$\Rightarrow l^2 + b^2 = 256 - 112 = 144$ . $\sqrt {l^2+b^2} = 12$ = diagonal of the rectangle. ( By Pythagorean theorem )

did the exact same thing

Karish Thangarajah - 5 years, 2 months ago

Great Solution!!!

Abdur Rehman Zahid - 5 years, 2 months ago

In Response to Abdur Rehman Zahid. Thank You!!

Jim Addams - 5 years, 2 months ago

So what are the values of l and b such that 2l + 2b = 32 while l*b = 56, went through the factors of 56 and none them work.

Ethan Pepper - 5 years, 2 months ago

We can get the ans by solving the following quadratics eq. X^2-16x+56=0 Here 16= half of the perimeter and 56= area .answer= lenght=10.8285 and breath =5.1715 .

Yash Jagani - 5 years, 2 months ago

Yes, they use the factors (8 + sqrt8) and (8- sqrt8) difference of squares 64-8 = 56. Now it makes sense.

Ethan Pepper - 5 years, 2 months ago

In response to Yash Jagani. I see that solving this quadratic gives the solution, but I can't work it backwards. Could you please show me how you got this equation from the given information? ie how do you know the 16 and 56 go here? Thanks!

Jim Addams - 5 years, 2 months ago

@Jim Addams – It is given that: $\begin{cases} 2l+2b=32\implies l+b=16\\ lb=56\end{cases}$ Isolate one of the variables from any one of the equations: Let's isolate $l$ from the first equation. We get: $l=16-b$ Substitute the value of $l$ from the first equation into the second equation. We get: $\begin{aligned} lb &=56\\ (16-b)b &=56\\ 16b-b^2&=56\\ -b^2+16b-56&=0\\ (-1)\times (-b^2+16b-56)&=(-1)\times 0\\ b^2-16b+56&=0 \end{aligned}$

Abdur Rehman Zahid - 5 years, 2 months ago

Pawan Pal
Mar 16, 2016

LET a be the length and b be the breadth. perimeter is 32 i.e 2(a+b)=32 area is 56 i.e a b=56 now we know a+b is 16 square both sides a^2 + b^+ 2ab =256 a^2 +b^2 = 256-2 56=144 therfore length of diagonal is square root of a^2 +b^2 i.e 12..

Can We Can Calculate Its Diagonal?

2 solutions

0 pending reports