Related to Open Problem #1: Knights and Kings unlimited!

Logic Level 4

On an infinitely large chess board, is there a configuration of kings and knights such that each king attacks exactly 2 kings and 2 knights, and each knight attacks exactly 2 kings and 2 knights?

This problem is part of the new Brilliant.org Open Problems Group (see Open Problem #1 ).

Yes No

Novak Radivojević by Novak Radivojević , 23, Serbia

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

6 solutions

Jon Haussmann
Nov 13, 2017

Assume the pattern continues indefinitely.

25 Helpful 0 Interesting 0 Brilliant 0 Confused

A white knight DOES not attack another white night, and a king CANNOT attack another king of any color.

Scot Magann - 3 years, 6 months ago

Log in to reply

You need not think lie a chessman; Think in terms of MATHS THE LANG. IS NOT MADE CLEAR ALTHOUGH

Ariijit Dey - 3 years, 6 months ago

see we don't need any pattern for the answer you r not asked to map the pieces to each other; you can think interns of distinct blocks SINCE THE BOARD IS INFINITE WE HAVE ENOUGH ROOM TO PLACE ANY OF THE PIECES WE WANT; KEEPING SUFFICIENT GAP IN BETWEEN IN ORDER TO AVOID MULTIPLE ATTACKS;;;

Ariijit Dey - 3 years, 6 months ago

TRY THIS https://brilliant.org/problems/pental-bogda/?ref_id=1433431

Ariijit Dey - 3 years, 6 months ago

https://brilliant.org/problems/pental-bogda/?ref_id=1433431

Ariijit Dey - 3 years, 6 months ago

There exists a pattern which can be used to fill the infinite grid as follows:

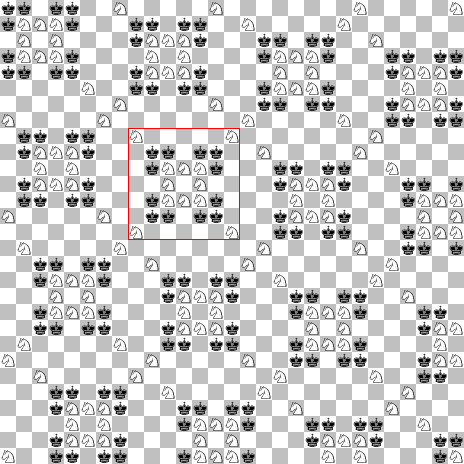
Can you find more of them?

14 Helpful 0 Interesting 0 Brilliant 0 Confused

Hey, I can but I can't because I wanna make an image. How did you made it? Thanks!

A Former Brilliant Member - 3 years, 7 months ago

Log in to reply

You can do it with a chess problem diagrams package for LaTeX, but honestly, I was too lazy to use it so I just copied a picture from the internet, and edited it using Paint. xD

Novak Radivojević - 3 years, 7 months ago

Log in to reply

Thats amazing! I didn't knew bout that package! Thanks.

A Former Brilliant Member - 3 years, 7 months ago

Log in to reply

@A Former Brilliant Member – No problem :)

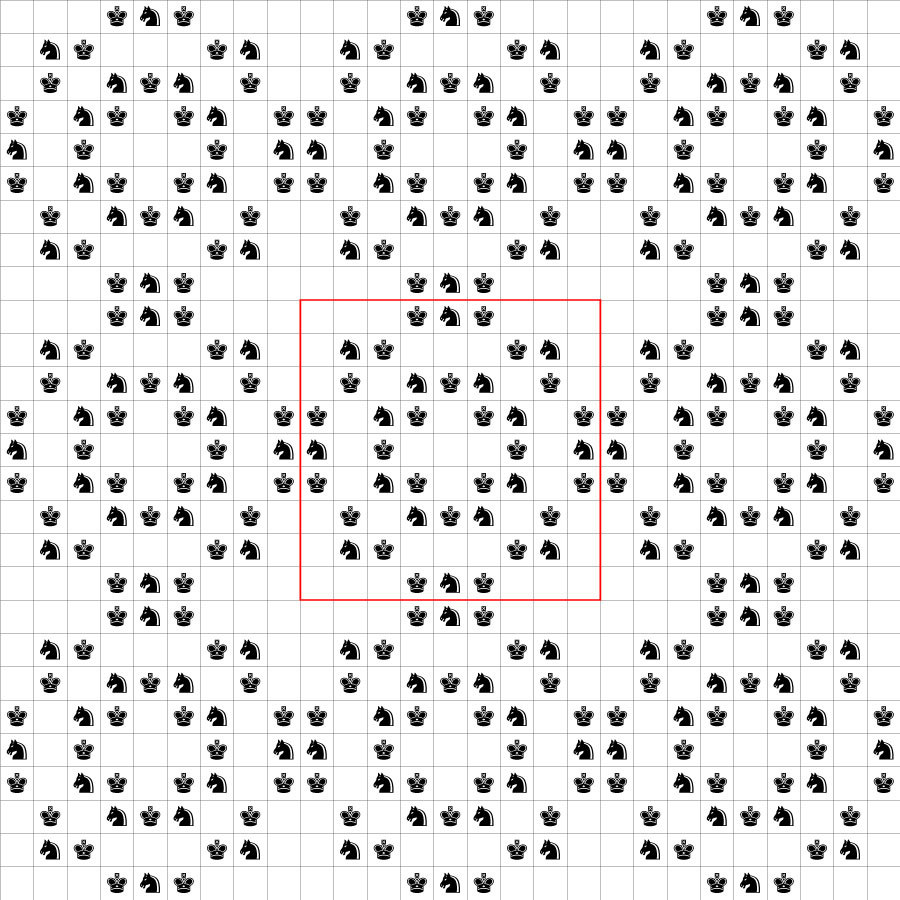
Novak Radivojević - 3 years, 7 months ago

Yep, here's another one: Infinite Grid Assume that the red squares are occupied by knights and the blue squares are occupied by kings.

Andrew Wang - 3 years, 6 months ago

A white knight DOES not attack another white night, and a king CANNOT attack another king of any color.

Scot Magann - 3 years, 6 months ago

see we don't need any pattern for the answer you r not asked to map the pieces to each other; you can think interns of distinct blocks SINCE THE BOARD IS INFINITE WE HAVE ENOUGH ROOM TO PLACE ANY OF THE PIECES WE WANT; KEEPING SUFFICIENT GAP IN BETWEEN IN ORDER TO AVOID MULTIPLE ATTACKS;;;

Ariijit Dey - 3 years, 6 months ago

CHECK THIS: https://brilliant.org/problems/pental-bogda/?ref_id=1433431

Ariijit Dey - 3 years, 6 months ago
Sizheng Chen
Nov 20, 2017

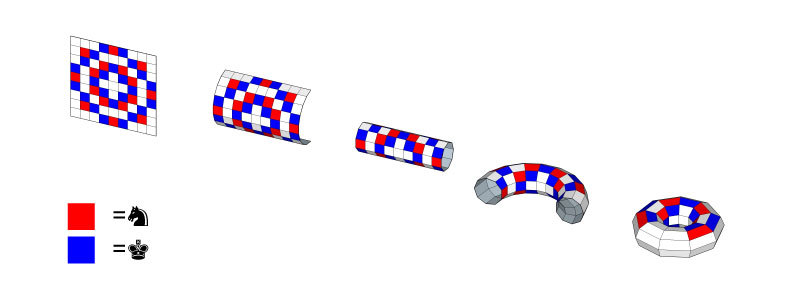
Here's another infinitely tiled pattern, with uneven numbers of kings and knights (3 kings for every 2 knights): Additionally, if the pattern is mapped onto a torus, it would work on its own:

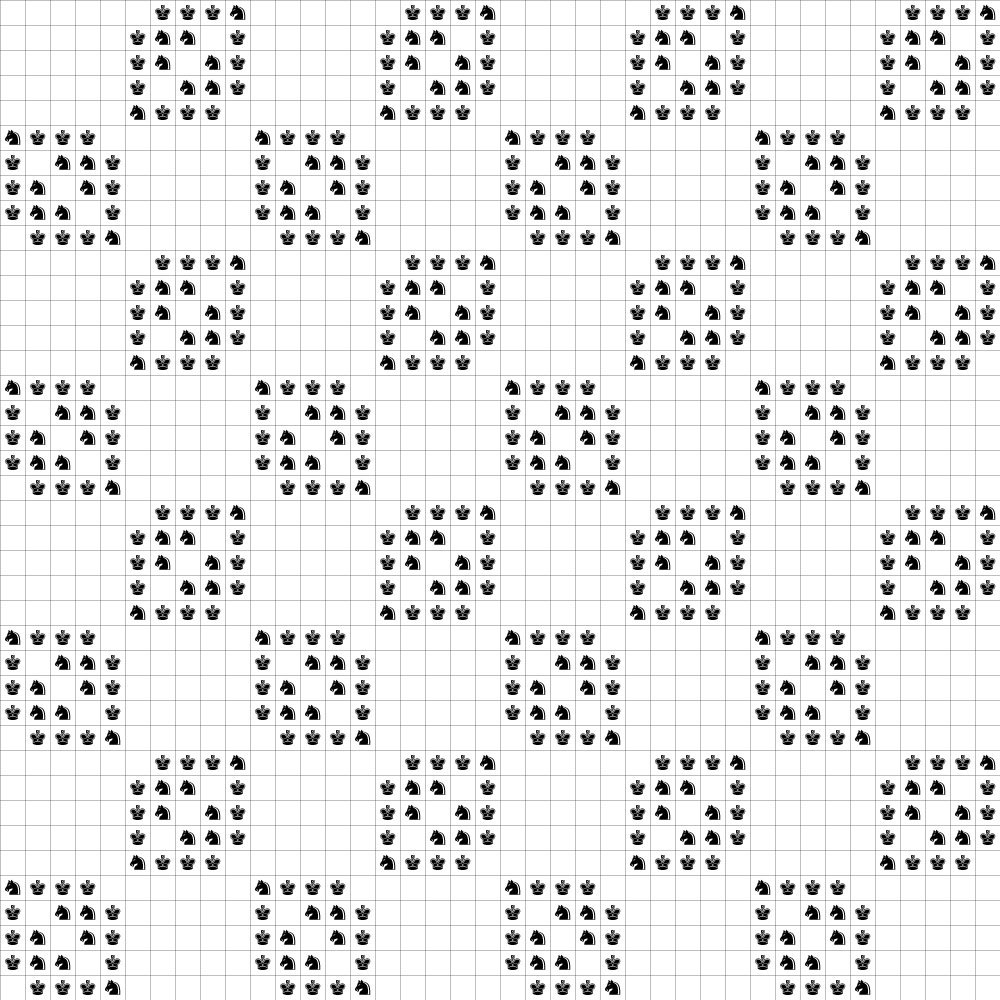
While working out the corner problem here , I also found this infinitely tiled pattern (interestingly, also 3 kings for every 2 knights):

13 Helpful 0 Interesting 0 Brilliant 0 Confused

see we don't need any pattern for the answer you r not asked to map the pieces to each other; you can think interns of distinct blocks SINCE THE BOARD IS INFINITE WE HAVE ENOUGH ROOM TO PLACE ANY OF THE PIECES WE WANT; KEEPING SUFFICIENT GAP IN BETWEEN IN ORDER TO AVOID MULTIPLE ATTACKS;;;

Ariijit Dey - 3 years, 6 months ago

https://brilliant.org/problems/pental-bogda/?ref_id=1433431

. CHECK MY PROBLEM

Ariijit Dey - 3 years, 6 months ago

2 Helpful 0 Interesting 0 Brilliant 0 Confused
Mark Hennings
Nov 20, 2017

2 Helpful 0 Interesting 0 Brilliant 0 Confused
Jeremy Galvagni
Nov 25, 2017

I'm not picture savvy but this is easy to describe:

Of three rows:

Make the middle an infinite row of Kings with knights in every third position in the row above and every third position in the row below, offset by 1.

(The Kings are fulfilled, but the knights only attack one knight at this point.)

Next row all blanks.

Next three rows same as first three, again offset by 1.

(Makes the knights attack one knight across the blank row.

0 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...