Roots Dilemma

Since $x^3-x+1 = 0\quad \Rightarrow \color{#3D99F6}{1} = x - x^3 = x(1-x^2) = \color{#3D99F6}{x(1-x)(1+x)}$ . Therefore,

$\begin{aligned} \frac{\color{#3D99F6}{1}}{a+1} + \frac{\color{#3D99F6}{1}}{b+1} + \frac{\color{#3D99F6}{1}}{c+1} & = \frac{\color{#3D99F6}{a(1-a)(1+a)}}{a+1} + \frac{\color{#3D99F6}{b(1-b)(1+b)}}{b+1} + \frac{\color{#3D99F6}{c(1-c)(1+c)}}{c+1} \\ & = a(1-a)+b(1-b)+c(1-c) \\ & = \color{#3D99F6}{a+b+c} - (\color{#D61F06}{a^2+b^2+c^2}) \quad \quad \small \color{#3D99F6}{\text{See Note.}} \\ & = \color{#3D99F6}{0} - (\color{#D61F06}{2}) = \boxed{-2} \end{aligned}$

Note: Using Vieta's formulas, we have:

$\color{#3D99F6}{a+b+c = 0}$

$\color{#D61F06}{a^2+b^2+c^2 = (a+b+c)^2 -2(ab+bc+ca) = 0^2 -2(-1) = 2}$

Observe that: $x^3-x+1= (x-a)(x-b)(x-c) = x^3 -(a+b+c)x^2+(ab+bc+ca)x-abc$ .Therefore $a+b+c = 0, ab+bc+ca= -1$ ... (1)

And: When $x=-1, 1 = (-1)^3 -(-1)+1 = (-1-a)(-1-b)(-1-c)= -(1+a)(1+b)(1+c)$ ... (2)

$\dfrac{1}{a+1}+\dfrac{1}{b+1}+\dfrac{1}{c+1} = \dfrac{ab+bc+ca+2(a+b+c)+3}{(1+a)(1+b)(1+c)}$

By substituting (1) and (2), $=\dfrac{-1+2(0)+3}{-1}$ $=-2$

if a,b,c are roots, then $f(x)=x^3-x+1=(x-a)(x-b)(x-c)$ d.w.r.x $f'(x)=3x^2-1=(x-a)(x-b)+(x-b)(x-c)+(x-c)(x-a)$ divide: $\dfrac{f'(x)}{f(x)}=\dfrac{3x^2-1}{x^3-x+1}=\dfrac{(x-a)(x-b)+(x-b)(x-c)+(x-c)(x-a)}{(x-a)(x-b)(x-c)}=\dfrac{1}{x-a}+\dfrac{1}{x-b}+\dfrac{1}{x-c}$ x = -1: $2=-(\dfrac{1}{1+a}+\dfrac{1}{1+b}+\dfrac{1}{1+c})$ hence answer is $\boxed{-2}$

Let $y=x+1.$

$(y-1)^3-(y-1)+1=0$

$y^3-1-3y(y-1)-y+2=0$

$y^3-3y^2+2y+1=0$

Let z= $\frac{1}{y}$

$y=\frac{1}{z}$

$\frac{1}{z^3}-\frac{3}{z^2}+\frac{2}{z}+1=0$

$z^3+2z^2-3z+1=0$

Roots of this equation are $\frac{1}{a+1}$ , $\frac{1}{b+1}$ , $\frac{1}{c+1}$

Sum of roots =-2.

Expanding numerator and denominator by simply taking LCM and multiplying we get [2(a+b+c) + (ab+ bc +ca)+3]

divided by

[(abc)+(ab+bc+ca)+(a+b+c)+1]

.....(1)

Now from the cubic equation of general form

Px^3+Qx^2+Rx+S

having roots a,b,c we know Sum of roots =a+b+c= -b/a

Sum of product of root taken two at a time =ab+ bc +ca =c/a

Product of roots = abc = -d/a

Comparing this with x^3-x+1 we get a+b+c = 0
ab+ bc +ca=-1
abc=-1

Now substituting in (1) we get ans as -2

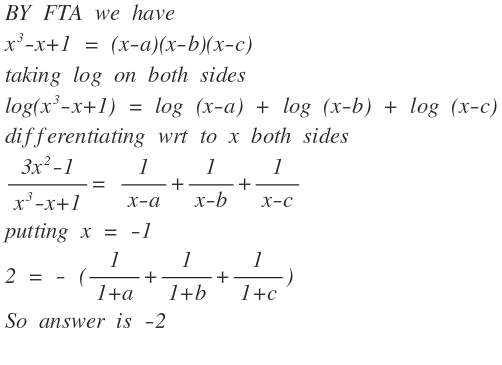
FTA means Fundamental Theorem of Algebra :- https://brilliant.org/wiki/fundamental-theorem-of-algebra/