Serious Series
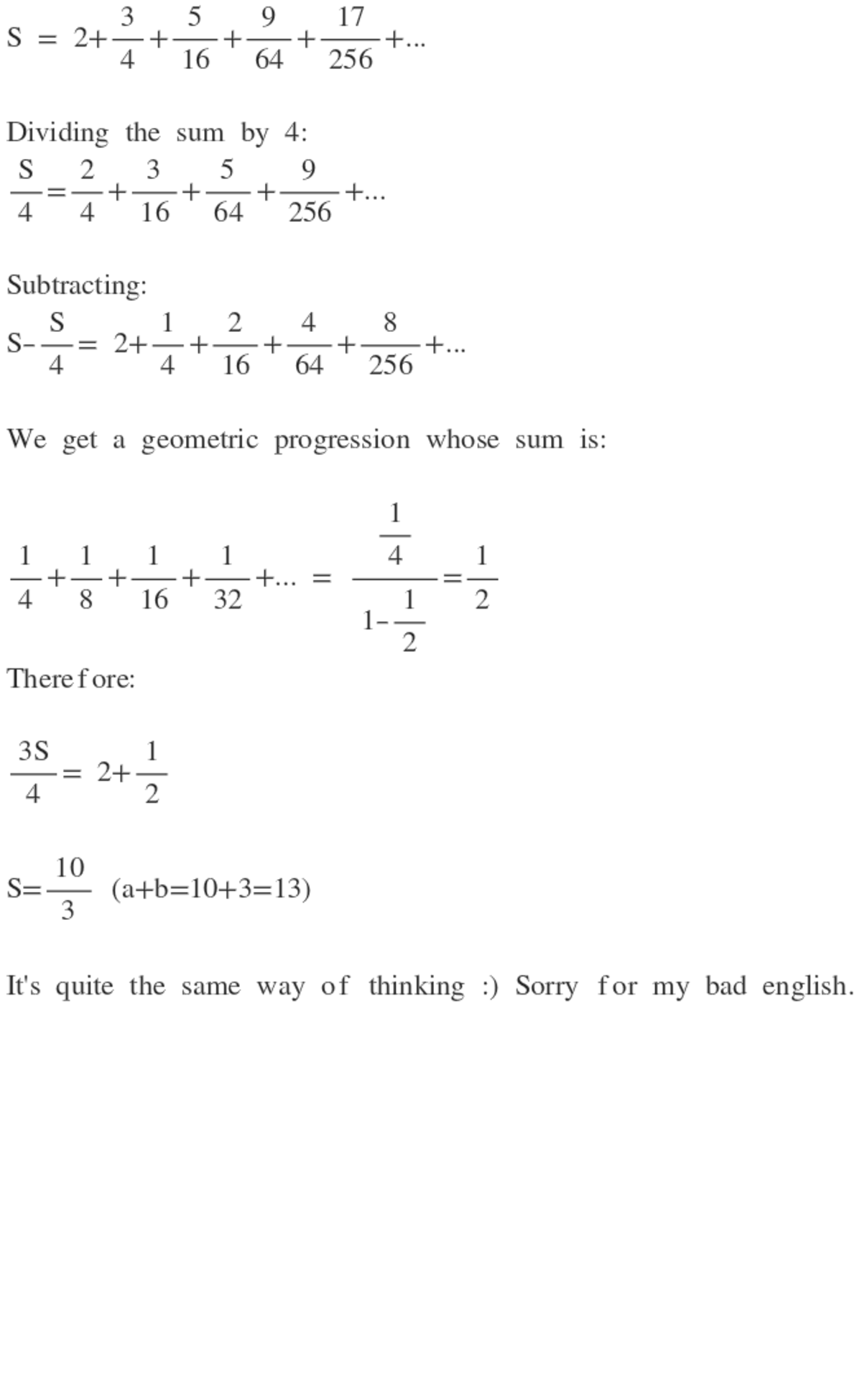
Let the sum of all terms of the infinite series 2 , 4 3 , 1 6 5 , 6 4 9 , 2 5 6 1 7 , ⋯ , be x .
Let x have a rational representation as x = b a , where a and b are co-prime.
What is the value of a + b .
The answer is 13.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
That is a beautiful approach. I just loved it.
Log in to reply
Thank you @Soumava Pal
Log in to reply
You are welcome. I did it by @Janardhanan Sivaramakrishnan 's method only, but yours seemed a lot elegant.
Log in to reply
@Soumava Pal – Also we can do it by dividing by 4.. and subtracting ..But both come same after 2 or 3 steps.
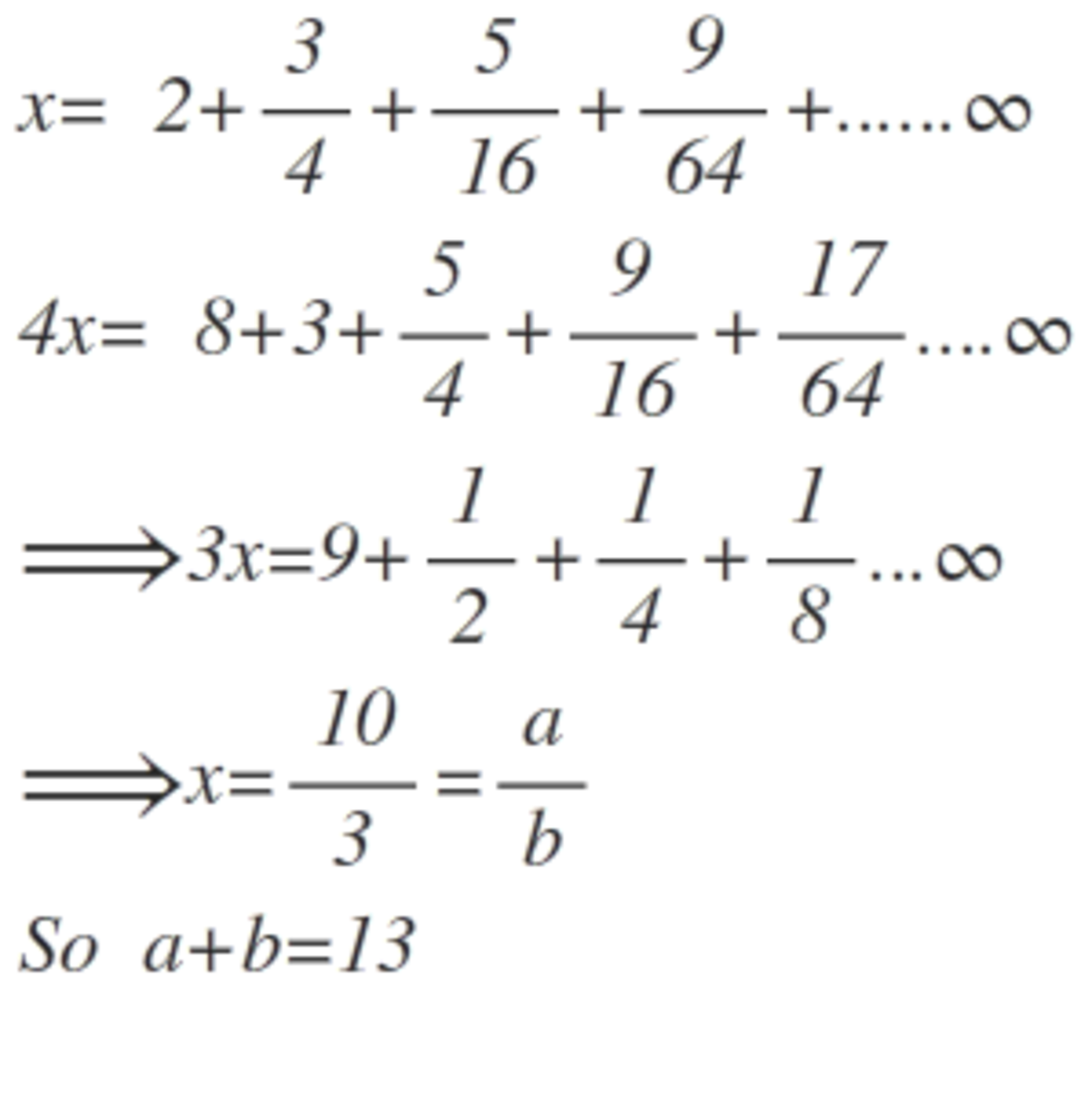
The series can be written as
1 + 1 , 4 2 + 1 , 4 2 2 2 + 1 , 4 3 2 3 + 1 , ⋯
which can be rewritten as
1 + 1 , 2 1 + 4 1 , ( 2 1 ) 2 + ( 4 1 ) 2 , ( 2 1 ) 3 + ( 4 1 ) 3 , ⋯
The series is the sum of terms of two convergent GPs both starting at 1 and with ratios 2 1 and 4 1 .
Thus, the required sum would be
S = 1 − 2 1 1 + 1 − 4 1 1 = 2 + 3 4 = 3 1 0
Thus, a = 1 0 , b = 3 , a + b = 1 3