Slippery Pulley!

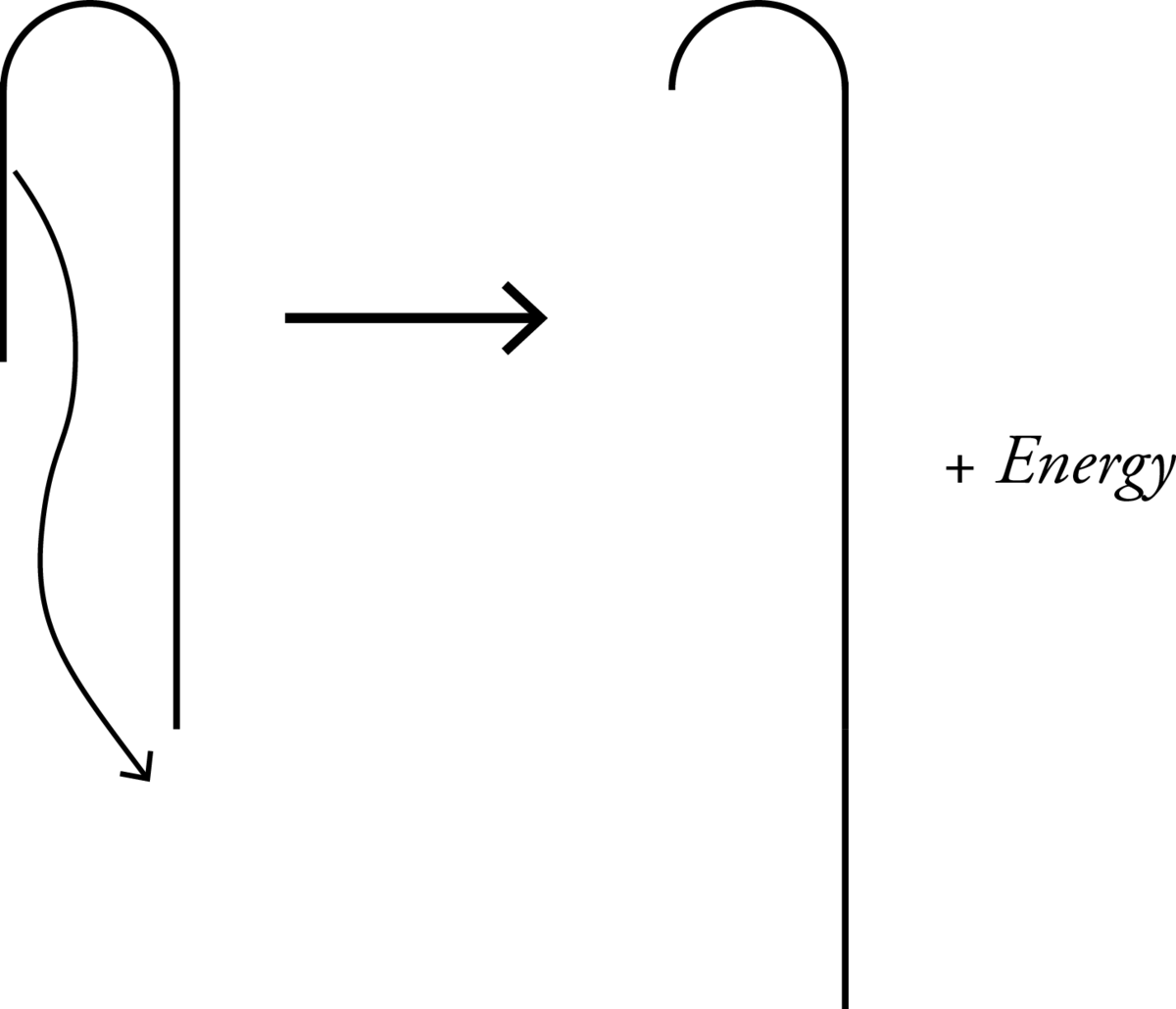
From the question All that matters is the constant velocity! , we know that once the chain is out of the pulley, $T = \rho v^2\cdots(1)$

Now when one side of the chain goes a height $x$ , $\bigtriangleup\! PE = \;\bigtriangleup\! KE\\\rho x^2g = \frac{1}{2}\rho L v^2\\\Rightarrow Lv^2 = 2x^2g\cdots(2)$

Now equating forces

$T -\rho \left(\dfrac{L}{2} - x\right)g = \rho \left(\dfrac{L}{2} - x\right) a\cdots(3)\\ \rho \left(\dfrac{L}{2} + x\right)g - T = \rho \left(\dfrac{L}{2}+ x\right)a\cdots(4)$

Solving $Lv^2 = \dfrac{L^2}{2}g - 2x^2g\cdots(5)$

Substituting $(1), (2)$ in $(5)$ , $h_{break} = \dfrac{L}{2\sqrt{2}}$

$v_{break} = \dfrac{\sqrt{Lg}}{2}$

$\Rightarrow v = w = 0.5,~x = 2,~y = 1,~z=2\sqrt{2}$

$\large\color{#3D99F6}{\therefore \boxed{\dfrac{v+w}{2} + x + y + z \approx 6.32843}}$