Sprinkler Power

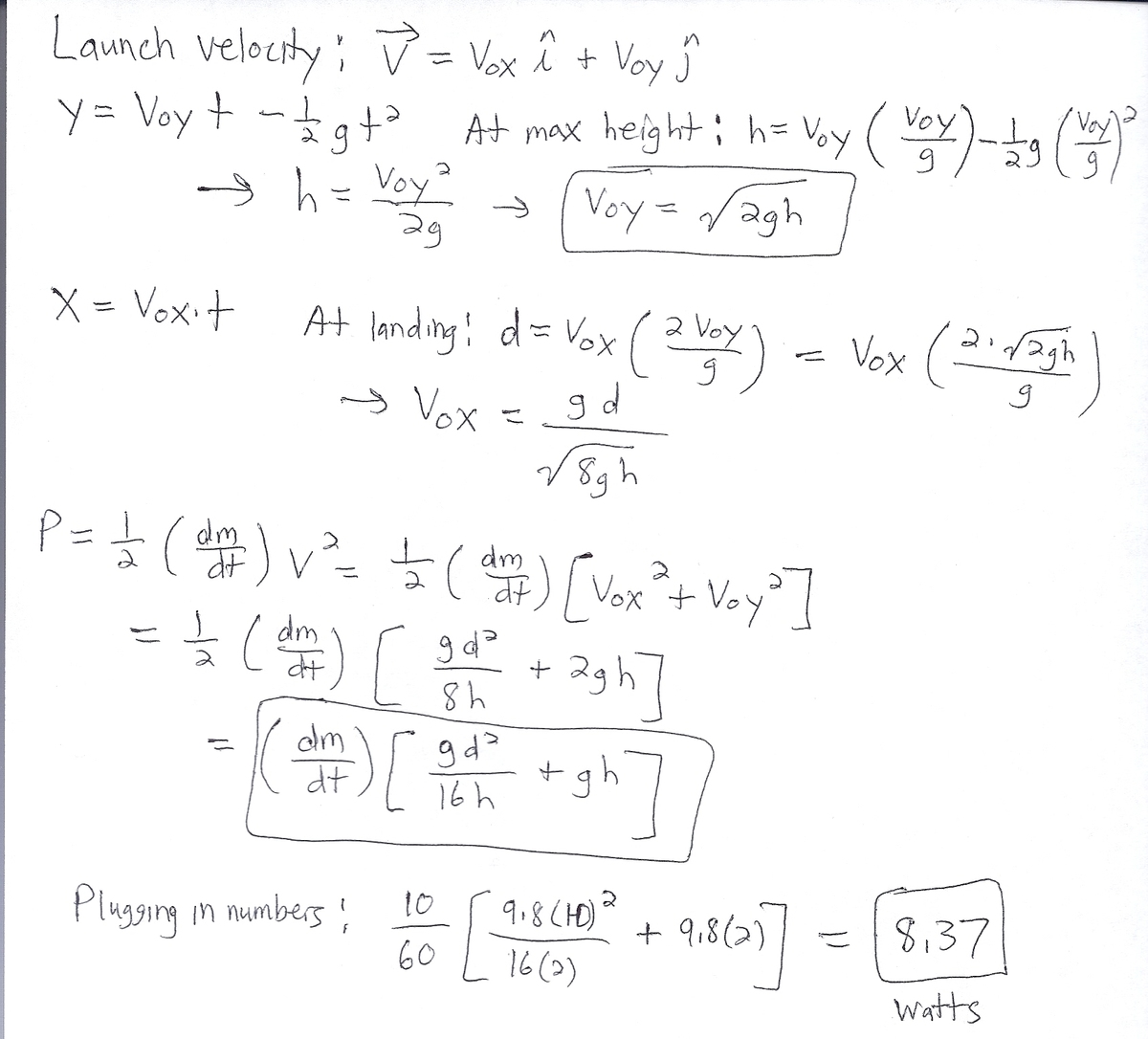
First, we have to calculate the energy that is being given to the water as it leaves the sprinkler. For any individual part of the stream, we know that it will travel to a maximum height of $2$ metres, and will travel a horizontal distance of $10$ metres. We know that:

$E_s \ = \ \frac{1}{2} mv^2 \ = \ \frac{1}{2} m(v_x^{2} \ + \ v_y^{2})$

In order to find the kinetic energy that arises from the velocity in the $y$ -direction, we simply remember that all of this kinetic energy will be converted to potential energy at the maximum height of the arc, thus:

$\frac{1}{2}mv_{y}^2 \ = \ mgh \ \Rightarrow \ v_y \ = \ \sqrt{2gh}$

We also know that:

$x \ = \ v_x t \ \ \ \ \text{and} \ \ \ y \ = \ v_y t \ - \ \frac{1}{2} gt^2$

The time at which the water has travelled the distance of $10$ metres will be when $y \ = \ 0$ , thus:

$v_y t \ - \ \frac{1}{2} gt^2 \ = \ 0 \ \Rightarrow \ v_y t \ = \ \frac{1}{2} gt^2 \ \Rightarrow \ t \ = \ 0, \ \frac{2 v_y}{g}$

We are interested in the second solution, as this allows us to find the velocity in the $x$ direction:

$x \ = \ v_x t \ \Rightarrow \ v_x \ \frac{2 v_y}{g} \ = \ R \ \Rightarrow \ v_x \ = \ \frac{Rg}{2 v_y}$

$E_s \ = \ \frac{1}{2} m(v_x^{2} \ + \ v_y^{2}) \ = \ mgh \ + \ \frac{1}{2} \ m \ \Big( \frac{Rg}{2 v_y} \Big)^2 \ = \ mg \Big( h \ + \ \frac{R^2}{8h} \Big)$

And recalling the definition of power, we have:

$P_s \ = \ \frac{\text{d}E}{\text{dt}} \ = \ \frac{\text{d}}{\text{dt}} \ mg \Big( h \ + \ \frac{R^2}{8h} \Big) \ = \ g \Big( h \ + \ \frac{R^2}{16h} \Big) \ \frac{\text{d}m}{\text{dt}} \ = \ (9.8) \ \Big( 2 \ + \ \frac{100}{32} \Big) \ \frac{1}{6} \ \approx \ 8.37 \ \text{W}$